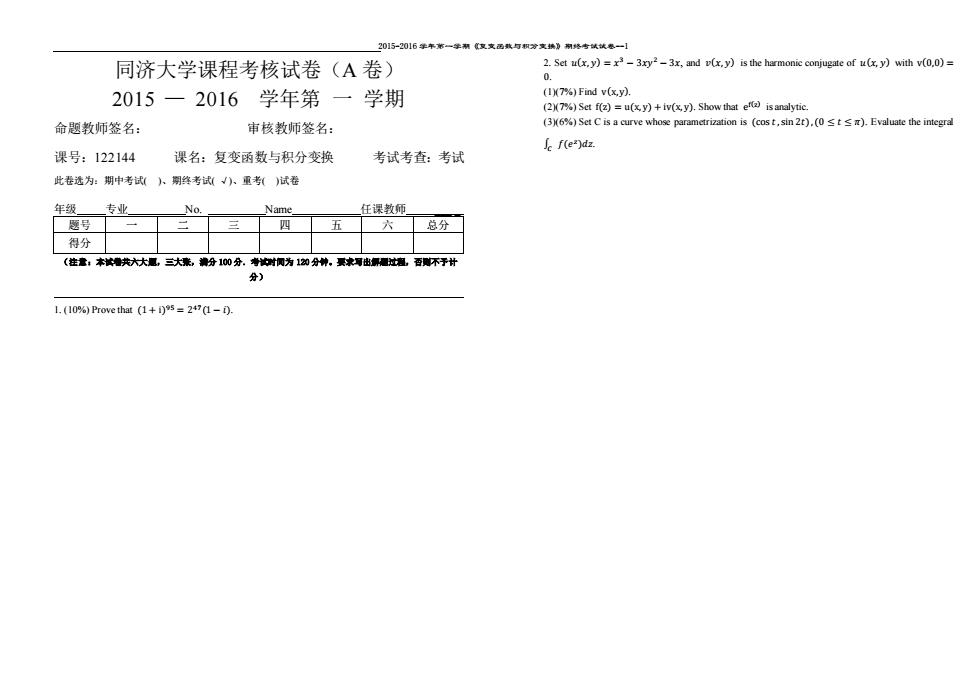
2015-2016学年常 同济大学课程考核试卷(A卷) 2.Set u(x.y)=x-3xy2-3x,and v(x,y)is the harmonic conjugate of u(x,y)with v(0.0)= 2015-2016 学年第一 学期 (I(7%)Find v(xy). (27%)Set f(z)=u(xy)+iv(xy).Show that e(is analytic. 命题教师签名: 审核教师签名: (3(6%)Set C is a curve whose parametrization is (cost,sin 2t).(0t).Evaluate the integral lf(e)dz. 课号:122144 课名:复变函数与积分变换 考试考查:考试 此卷选为:期中考试(、期终考试(√)、重考)试卷 年级 专业 No. Name 任课教师 题号 总分 得分 (往意:本试增共大大题,三大张,潮分100分.考汝树阅为12动分钟,要来可出圆过超,否不于计 分) L(I0%)Prove that(1+)5=2470-)
2015-2016 学年第一学期《复变函数与积分变换》期终考试试卷--1 同济大学课程考核试卷(A 卷) 2015 — 2016 学年第 一 学期 命题教师签名: 审核教师签名: 课号:122144 课名:复变函数与积分变换 考试考查:考试 此卷选为:期中考试( )、期终考试( √)、重考( )试卷 年级 专业 No. Name 任课教师 ___ _ 题号 一 二 三 四 五 六 总分 得分 (注意:本试卷共六大题,三大张,满分 100 分.考试时间为 120 分钟。要求写出解题过程,否则不予计 分) 1. (10%) Prove that (1 + i) 95 = 2 47(1 − 𝑖). 2. Set 𝑢(𝑥, 𝑦) = 𝑥 3 − 3𝑥𝑦2 − 3𝑥, and 𝑣(𝑥, 𝑦) is the harmonic conjugate of 𝑢(𝑥, 𝑦) with v(0,0) = 0. (1)(7%) Find v(x,y). (2)(7%) Set f(z) = u(x, y) + iv(x, y). Show that e f(z) is analytic. (3)(6%) Set C is a curve whose parametrization is (cos 𝑡, sin 2𝑡) , (0 ≤ 𝑡 ≤ 𝜋). Evaluate the integral ∫ 𝑓(𝑒 𝑧)𝑑𝑧 𝐶

2015-2016华年一学文西我与积分支换南以一2 3.Set /(a) 4.(1)(%)Evaluate the integral by using residue theorem sin6 de (1)(7%)Find the first three nonzero terms in the Taylor expansion of f(z)around. 5-3cos 8 (2)(7%)Find and classify the isolated singularities in the extended complex plane of f(z). (3)(6%)Evaluate the integral (2)(10%)Find the Fourier transform of 1 )=x2+x+丙
2015-2016 学年第一学期《复变函数与积分变换》期终考试试卷--2 3. Set 𝑓(𝑧) = 1 1−𝑠𝑖𝑛𝑧 (1) (7%) Find the first three nonzero terms in the Taylor expansion of 𝑓(𝑧) around 0. (2) (7%) Find and classify the isolated singularities in the extended complex plane of 𝑓(𝑧). (3) (6%) Evaluate the integral ∫ 𝑓(𝑧) 𝑧 𝑑𝑧 |𝑧|=2 4.(1) (10%)Evaluate the integral by using residue theorem ∫ sin 𝜃 𝑑𝜃 5 − 3 cos 𝜃 2𝜋 0 (2) (10%)Find the Fourier transform of f(x) = 1 4x2 + 4𝑥 + 5

2015-2016学年常一学《复文西我与积2分换考枣一3 5.(10%)Solve the IVP x"(t)+2x()+x(t)=1,x(0)=1,x(0)=-1 6.(20%scta=, (1)Showthat f(z)maps lines not passing through the origin onto circles passing through the origin. (2)Show that f(z)maps circles passing through the origin onto lines not passing through the origin. (3)Show that f(z)maps circles not passing through the origin onto circles not passing through the origin
2015-2016 学年第一学期《复变函数与积分变换》期终考试试卷--3 5. (10%) Solve the IVP 𝑥 ′′(𝑡) + 2𝑥′(𝑡) + 𝑥(𝑡) = 1, 𝑥(0) = 1, 𝑥 ′(0) = −1. 6. (20%) Set (𝑧) = 1 𝑧 . (1) Show that 𝑓(𝑧) maps lines not passing through the origin onto circles passing through the origin. (2) Show that 𝑓(𝑧) maps circles passing through the origin onto lines not passing through the origin. (3) Show that 𝑓(𝑧) maps circles not passing through the origin onto circles not passing through the origin