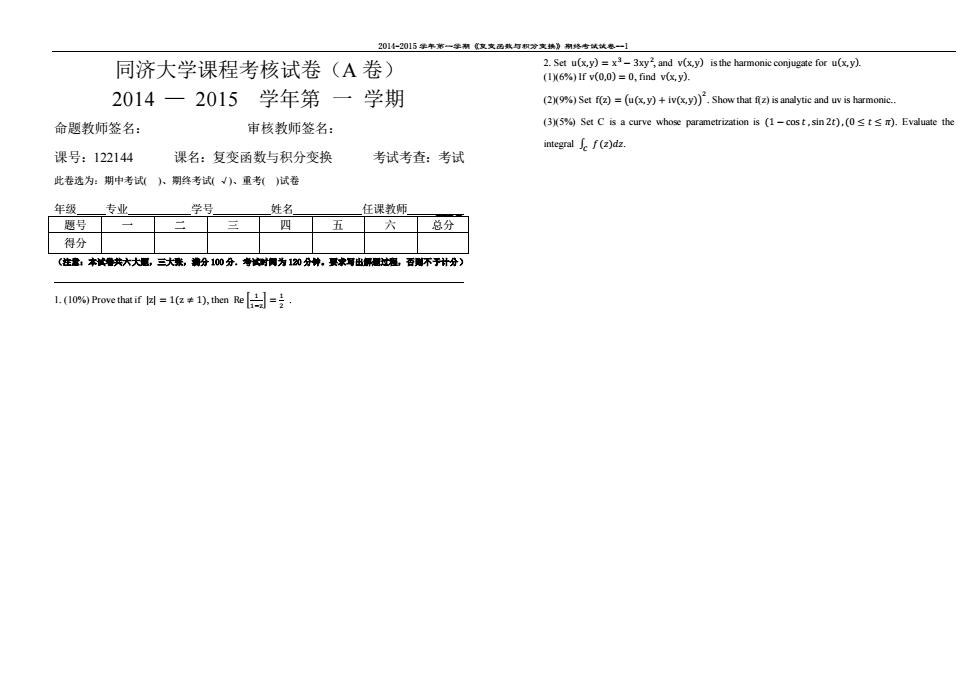
201-2015学年一生湘《支西戴与积2分支》以状枣一】 同济大学课程考核试卷(A卷) 2.Set u(x.y)=x3-3xy2,and v(x,y)is the harmonic conjugate for u(x,y) (I(6%)fv(0,0)=0,find v(x,y. 2014-2015 学年第一 学期 (2)%)Set f(z)=(u(xy)+iv(x.y)Show that f(z)is analytic and uv is harmonic. 命题教师签名: 审核教师签名: (3)(5%)Set C is a curve whose parametrization is (1-cost,sin 2t),().Evaluate the integral ff(z)dz. 课号:122144 课名:复变函数与积分变换 考试考查:考试 此卷进为:期中考试(、期终考试()、重考数)试卷 年级 专业 学号」 姓名 任课教师 题号 六总分 得分 (注这:本试增共大大愿,三大张,满分100分.考的时间为120分钟,具球可出铜圆过复,否测不于计分) l.0%)Prove that if以=1e≠10,then Re周=
2014-2015 学年第一学期《复变函数与积分变换》期终考试试卷--1 同济大学课程考核试卷(A 卷) 2014 — 2015 学年第 一 学期 命题教师签名: 审核教师签名: 课号:122144 课名:复变函数与积分变换 考试考查:考试 此卷选为:期中考试( )、期终考试( √)、重考( )试卷 年级 专业 学号 姓名 任课教师 ___ _ 题号 一 二 三 四 五 六 总分 得分 (注意:本试卷共六大题,三大张,满分 100 分.考试时间为 120 分钟。要求写出解题过程,否则不予计分) 1. (10%) Prove that if |z| = 1(z ≠ 1), then Re [ 1 1−z ] = 1 2 . 2. Set u(x,y) = x 3 − 3xy2 , and v(x,y) is the harmonic conjugate for u(x, y). (1)(6%) If v(0,0) = 0, find v(x, y). (2)(9%) Set f(z) = (u(x, y) + iv(x,y)) 2 . Show that f(z) is analytic and uv is harmonic.. (3)(5%) Set C is a curve whose parametrization is (1 − cos 𝑡 , sin 2𝑡) , (0 ≤ 𝑡 ≤ 𝜋). Evaluate the integral ∫ 𝑓(𝑧)𝑑𝑧 𝐶

201-2015学年米一学湘《区文西与积分状一2 3sa园= 4.(1)(%)Evaluate the integral (1)(7%)Find the first two nonzero terms in the Taylor expansion of fz)around0. (2)(7%)Find the first two nonzero terms in the Laurent expansion of fz)around 1. (3)(6%)Does the limit f包 exi过?Prove your conclusion. (2)(10%)Find the Fourier transform of 00=x2+x+5
2014-2015 学年第一学期《复变函数与积分变换》期终考试试卷--2 3. Set f(z) = 𝑒 𝑧−1 𝑧(1−𝑧) (1) (7%) Find the first two nonzero terms in the Taylor expansion of f(z) around 0. (2) (7%) Find the first two nonzero terms in the Laurent expansion of f(z) around 1. (3) (6%) Does the limit lim𝑧→∞ 𝑓(𝑧) exist? Prove your conclusion. 4.(1) (10%)Evaluate the integral ∫ 𝑑𝑧 z 2sin𝑧 |𝑧|=4 (2) (10%)Find the Fourier transform of f(x) = 1 x 2 + 4𝑥 + 5

201-2015学年第一学和《复文西我与积2分变编》有终考就状枣一3 5.(10%)Solve the IVP 6.(1)(5%)What is the image under the mapping w=(z-a+bi)/(z+a+bi)of [Re z20), x"()-6x'()+5x(t)=cost,x(0)=0,x'(0)=0. where a is positive and b is real. )5)Prove thaT因=maps the half-plane (Re>o啡ono the die-0 onregicn contained in [Re z>0),where ak is positive,bk is real and Rezk0. (4)(5%)Prove that if a rational function f(z)can be written as the composition TooT.Tn(z), where To.T..Tn are defined as (2)and (3),then all the poles of f(z)have negative real part
2014-2015 学年第一学期《复变函数与积分变换》期终考试试卷--3 5. (10%) Solve the IVP x ′′(t) − 6x′(t) + 5x(t) = cos 𝑡, x(0) = 0, x ′(0) = 0. 6. (1) (5%) What is the image under the mapping w = (z − a + bi)/(z + a + bi) of {Re z ≥ 0}, where a is positive and b is real. (2) (5%) Prove that T0 (z) = a0 z+a0+ib0 maps the half-plane {Re z > 0} onto the disc {|w− 1 2 | 0} onto a region contained in {Re z > 0}, where ak is positive, bk is real and Re z𝑘 ≥ 0. (4) (5%) Prove that if a rational function f(z) can be written as the composition 𝑇0 ∘ 𝑇1 ∘ ⋯ ∘ 𝑇𝑛(𝑧), where 𝑇0 , 𝑇1 , … , 𝑇𝑛 are defined as (2) and (3), then all the poles of f(z) have negative real part