
ME369 Modeling,Analysis and System Control-A --Lecture 141029 Week 7# Ch5.3~5. Oct.27(M) Transient,second order system, spec. 6 Transient,higher order system Ch5.45. Oct.29(W) HW4 Stability,Routh Criterion 7 Week 8# Routh Criterion Ch5.7 Nov.3(M) HW3 due Steady State Error Ch5.8 Practical lecture 1-control Nov.5(W) system modeling Quiz 2
ME369 Modeling, Analysis and System Control-A --Lecture_141029 Week 7# Oct.27(M) Transient, second order system, spec. Ch5.3~5. 6 Oct.29(W) Transient, higher order system Stability, Routh Criterion Ch5.4~5. 7 HW4 Week 8# Nov.3(M) Routh Criterion Steady State Error Ch5.7 Ch5.8 HW3 due Nov.5(W) Practical lecture 1 – control system modeling Quiz 2

Problems of HW4 ·Refer to the ftp site. ftp://public.sjtu.edu.cn ·User:yuanjj ·Password:public DUE:Nov.12(W)
Problems of HW4 • Refer to the ftp site. • ftp://public.sjtu.edu.cn • User: yuanjj • Password: public DUE: Nov.12(W)
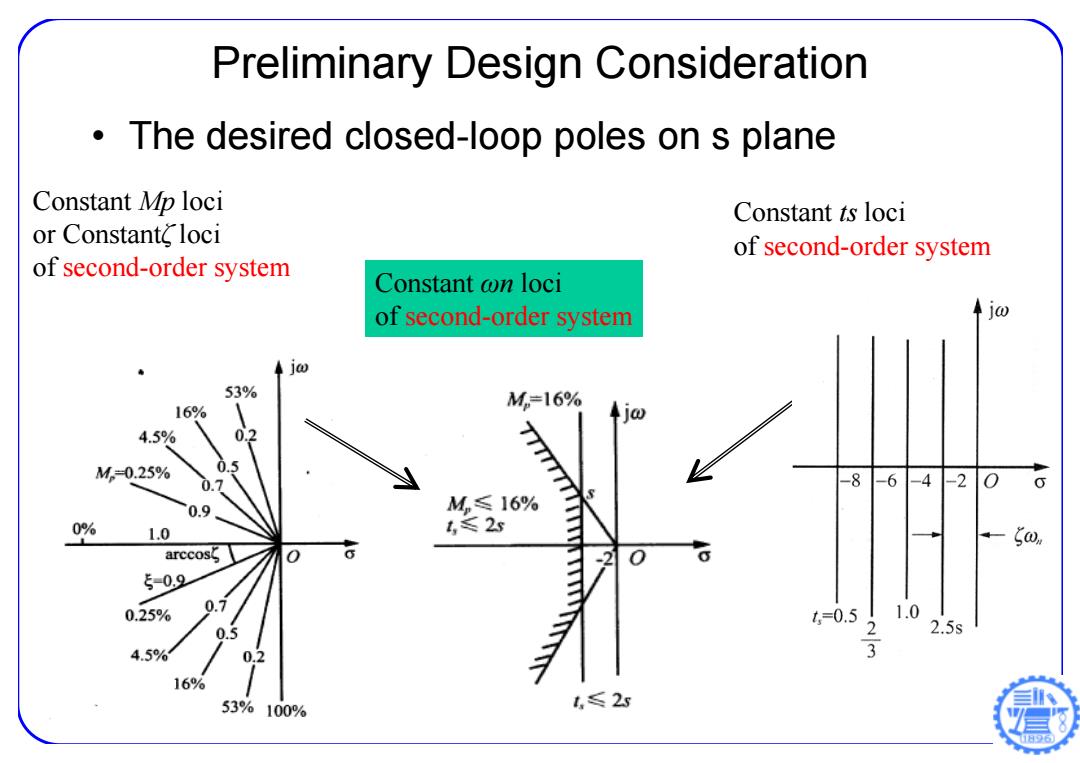
Preliminary Design Consideration The desired closed-loop poles on s plane Constant Mp loci Constant ts loci or Constant loci of second-order system of second-order system Constant wn loci of second-order system ◆j 53% M=16% 16% jω 4.5% 0.2 M-0.25% 0.5 0.7 -8 -6 -4 -2 O 0.9 M≤16% 0% 1.0 1≤2 o, arccos 509 0.25% 0.7 1=0.5 1.0 0.5 2.5s 4.5% 0.2 3 16% 53%100% 1≤2s
Preliminary Design Consideration • The desired closed-loop poles on s plane Constant Mp loci or Constant ζ loci of second-order system Constant ts loci of second-order system Constant ω n loci of second-order system

Exercise #1 A certain servomechanism system has dynamics dominated by a pair of complex poles and no finite zeros.The time-domain specifications on the rise time,settling time,and maximum overshoot are given by, rise from 10%to 90% M,≤17% t,≤4.6sec t,≤l.2sec Sketch the region in the s-plane where the poles could be placed so that the system will meet all three specifications. M,=e ≤0.17→6≥0.491 on≥1.5rad/s
A certain servomechanism system has dynamics dominated by a pair of complex poles and no finite zeros. The time-domain specifications on the rise time, settling time, and maximum overshoot are given by, 1.2sec? r t 4.6sec s 17% t M p Sketch the region in the s-plane where the poles could be placed so that the system will meet all three specifications. Exercise #1 rise from 10% to 90% 0.17 0.491 2 1 M e p 1.5 / n rad s
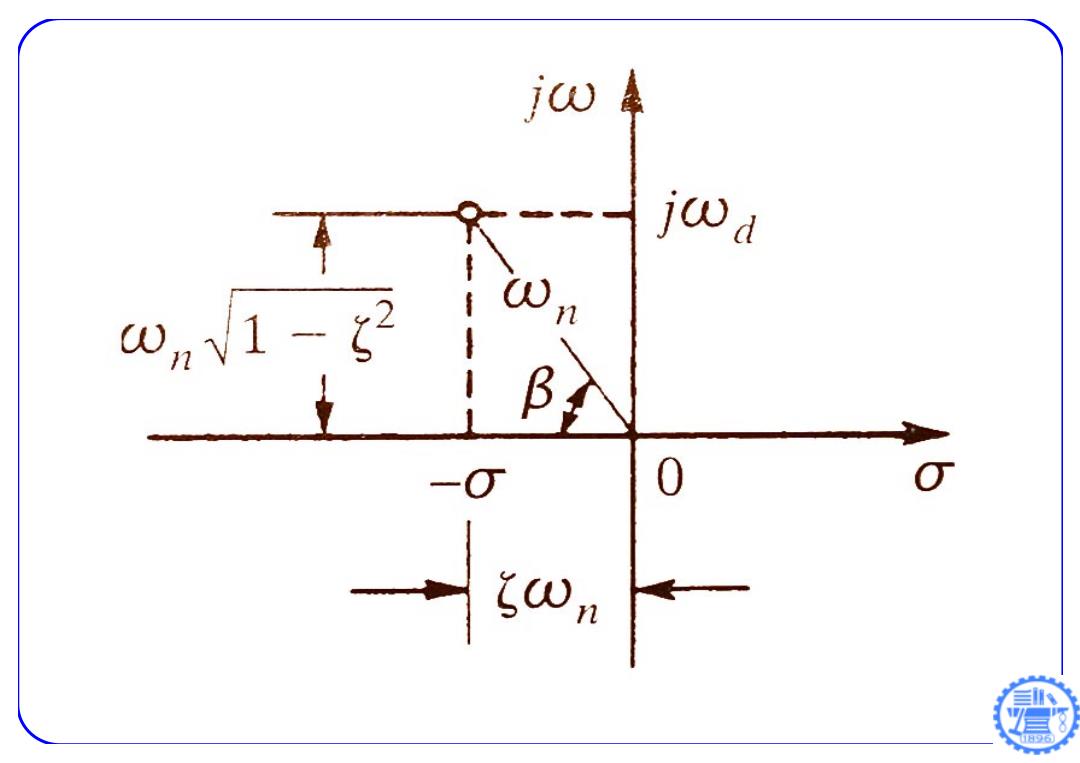
j0率 jwd wnv1-22 n B 0 O 日6
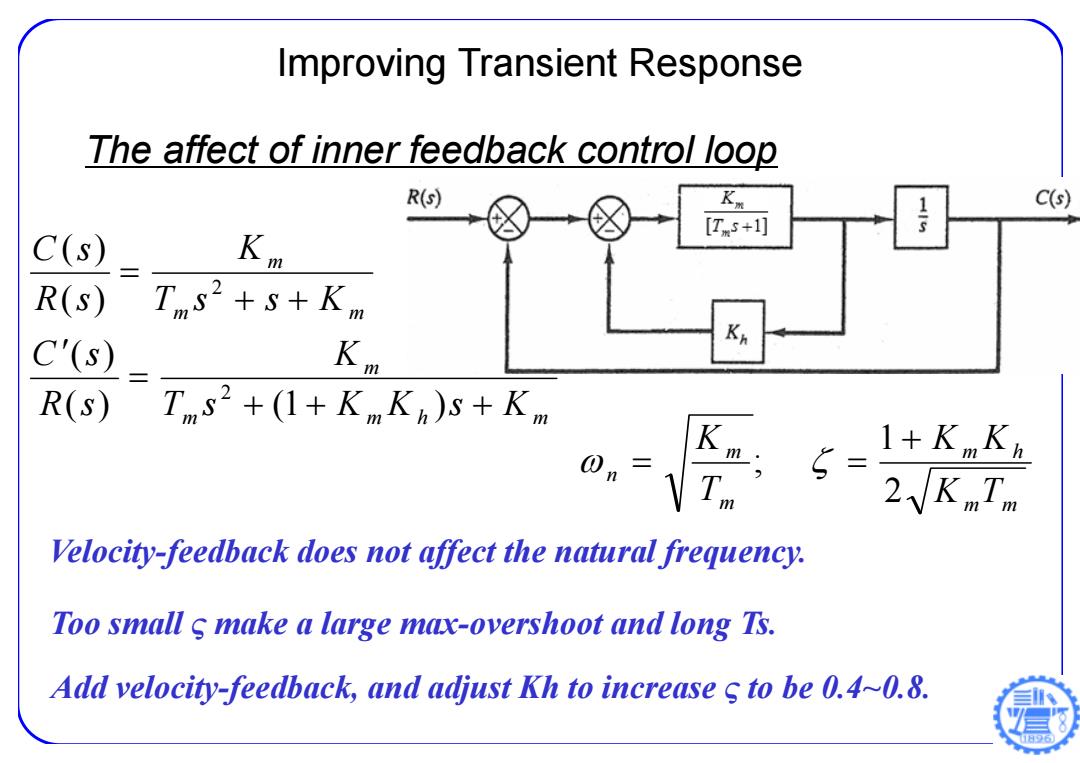
Improving Transient Response The affect of inner feedback control loop R(s) C(s) [Ts+1] C(s) Km R(s) Tms2+s+K C'(s) K Kh m R(s) Ts2+(1+KmKn)s+Km K, m 5= 1+KmKn 2KmTm Velocity-feedback does not affect the natural frequency. Too small make a large max-overshoot and long Ts. Add velocity-feedback,and adjust Kh to increase s to be 0.4-0.8
Improving Transient Response The affect of inner feedback control loop Velocity-feedback does not affect the natural frequency. Add velocity-feedback, and adjust Kh to increase ς to be 0.4~0.8. m m h m m m m m T s K K s K K R s C s T s s K K R s C s ( ) ( 1 ) ( ) ( ) ( ) 2 2 m m m h m m n K T K K T K 2 1 ; Too small ς make a large max-overshoot and long Ts

Example 5-2,Ogata,pp.236,V4 e For system with J=2kgm2,and b=1Nms,determine the values of K and K,so that the max-overshoot is 0.2 and the peak time is 1s. R(s) K C(s) 5+b Mn=e-G=0.2→5=0.456 π π tp =1→on=3.53rad/s 0a0nV1-52 =K/J=3.53rad/sK=24.92Nm K。=2(26@J-b)=0.218s K
Example 5-2, Ogata, pp.236, V4 • For system with J=2kgm 2, and b=1Nms, determine the values of K and K h, so that the max-overshoot is 0.2 and the peak time is 1s. 0.2 0.456 2 / 1 M e p t rad s n n d p 1 3.53 / 1 2 / 3.53 / 24.92 n K J rad s K Nm 1 (2 ) 0.218 K Jb s h n K
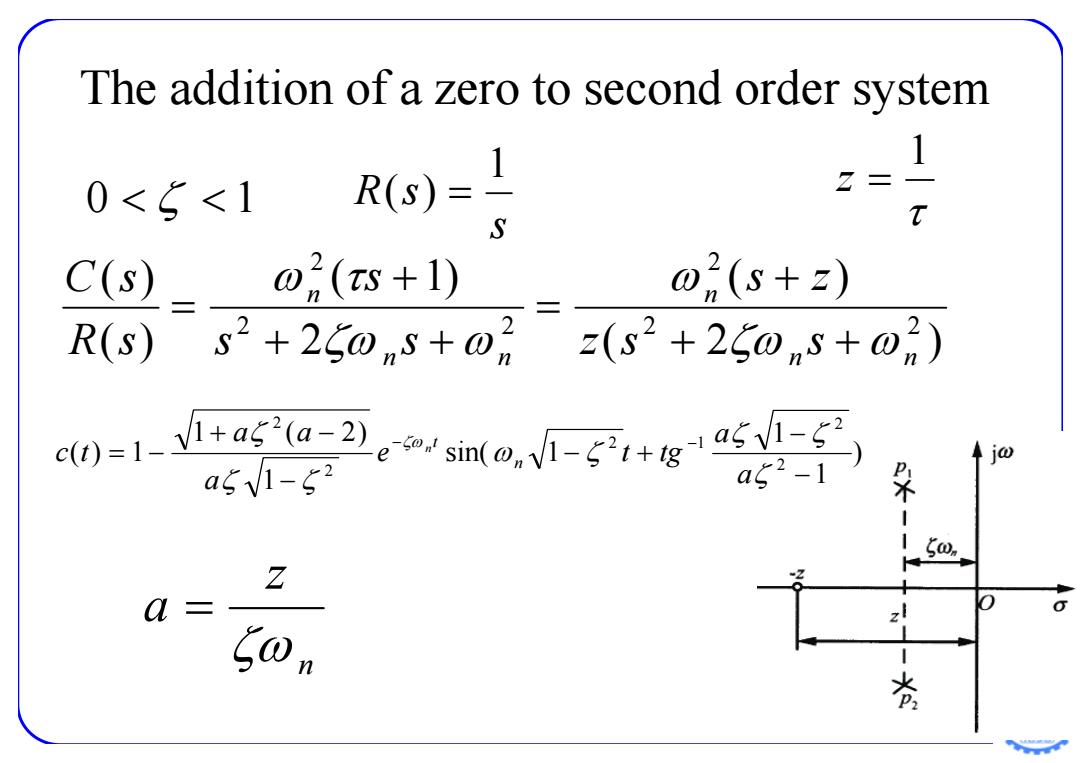
The addition of a zero to second order system 1 1 0<5<1 R(S)= Z= S T C(s) o2(S+1) 02(s+z) R(s) s2+260ns+07 z(s2+250nS+0) 0-1。wu发 a5V1-52 Z 2 a z 为
( 2 ) ( ) 2 ( 1) ( ) ( ) 2 2 2 2 2 2 n n n n n n z s s s z s s s R s C s 0 1 1 z s R s 1 ( ) ) 1 1 sin( 1 1 1 ( 2) ( ) 1 2 2 2 1 2 2 a a e t tg a a a c t n t n n z a The addition of a zero to second order system
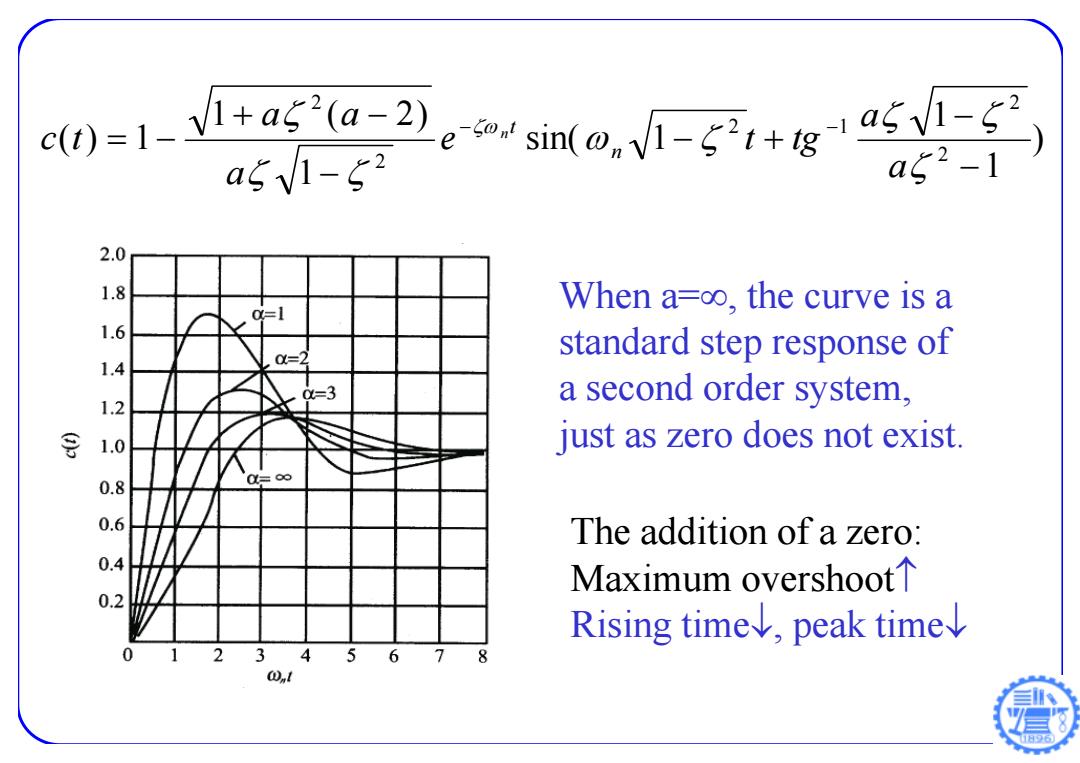
a012可,me-+gaf a5V1-52 2.0 1.8 When a=oo,the curve is a 0=1 1 14 0=2 standard step response of 0=3 a second order system, 1.2 号 1.0 just as zero does not exist. 0.8 0=∞ 0.6 The addition of a zero: 0.4 Maximum overshoot'个 0.2 Rising timev,peak time 0 2 6 7 8 @
When a=, the curve is a standard step response of a second order system, just as zero does not exist. ) 1 1 sin( 1 1 1 ( 2) ( ) 1 2 2 2 1 2 2 a a e t tg a a a c t n t n The addition of a zero: Maximum overshoot Rising time, peak time
The addition of a zero to system c0=1-1+a5a=2)esin(o.V-5+ga51- a5V1-52 a2-1 Closed loop zero: Affect the magnitude and phase angle of the transient response. Does not affect decay ratio,damping ratio and the frequency >The response curve is determined by closed-loop poles. >Detailed shape is by closed-loop poles and zeros
Closed loop zero: Affect the magnitude and phase angle of the transient response. Does not affect decay ratio, damping ratio and the frequency The response curve is determined by closed-loop poles. Detailed shape is by closed-loop poles and zeros. ) 1 1 sin( 1 1 1 ( 2) ( ) 1 2 2 2 1 2 2 a a e t tg a a a c t n t n The addition of a zero to system