
Lecture 9 Power System Optimal Power Flow Dr.QI Huang School of Energy Science and Engineering,UESTC
Lecture 9 Power System Optimal Power Flow Dr. QI Huang School of Energy Science and Engineering, UESTC

Contents Introduction Optimal power flow model Solution of optimal power flow by linear programming 电力拿镜广城洲量写控制四川省重点实验蜜 家电
电力系统广域测量与控制四川省重点实验室 Contents Introduction Optimal power flow model Solution of optimal power flow by linear programming
Introduction Optimal power flow:a stable system operation status,while operation and security constraints s are satisfied,achieved by adjusting those controllable variables to realize the optimization of specific targets. First proposed by French scientist Carpentier in 60's More optimal than ED:more constraints are considered; more controllable variables (MW) ■ Generator Voltage Tap changer Synchronous condenser Switching capacitor ■ SVC Load shedding ■ DC power flow 电力拿镜广城洲量写控制四川省童点实验蜜 国家电网
电力系统广域测量与控制四川省重点实验室 Introduction Optimal power flow: a stable system operation status, while operation and security constraints are satisfied, achieved by adjusting those controllable variables to realize the optimization of specific targets. First proposed by French scientist Carpentier in 60’s More optimal than ED: more constraints are considered; more controllable variables(MW) Generator Voltage Tap changer Synchronous condenser Switching capacitor SVC Load shedding DC power flow
Introduction Assumptions in optimal power flow The thermal(nuclear)plant commissioned is given(start up/shutdown is neglected) The output of every hydroplant is given(hydroplant ED) Power system structure remains unchanged Objective function of OPF: Min of operation cost:max of GenCO profit Min of active power loss:energy efficiency,improvement of voltage quality In order to guarantee the convex property of objective function,quadratic function is used (large than 3rd order may not guarantee convex) 电力拿镜广城洲量写控制四川省童点实验蜜 家电网
电力系统广域测量与控制四川省重点实验室 Introduction Assumptions in optimal power flow The thermal (nuclear) plant commissioned is given (start up/shutdown is neglected) The output of every hydroplant is given (hydroplant ED) Power system structure remains unchanged Objective function of OPF: Min of operation cost: max of GenCO profit Min of active power loss: energy efficiency, improvement of voltage quality In order to guarantee the convex property of objective function, quadratic function is used (large than 3rd order may not guarantee convex)
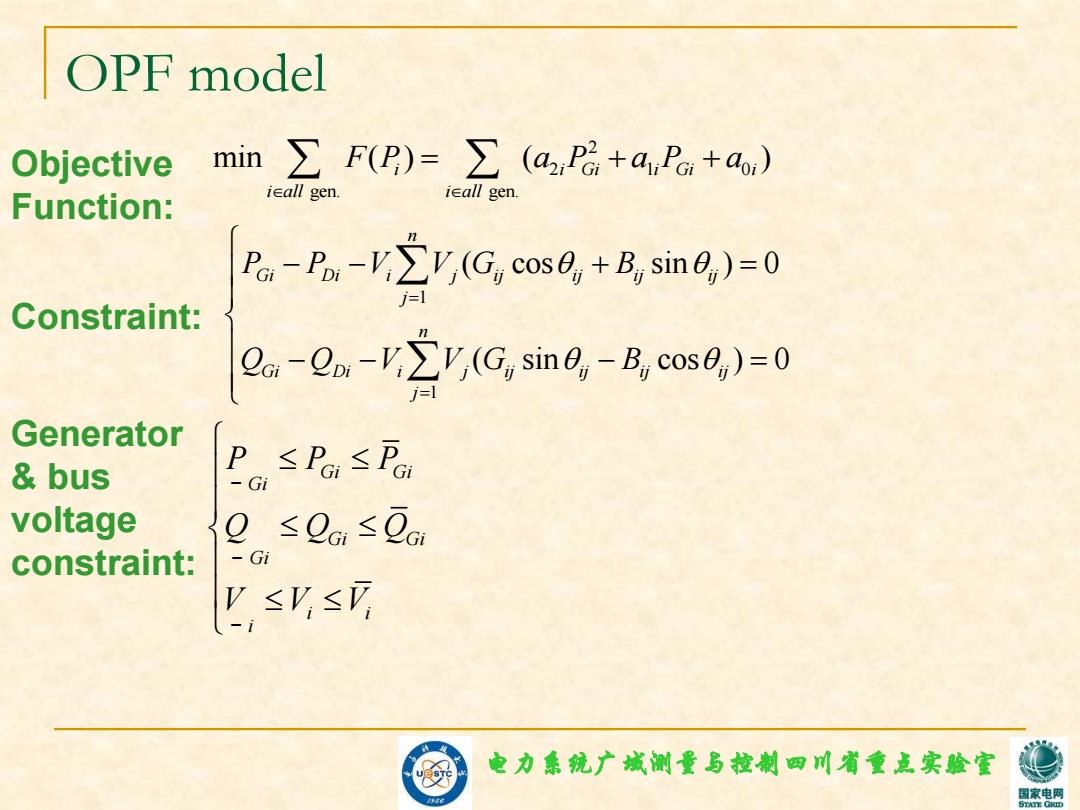
OPF model Objective min ∑F(P)=∑(a,P+a,P+a,) Function: ieall gen ieall gen. Po-Po-V V,(G cos0 +B sin)=0 Constraint: Q-0-25(G,sn8-R,os0,)=0 Generator bus P。≤PaSFa voltage Q≤Qo≤0a constraint: -Gi '≤r≤ 电力集院广城测量与控制四川省重点实验蜜 家电
电力系统广域测量与控制四川省重点实验室 OPF model 2 2 1 0 gen. gen. min ( ) ( ) i i Gi i Gi i i all i all F P a P a P a 1 1 ( cos sin ) 0 ( sin cos ) 0 n Gi Di i j ij ij ij ij j n Gi Di i j ij ij ij ij j P P V V G B Q Q V V G B Gi Gi Gi Gi Gi Gi i i i P P P Q Q Q V V V Objective Function: Constraint: Generator & bus voltage constraint:

Solution of OPF Lambda-iterative: Gradient:slow convergence;cannot handle inequality constraints Newton method:fast convergence;may have problem with inequality constraints Linear Programming:mature algorithm;linearize the nonlinear objective function and constraints ■ Non-linear programming:handle non-linear objective function and constraint (equality or inequality) Interior point method:widely used;easy for handling inequality constraints 电力拿镜广城洲量写控制四川省重点实验蜜 家电网
电力系统广域测量与控制四川省重点实验室 Solution of OPF Lambda-iterative: Gradient: slow convergence; cannot handle inequality constraints Newton method: fast convergence; may have problem with inequality constraints Linear Programming: mature algorithm; linearize the nonlinear objective function and constraints Non-linear programming: handle non-linear objective function and constraint (equality or inequality) Interior point method: widely used; easy for handling inequality constraints

Gradient method min ∑F(E)=∑(a,P+a,P+a) ieall gen. ieall gen. PQ bus X= on the reference bus IEI PV bus P y= on each PQ bus control or adjustable variables V- P > p fixed or constant variables on each QV bus 电力拿镜广城洲量写控制四川省重点实验蜜 家电
电力系统广域测量与控制四川省重点实验室 Gradient method 2 2 1 0 gen. gen. min ( ) ( ) i i Gi i Gi i i all i all F P a P a P a PQ bus | | PV bus i i i E x on the reference bus | | on each PQ bus on each QV bus | | k k net k net k net k sch k E P Q P E y control or adjustable variables fixed or constant variables u y p
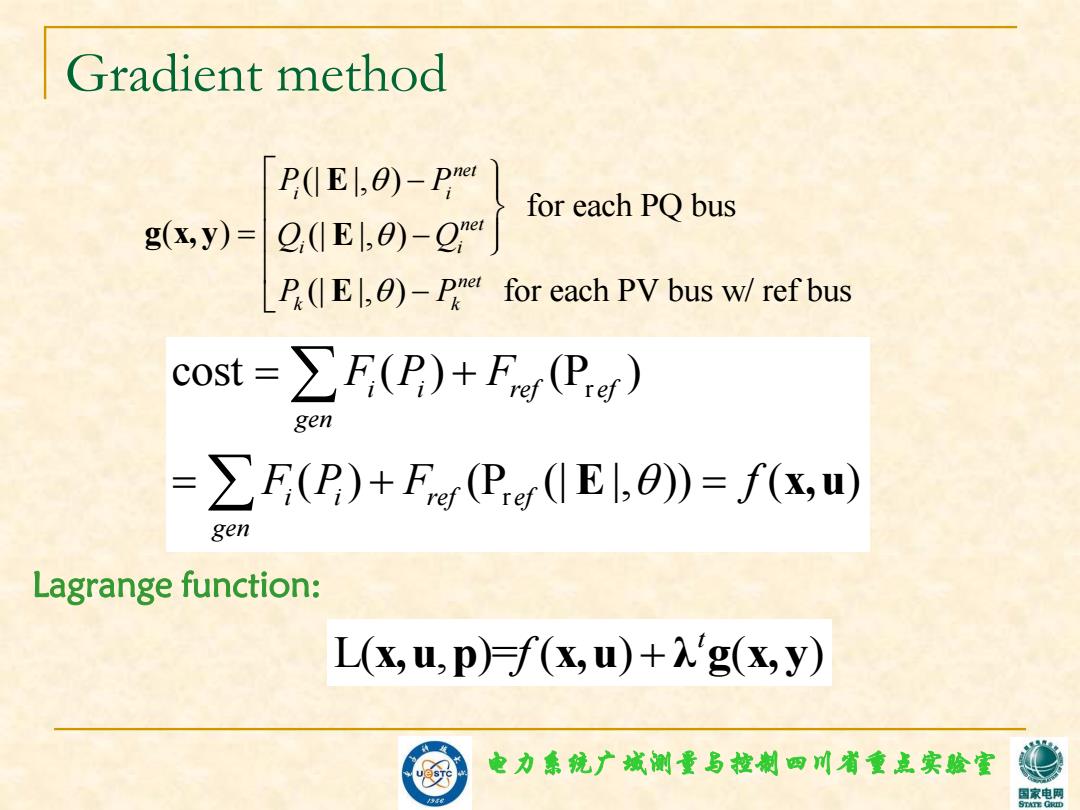
Gradient method P(El,0)-Pe for each PQ bus g(xy)=Q,E1,0)-g P.(1El0)-Pe for each PV bus w/ref bus cost=∑F(P)+Fgr(Per) gen =∑F(P)+Fer(Per(El,θ)=f(&,m) gen Lagrange function: L(x,u,p)(x,u)+g(x,y) 电力拿镜广城洲量写控制四川省重点实验蜜 家电
电力系统广域测量与控制四川省重点实验室 Gradient method (| |, ) for each PQ bus ( ) (| |, ) (| |, ) for each PV bus w/ ref bus net i i net i i net k k P P Q Q P P E g x, y Ε E r r cost ( ) (P ) ( ) (P (| |, )) ( ) i i ref ef gen i i ref ef gen F P F F P F f E x,u Lagrange function: L( , )= ( ) ( ) t x,u p x,u f λ g x, y
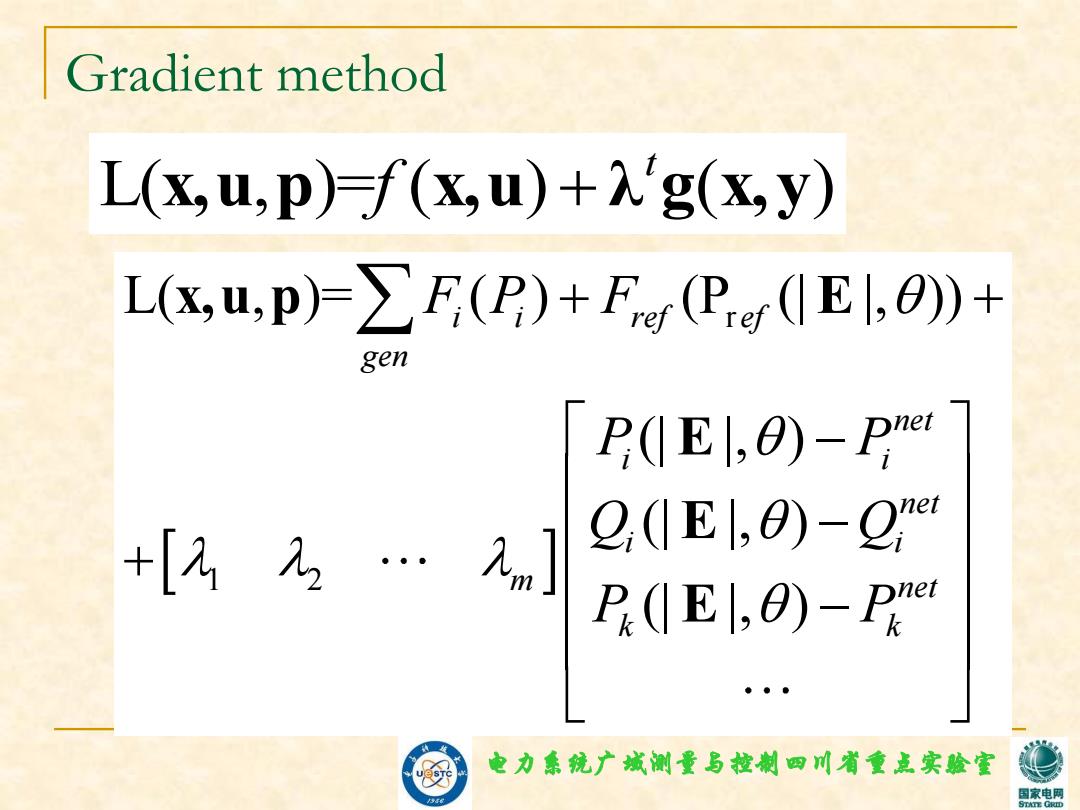
Gradient method L(x,u,p)f(x,u)+g(x,y) L(x,u,p)=>F(P)+Fr(Prer(E,))+ gen P(E,0)-Pmet +[乃….n] Q,(E,0)-9"e P(El,)-Pme 电力康镜广城测量与控制四川省重点实验蜜 家电
电力系统广域测量与控制四川省重点实验室 Gradient method L( , )= ( ) ( ) t x,u p x,u f λ g x, y r 1 2 L( , )= ( ) (P (| |, )) (| |, ) (| |, ) (| |, ) i i ref ef gen net i i net i i m net k k F P F P P Q Q P P x,u p E E Ε E
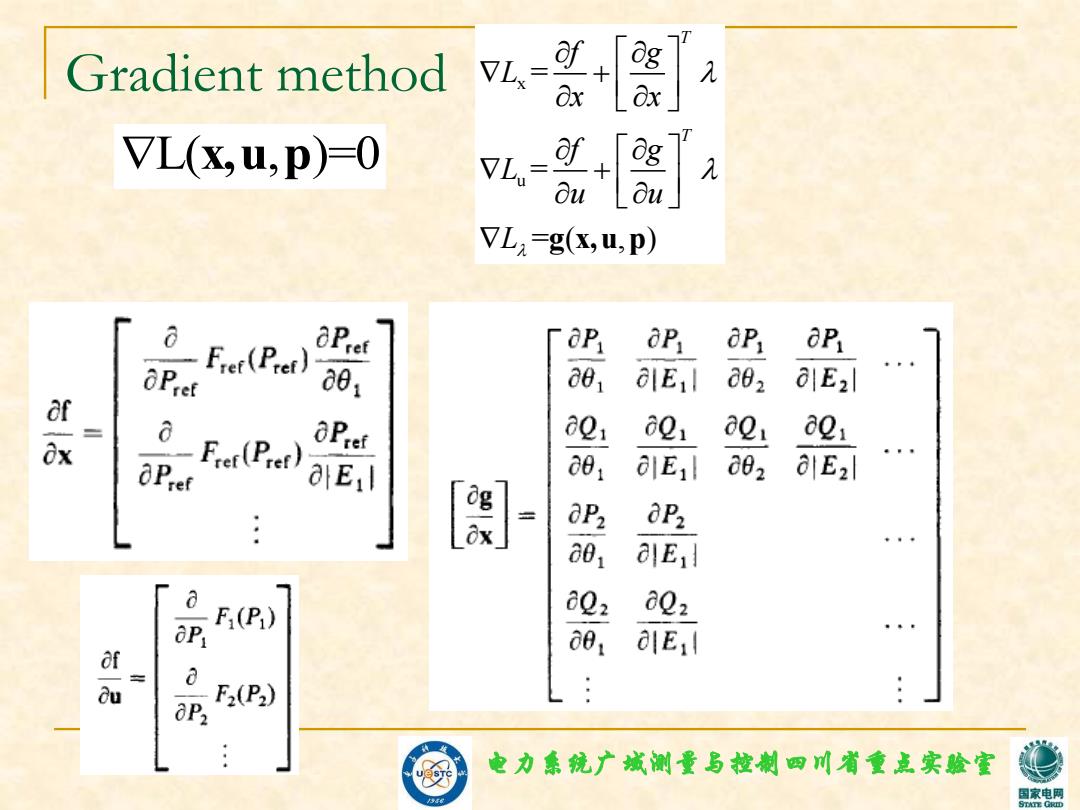
Gradient method VL 0g VL(x,u,p)-0 V 时 og Bu VL2-g(x,U,p) a 胎, OP OP aP Pret a01 lE,l a82 aE21 0x Pret 2 01 0Q1 01 aE:l 6JE,l a82 aE21 [ 码湖 OP2 alE,l a 0 a02 OP F(P) , alE:l of Qu 0P F2(P) 电力拿镜广城洲量写控制四川省重点实验蜜 家电
电力系统广域测量与控制四川省重点实验室 Gradient method L( , )=0 x,u p x u = = = ( , ) T T f g L x x f g L u u L g x,u p