
3.1 NEURONAL DYNAMICAL SYSTEM Neuronal activations change with time.The way they change depends on the dynamical equations as following: x=g(Fx,Fy…) (3-1) y=h(Fx,Fy,…y (3-2) 2006.10.9
2006.10.9 3.1 NEURONAL DYNAMICAL SYSTEM Neuronal activations change with time. The way they change depends on the dynamical equations as following: x = g(FX ,FY ,) • y = h(FX ,FY ,) • (3-1) (3-2)
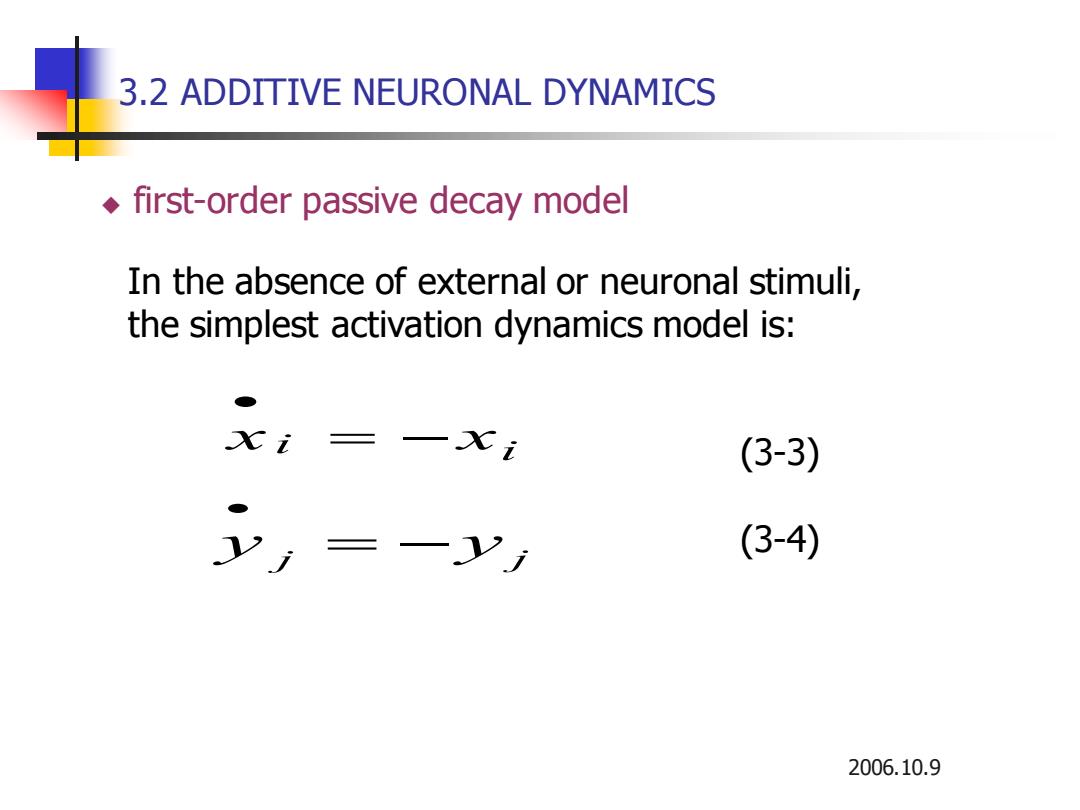
3.2 ADDITIVE NEURONAL DYNAMICS first-order passive decay model In the absence of external or neuronal stimuli, the simplest activation dynamics model is: xi=一xi (3-3) (3-4) 2006.10.9
2006.10.9 In the absence of external or neuronal stimuli, the simplest activation dynamics model is: i xi = −x • y j = −yj • 3.2 ADDITIVE NEURONAL DYNAMICS (3-3) (3-4) ◆ first-order passive decay model
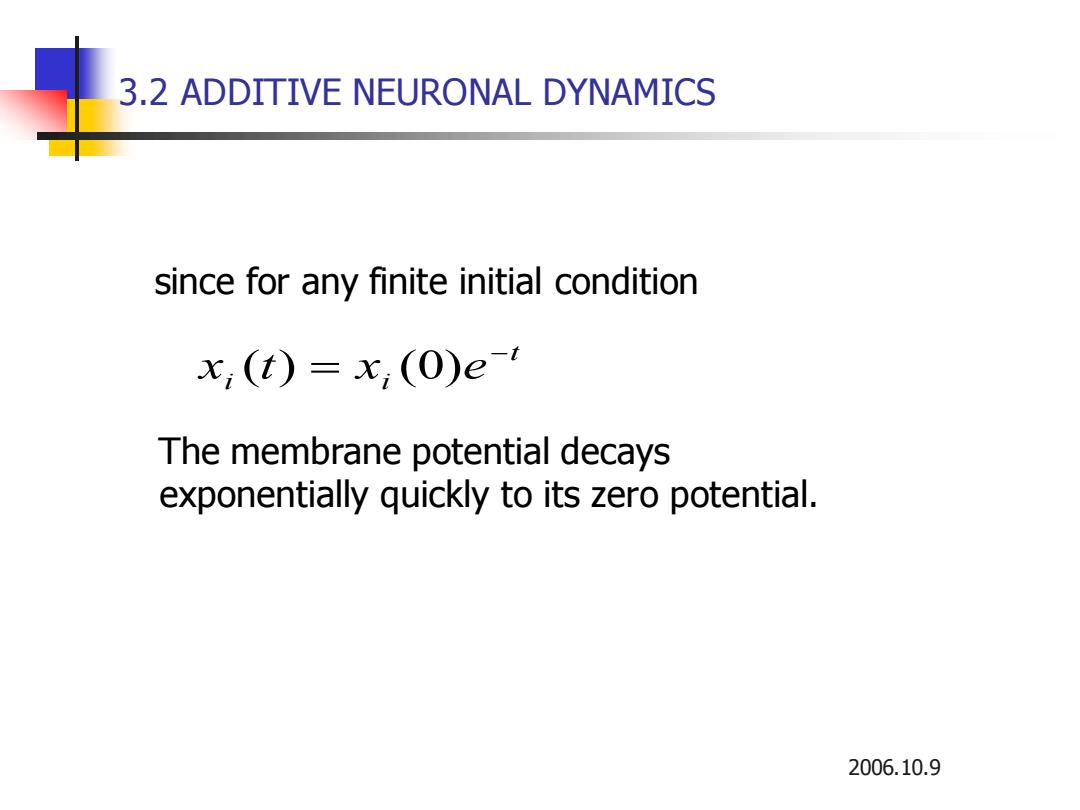
3.2 ADDITIVE NEURONAL DYNAMICS since for any finite initial condition x,(t)=x,(0)et The membrane potential decays exponentially quickly to its zero potential. 2006.10.9
2006.10.9 3.2 ADDITIVE NEURONAL DYNAMICS t xi t xi e − ( ) = (0) since for any finite initial condition The membrane potential decays exponentially quickly to its zero potential
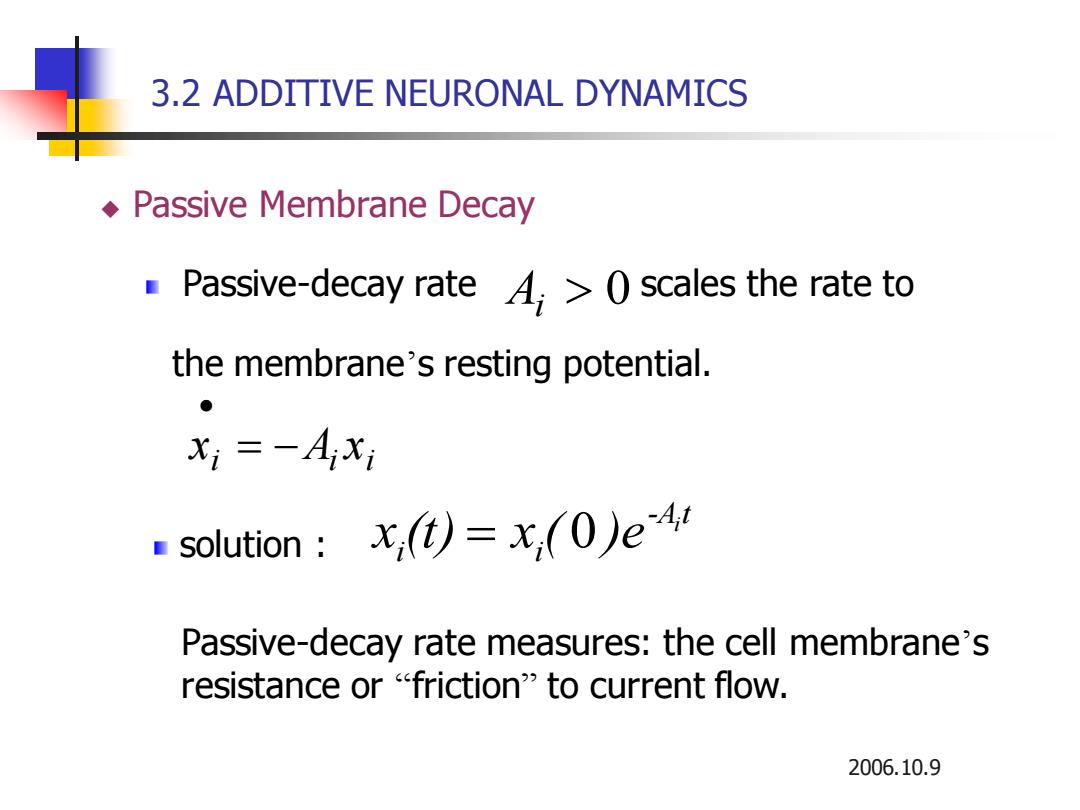
3.2 ADDITIVE NEURONAL DYNAMICS Passive Membrane Decay Passive-decay rate ,>0 scales the rate to the membrane's resting potential. xi =-Ajxi solution:. ,()=x,(0)e4 Passive-decay rate measures:the cell membrane's resistance or friction"to current flow. 2006.10.9
2006.10.9 Passive-decay rate Ai 0 scales the rate to the membrane’s resting potential. solution : Passive-decay rate measures: the cell membrane’s resistance or “friction” to current flow. i i i x = −A x • 3.2 ADDITIVE NEURONAL DYNAMICS ◆ Passive Membrane Decay -A t i i i x (t) = x ( 0 )e

property Pay attention to 4;property The larger the passive-decay rate,the faster the decay--the less the resistance to current flow
Ai The larger the passive-decay rate,the faster the decay--the less the resistance to current flow. Pay attention to property property
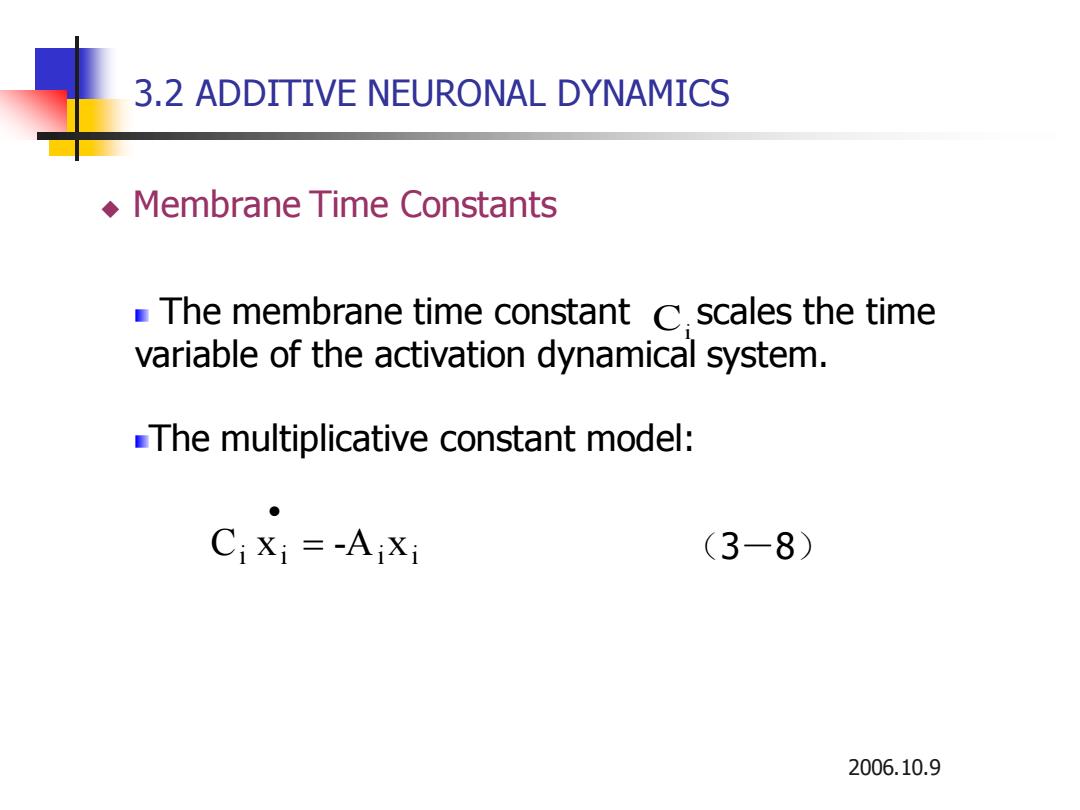
3.2 ADDITIVE NEURONAL DYNAMICS Membrane Time Constants The membrane time constant C.scales the time variable of the activation dynamical system. The multiplicative constant model: Cixi=-AiXi (3一8) 2006.10.9
2006.10.9 The membrane time constant scales the time variable of the activation dynamical system. The multiplicative constant model: Ci i i i i C x = -A x • (3-8) 3.2 ADDITIVE NEURONAL DYNAMICS ◆ Membrane Time Constants

Solution and property ■solution x;(t)=x,(0)e property The smaller the capacitance the faster things change As the membrane capacitance increases toward positive infinity,membrane fluctuation slows to stop
Solution and property solution t C A i i i t x e − x ( ) = (0) i property The smaller the capacitance ,the faster things change As the membrane capacitance increases toward positive infinity,membrane fluctuation slows to stop
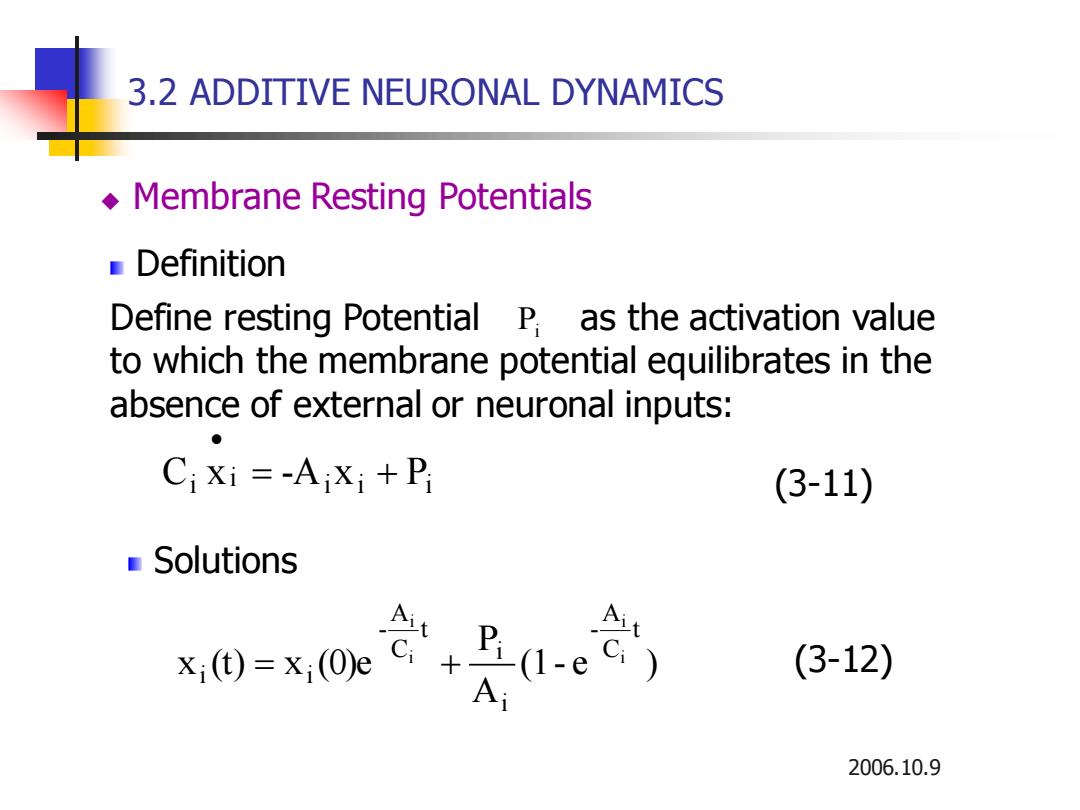
3.2 ADDITIVE NEURONAL DYNAMICS Membrane Resting Potentials Definition Define resting Potential P as the activation value to which the membrane potential equilibrates in the absence of external or neuronal inputs: Cixi=-AiXi+P (3-11) Solutions Ait x1(t)=x;(0)e + (3-12) 2006.10.9
2006.10.9 3.2 ADDITIVE NEURONAL DYNAMICS Define resting Potential as the activation value to which the membrane potential equilibrates in the absence of external or neuronal inputs: Pi i i i i Ci x = -A x + P • Solutions (1- e ) A P x (t) x (0)e t C A - i i t C A - i i i i i i = + Definition (3-11) (3-12) ◆ Membrane Resting Potentials

Note The time-scaling capacitance dose not affect the asymptotic or steady-state solution. The steady-state solution does not depend on the finite initial condition. In resting case,we can find the solution quickly
Note The time-scaling capacitance dose not affect the asymptotic or steady-state solution. The steady-state solution does not depend on the finite initial condition. In resting case,we can find the solution quickly
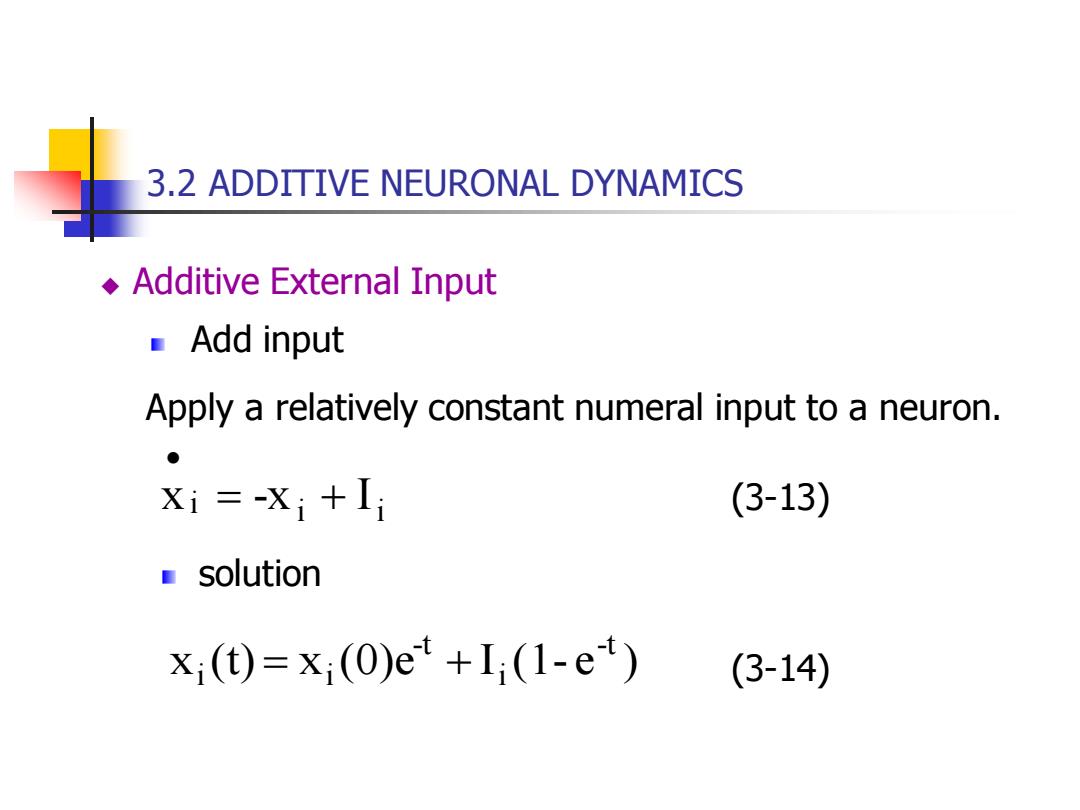
3.2 ADDITIVE NEURONAL DYNAMICS Additive External Input ■Add input Apply a relatively constant numeral input to a neuron. ● Xi=Xi+li (3-13) solution x;()=x(0)et+I;(1-et) (3-14)
Add input Apply a relatively constant numeral input to a neuron. i i xi = -x + I • solution x (t) x (0)e I (1- e ) -t i -t i = i + (3-13) (3-14) ◆ Additive External Input 3.2 ADDITIVE NEURONAL DYNAMICS