Chapter 4 Chapter 4 State Space Solutions and Realizations Objectives: Concepts of linear Ordinary Differential Equations Solution of linear state equations Similarity Transformation Discretization of state space model Realization of rational transfer functions 。Canonical forms heory
Chapter 4 Chapter 4 State Space Solutions and Realizations Objectives: • Concepts of linear Ordinary Differential Equations • Solution of linear state equations • Similarity Transformation • Discretization of state space model • Realization of rational transfer functions • Canonical forms Chapter 4 1

Chapter 4 3 4.1 Solutions of LTI system 1.Eigenvalues,Eigenvectors Solution of Differential Equations Define a linear homogeneous differential equationsystem X(t)=AX(t) or x() a a2 an 「x) 无(t) d an … 七() … … … 元0 a a… x() Suppose the solution is in the form of x,()=c,e =1,2., Substituting the solution into the equation a11 a12. ain e"= a21a2 …a2m C an an ann Cn then we get 见=A That is AC=AC So A is a eigenvalue of matrix A and vector C is the eigenvector of A
Chapter 4 2
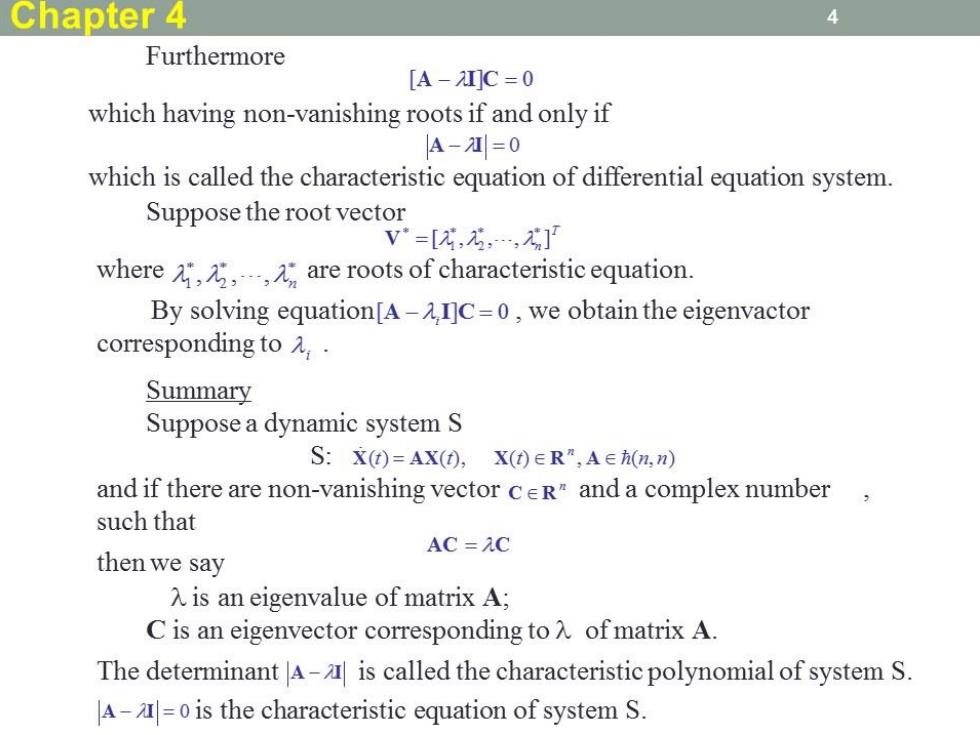
Chapter 4 4 Furthermore [A-IC=0 which having non-vanishing roots if and only if A-I=0 which is called the characteristic equation of differential equation system. Suppose the root vector '=[,5,,灯 whereare roots of characteristic equation. By solving equation[A-I]C=0,we obtain the eigenvactor corresponding to. Summary Suppose a dynamic system S S:X0=AX(0,X(0∈R,A∈nn) and if there are non-vanishing vector ceR and a complex number such that AC=AC then we say A is an eigenvalue of matrix A; C is an eigenvector corresponding to of matrix A. The determinant A-is called the characteristic polynomial of system S. A-=0 is the characteristic equation of system S
Chapter 4 3
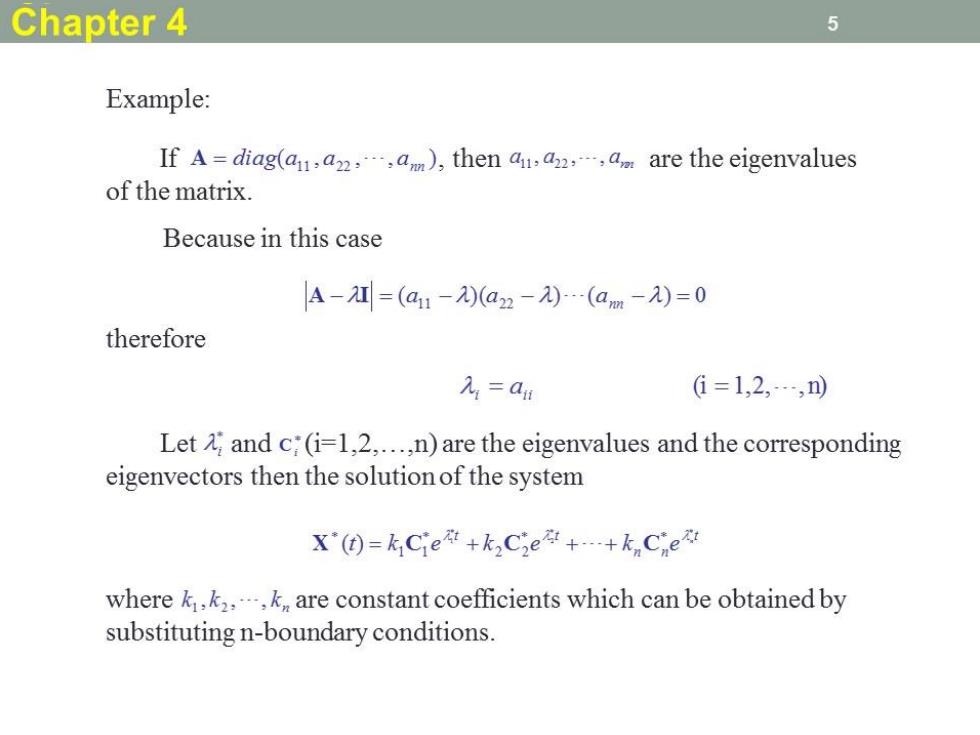
Chapter 4 5 Example: If A=diag(an.a..m),then aa are the eigenvalues of the matrix. Because in this case A-2川=(a1-0(a2-2)-(am-)=0 therefore 元=aa =1,2,,) Let and c:(i=1,2,...,n)are the eigenvalues and the corresponding eigenvectors then the solution of the system X'(t)=k Cie+kCze+Cre wherekk.,k are constant coefficients which can be obtained by substituting n-boundary conditions
Chapter 4 4

Chapter 4 6 2.State Matrix If the real part of every eigenvalues Ais negative,that is Re()<o then lim X(t)=0 the system is stable,otherwise lim X(t)=co the system is unstable. Consider the linear time-invariant control system differential equation X(t)=AX(t)+BU(t) where Aeh(n,n),Beh(n,r),X(t)ER",U()ER'is a controlvector. A is called the system matrix(系统矩阵)in open-loop case. If the controllaw is U=-KX(t),then X(t)=(A-BK)X() So the condition of stability of the closed loop control system is that the real part of every eigenvalue of matrix(A-BK)is negative
Chapter 4 5
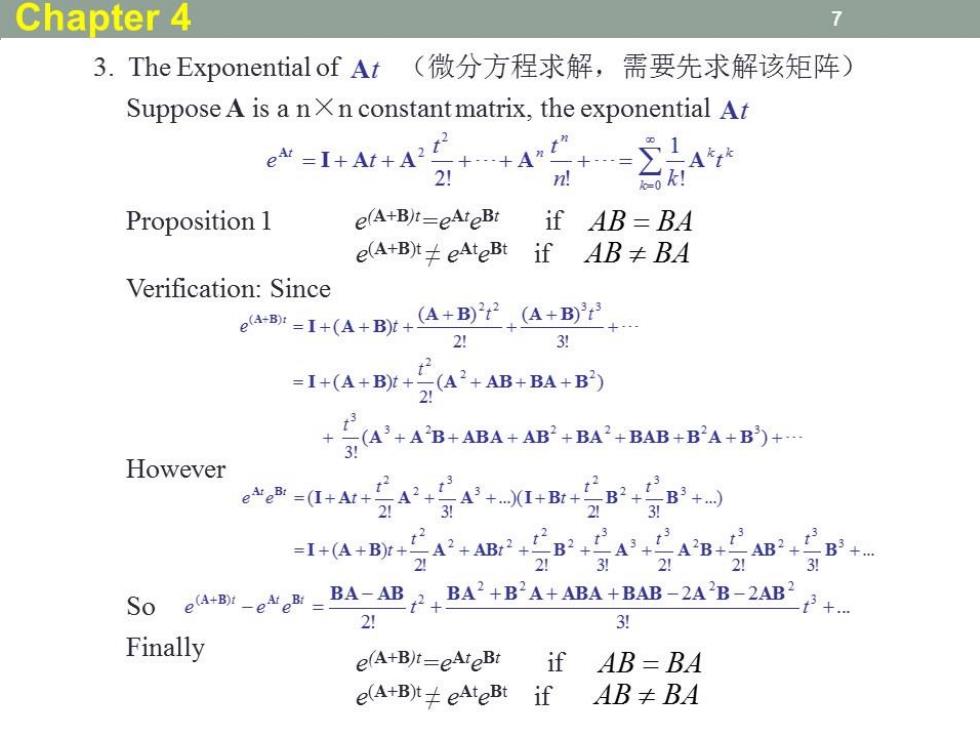
Chapter 4 3.The Exponential of At(微分方程求解,需要先求解该矩阵) Suppose A is a nXn constant matrix,the exponential At e=I+At+A2号 2,++4* Proposition 1 e(A+B)t=eAteBt if AB=BA e(A+Bt≠eAteBt if AB≠BA Verification:Since Q=I+(A+Bg+a+B,A+BP+ 21 31 -I+(A+B 2A'+AB+BA+B码 (A+AB+ABA+AB+BA+BAB+BA+B+ + However ee=I+Ar+ Xa雪 31 =+(A+Br+ 2 3 21 21 31 SO (4+m:e=BA-ABBA2+B2A+ABA+BAB-2A'B-2AB2 3+ Finally e(A+B)t-eAreBt if AB=BA e(a+Bt≠eAteBt if AB≠BA
Chapter 4 6
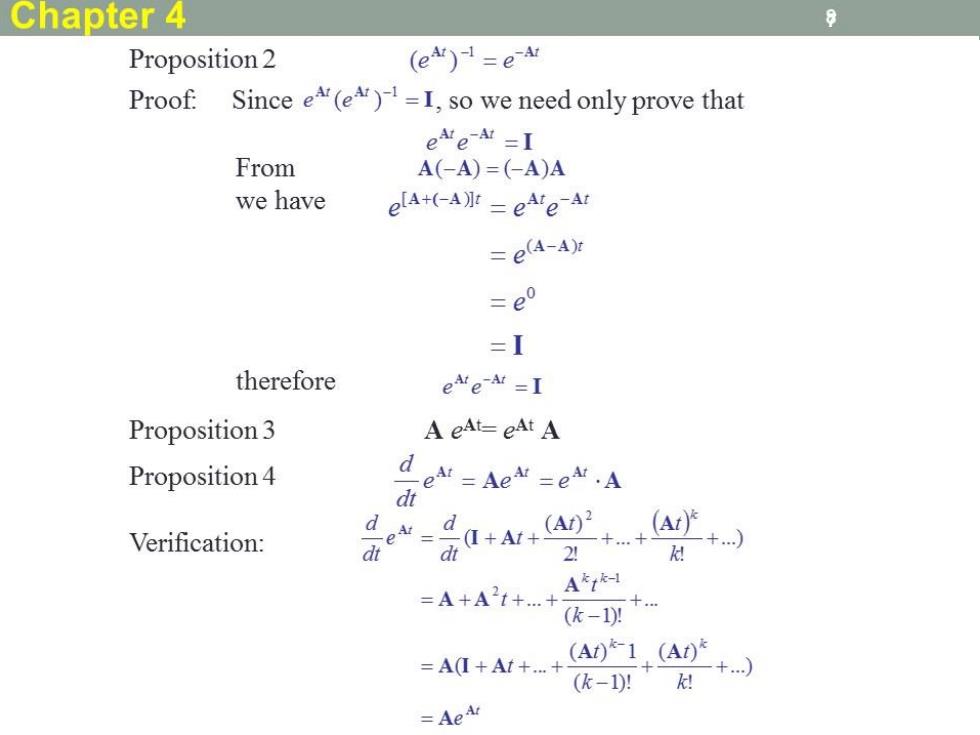
Chapter 4 Proposition 2 (eA)-1=e-A Proof: Since e4(e4)=I,so we need only prove that eNe-A =I From A(-A)=(-A)A we have elA+(-A)eAre-Ar =e(A-4)r =eo =I therefore eMe-Ar=I Proposition3 A eAt=eAt A Proposition4 d -eAr=AeAr =eA.A dt Verification: 0+4+a2 dov-d (A) 21 +) d =A+A2t+.+ A-1 (k-1)! (A)1,(A)* =A(I+At+.+ (k-1)!: + =AeA
Chapter 4 7

Chapter 4 The Fundamental Matrix Consider the linear differential equation system X(t)=AX(t) where X is the initial state vector,X(t)eR",Ae consth(n,n) Multiply both sides by e-Ar eAx()=eAAX(t) According to Proposition 4 eA=-e(←A)=-de dt ew4x(+4ex()=0 dt dt According to the product rule for differentiation of matrix function (ex())=0 dt SO eX(①=C, CERT Vector C is decided by the initial condition: C=e-A X(to) so etx①=e点Xt) Considering e-A=(e4),we have X(t.X)=eNle-Ax(t) This is the solution of system
Chapter 4 8

Chapter 4 10 And we call o(r)=e4'as the fundamental matrix of system. The solution of fundamental matrix has been discussed in chapter 3. Summary1(线性齐次方程的解,无控制输入时的状态方程解,由初始状态 决定) X(t)=AX(t), X(to)=Xo its solution Xt,X)=pt)ekX。 Especially,to=0,we have X(t,X。)=p(t)X。 Summary2(线性状态方程的解,由输入与初始状态共同决定) Consider the linear control system X(t)=AX(t)+BU(t)X(to)=Xo its solution X(t,Xo.U)=eAX+e A(-BU(r)dr
Chapter 4 9
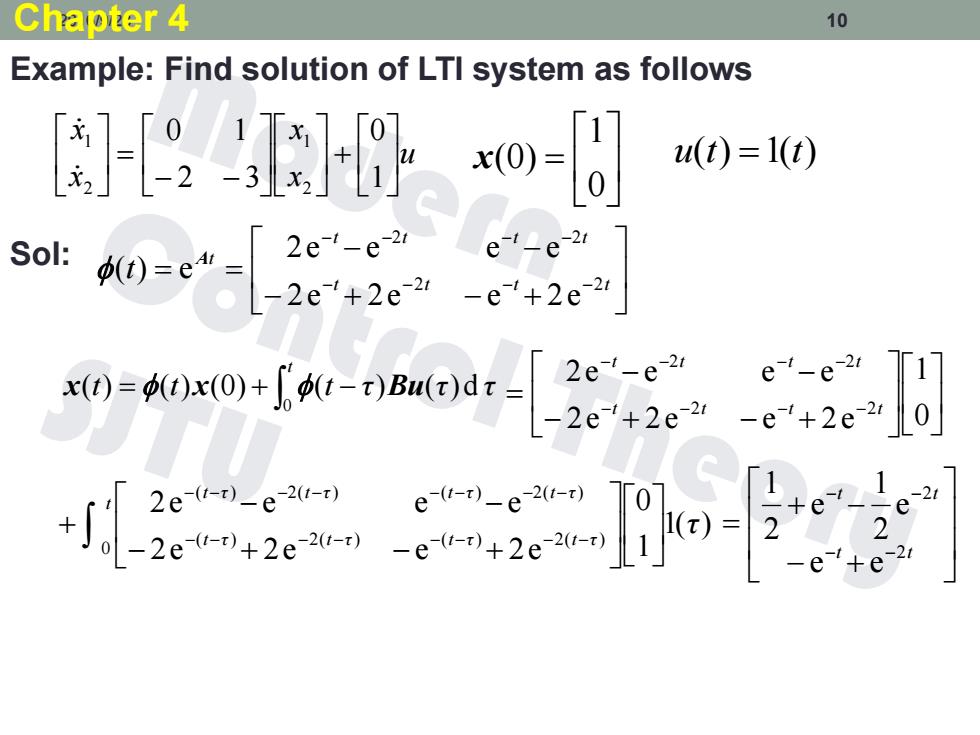
Chapter 4 10 Example:Find solution of LTI system as follows -+0日 u(t)=1(t) e e e-2 w=0a-o-anrgg8aad 月]g
2016/9/23 10 Example: Find solution of LTI system as follows u x x x x 1 0 2 3 0 1 2 1 2 1 0 1 x(0) u(t) 1(t) Sol: t t t t t t t t t t 2 2 2 2 2e 2e e 2e 2e e e e ( ) e A t t t t 2 2 e e e 2 1 e 2 1 1( ) 1 0 2e 2e e 2e 2e e e e 0 ( ) 2( ) ( ) 2( ) ( ) 2( ) ( ) 2( ) τ t t τ t τ t τ t τ t τ t τ t τ t τ t t t τ τ τ t ( ) ( ) (0) ( ) ( )d 0 x x Bu 0 1 2e 2e e 2e 2e e e e 2 2 2 2 t t t t t t t t Chapter 4