上海交通大学：《现代控制理论》课程教学资源（讲稿）Chapter 7 State Feedback and State Estimator

Chapter 7 2 Chapter 7 State Feedback and State Estimator Objectives: State feedback and design of feedback matrix .Design of state estimator Feedback control with estimated states Theory

Chapter 7 State Feedback and State Estimator Objectives: • State feedback and design of feedback matrix • Design of state estimator • Feedback control with estimated states Chapter 7 2

Chapter 7 3 7.1 State Feedback Output feedback control system Desired output response Comparison Controller Process ◆Oulput Measurement Desired (O加lpl oulpul Controller Process variables D Mersurement

7.1 State Feedback • Output feedback control system Chapter 7 3

Chapter 7 4 Output feedback system 文=Ax+Bu Desired oulput response Comparison Controller Process Oulput y=Cx+Du Measurement let u=V-Hy =Ax+B(V-Hy)=[A-BH(I+DH)C]x+[B-BH(I+DH)DWV y=(I+DH)Cx+(I+DH)DV H

Output feedback system 4 let u V  Hy       y Cx Du x Ax Bu x Ax B(V Hy) [A BH(I DH) C]x [B BH(I DH) D]V 1 1           y I DH Cx I DH DV 1 1 ( ) ( )       Chapter 7

Chapter 7 ◇ State feedback system A linear system: =Ax+Bu y=Cx+Du Kx-State feedback Let:u=V-Kc→ -input of system u-output of controller/input of plant Then =Ax+B(V-Kx)=(A-BK)x+BV y=(C-DK)x+DV

State feedback system • A linear system: • Then 5        y Cx Du x Ax Bu Let: u V  Kx y  (C  DK)x  DV x   Ax  B(V  Kx)  (A BK)x  BV  Kx - State feedback V – input of system u – output of controller/input of plant Chapter 7

Chapter 7 6 7.2 Controllability of Feedback system Linear system: 文=Ax+BM y=Cx State feedback Feedback system=(A-BKx+Br y=Cx Theorem:The system(A-BK,B)is controllable IFF system (A,B)is controllable. Note:The observability may be changed

7.2 Controllability of Feedback system • Theorem: The system (A-BK, B) is controllable IFF system (A,B) is controllable. • Note: The observability may be changed. 6 Linear system:       y Cx x Ax Bu State feedback         n i n ki xi u r Kx r- k k x r 1 1         y Cx Feedback system x (A BK)x Br Chapter 7

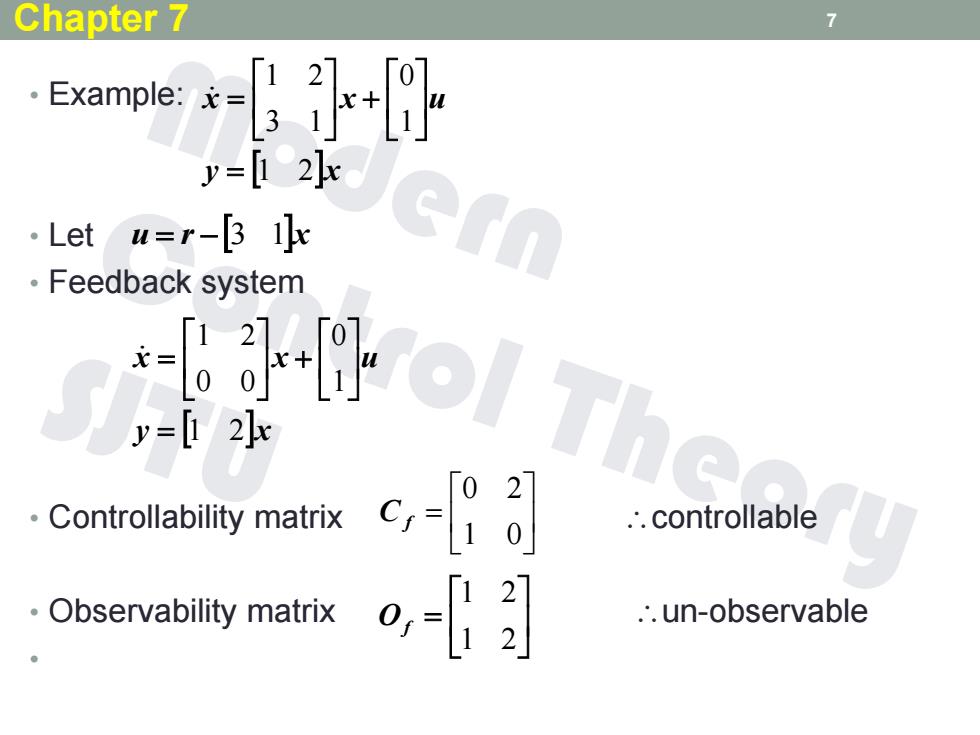
Chapter 7 7 「12].「01 Example:;xgk+ y=[2k .Let u=r-[3 Ik ol The ·Feedback system y=1/2]x Controllability matrix c .∴controllable Observability matrix [121 012 ..un-observable

• Example: • Let • Feedback system • Controllability matrix controllable • Observability matrix un-observable • 7 y  x x x u 1 2 1 0 3 1 1 2                 u  r 3 1x y  x x x u 1 2 1 0 0 0 1 2                        1 0 0 2 C f        1 2 1 2 Of Chapter 7

Chapter 7 8 7.3 Pole placement and design of feedback matrix State feedback can be used to place eigenvalues(pole)of A in any position if the system is controllable. Pole placement:choosing state feedback gains to place poles in desired positions. Theorem:If the n-dimensional linear system is controllable,then the eigenvalues of 4-BK can arbitrarily assigned by state feedback u=r-Kx,where k is a 1xn real constant vector

7.3 Pole placement and design of feedback matrix • State feedback can be used to place eigenvalues(pole)of A in any position if the system is controllable. • Pole placement: choosing state feedback gains to place poles in desired positions. • Theorem: If the n-dimensional linear system is controllable, then the eigenvalues of A-BK can arbitrarily assigned by state feedback u=r-Kx, where k is a 1n real constant vector. Chapter 7 8

Chapter 7 9 Theorem:Consider the state equation x=Ax+bu,y =cx with n =4 and the characteristic polynomiai △(s)=det(l-A)=s4+4s3+a2s2+4s+&4: If the given state equation is controllable,then it can be transformed by the transformation x=Px with 10%23 Q=P-=[b Ab A2b Ab] 0112 0014 into the controllable canonical form 0001 [-%1-2-%3-4 T1 1 00 0 0 文=Ax+bM= 区+ 01 0 0 0 u.y=Cx=IB B2 B Bx. 001 0 0

Chapter 7 9

Chapter 7 10 Remark:For the case of n=4,from any set of desired eigenvalues,we can readily form Let △(S)=S4+瓦S3+a2s2+as+瓦4 k=[a1-41a2-2a3-a3a4-4l,k=kP where [1%a2% P-=[b Ab AbAb] 01%02 00101 0001 cory

Chapter 7 10

Chapter 7 11 Example: Consider a linear system -6* A(S)=(s-1)2-9=(s-4)(s+2),→S=4,S2=-2 State feedback 店+-[母 4(s)=s2+(k-2)5+(3k-k2-8) New s,s2 is determined by values of k1 and k2

Example: • Consider a linear system 11 x x u              0 1 3 1 1 3  2 1 2            ( ) ( 1) 9 ( 4)( 2), 4, 2 s s s s s s x r k k x u k k x                                             0 1 3 1 1 3 0 1 3 1 0 0 1 3 1 2 1 2  ( s ) s ( k 2 )s ( 3k k 8 ) 1 1 2 2        State feedback         n i n ki xi u r Kx V- k k x r 1 1  New s1 s2 is determined by values of k1 and k2 . Chapter 7