Chapter 6 Electronic Structure of Atoms 6.4 The Wave Behavior of Matter 6.5 Quantum Mechanical Atomic Orbitals 6.6 Representations of Orbitals 6.7 Many electron atoms 6.8 Electron Configurations 6.9 Electron Configurations Periodic Table Chapter 6
Chapter 6 Electronic Structure of Atoms 6.4 The Wave Behavior of Matter Chapter 6 6.5 Quantum Mechanical & Atomic Orbitals 6.6 Representations of Orbitals 6.7 Many electron atoms 6.8 Electron Configurations 6.9 Electron Configurations & Periodic Table

The Wave Nature of Light Wave properties of light Wavelength A Amplitude kcue c=A.v Wide gop-small efec 山》 衍射
The Wave Nature of Light Wave properties of light : Wavelength λ Frequency ν diffraction c = ⋅ λ ν 衍射
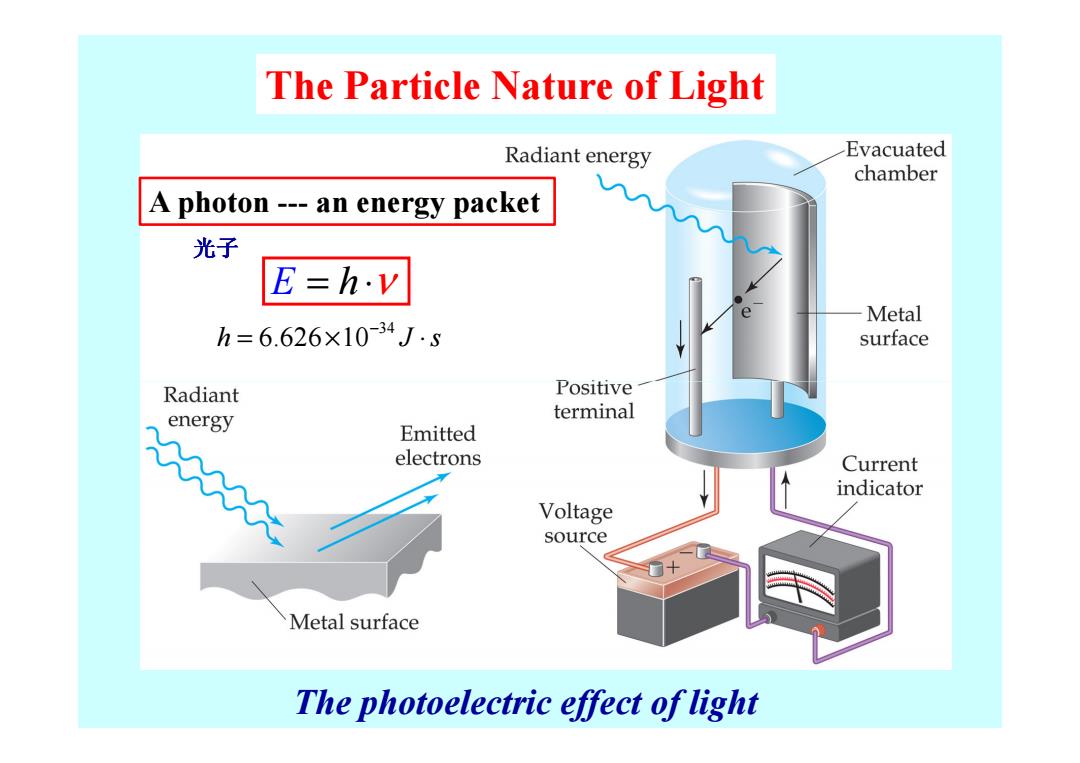
The Particle Nature of Light Radiant energy Evacuated chamber A photon---an energy packet 光子 E=h.v Metal h=6.626×10-34J-s surface Radiant Positive energy terminal Emitted electrons Current indicator Voltage source Metal surface The photoelectric effect of light
The Particle Nature of Light E = ⋅ h ν A photon --- an energy packet 光子 34 h J s 6.626 10 − = × ⋅ The photoelectric effect of light

Duality of Light A photon--- an energy packet Wavelength E hoton =h.v h=6.626×10-34Js =A.h.v 乏diffraction≤ A=6.02×1023/mol The Particle The Wave Nature of Light Nature of Light
Duality of Light Wavelength λ Frequency ν A photon --- an energy packet Ephoton = ⋅ h ν 34 h J s 6.626 10− = × ⋅ The Wave Nature of Light The Particle Nature of Light diffraction ν mole E = ⋅ ⋅ A h ν 23 A mol = × 6.02 10 / 34 h J s 6.626 10− = × ⋅

The visible light---electromagnetic radiation 电磁辐射 The electromagnetic spectrum 电磁波谱 1020 1018 1016 104 1012 1010 10 105 104 Frequency (Hz) UV IR Gamma X rays Ultra- Infrared Microwave Radio waves rays violet Type of radiation L Microwave ovel UHF TV, FM radio. AM 3.0×108m/s police radar. VHF TV V=c/A= =5.75x10-14s-satellite stations cellular radio elephones 522nm×(10-°m/nm) E=hy=6.626×10-34J·s×5.75×1014s1 Emole=A.h-v Photon =6.02×1023×6.626×10-34Js×5.75×104s =3.808×10-20J =2.294×103J=229.4kJ E=h.v 400nm 500 600 700
The electromagnetic spectrum The visible light --- electromagnetic radiation 电磁辐射 电磁波谱 UV IR E = ⋅ h ν Photon 34 14 1 20 6.626 10 5.75 10 3.808 10 E h s J s J ν − − − = ⋅ × × × = × = ⋅ 23 34 14 1 5 6.02 10 6.626 10 5.75 10 2.294 10 229.4 Emole J A s kJ s J h ν − − = ⋅ ⋅ = × × × ⋅ × × = × = 8 14 1 9 (1 3.0 10 / 5.75 10 522 0 / ) m s c n m n s m m ν λ − − × − = = = × ×

6.4 Particle-Wave Duality Is light a wave or a particle? Light's wave characteristics-- electromagnetic radiation Light's particle characteristics- based on Einstein's interpretation of the photoelectric effect. E=h.v Particle-wave duality: “photons' It's both a wave and a particle!
6.4 Particle 6.4 Particle-Wave Duality Wave Duality • Light’s wave characteristics -- electromagnetic radiation • Light’s particle characteristics– based on Einstein’s interpretation of the photoelectric effect. Particle Particle-wave duality wave duality : “photons” It’s both a wave and a particle! E = ⋅ h ν
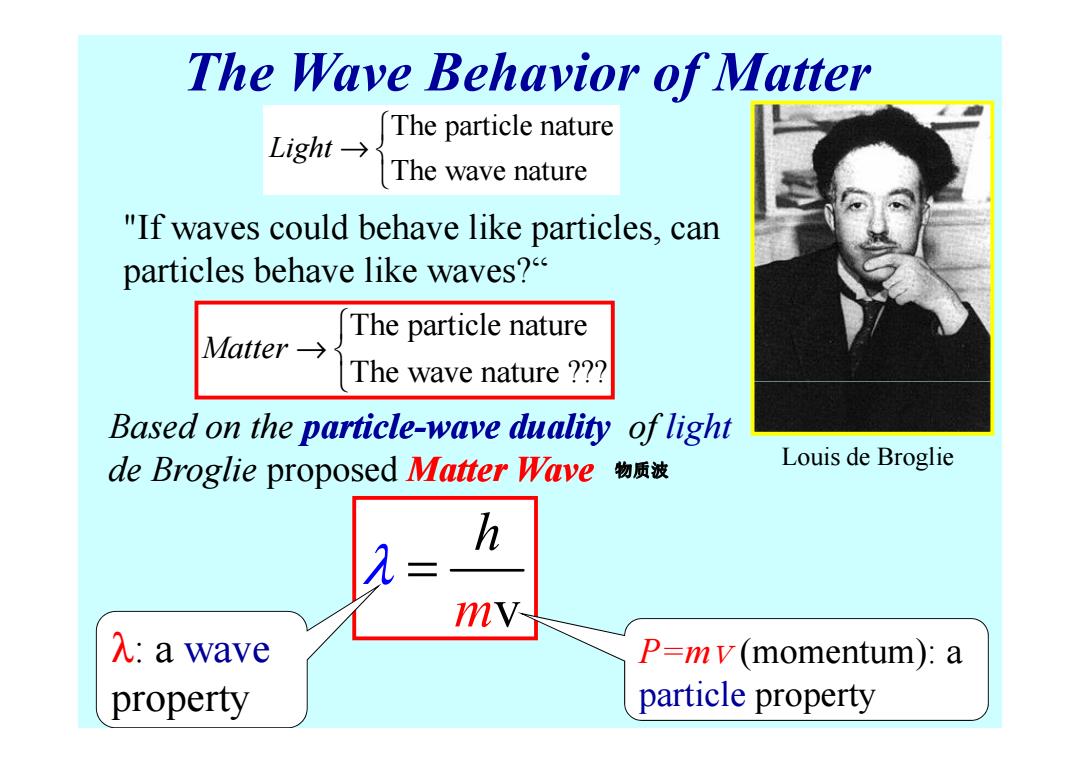
The Wave Behavior of Matter The particle nature Light→ The wave nature "If waves could behave like particles,can particles behave like waves? The particle nature Aatter→s The wave nature ?? Based on the particle-wave duality of light de Broglie proposed Matter Wave物质被 Louis de Broglie h mV- λ:a wave P=mv(momentum):a property particle property
The Wave Behavior of Matter The particle nature The wave nature Light → The particle nature The wave nature ??? Matter → "If waves could behave like particles, can particles behave like waves?“ Based on the particle particle-wave duality wave duality of light de Broglie proposed Matter Wave The wave nature ??? m v h λ = P=m v (momentum): a particle property λ: a wave property Louis de Broglie 物质波

The Wave Behavior of Matter de Broglie wave: h The wavelength(A)of a particle depends on its mass (m)and velocity (v). mV Ex.1:Calculate the wavelength of a tennis ball of mass 200g traveling at a velocity of 25 m/s. h 6.626×10-34Js = mv (0.2kg)×(25m/s) 6.626×10-34(kg·m2.s2)s (0.2kg)×(25m·s) 元=1.3×10-34m Undetectable small
The Wave Behavior of Matter Ex. 1: Calculate the wavelength of a tennis ball of mass 200g traveling at a velocity of 25 m/s. m v h λ = de Broglie wave: The wavelength(λ) of a particle depends on its mass (m) and velocity (v). traveling at a velocity of 25 m/s. 34 6.626 10 v (0.2 ) (25 / ) h s J m kg m s λ − × ⋅ = = × 1 34 2 2 6.626 10 ( ) (0.2 ) (25 ) s kg m s kg m s λ − − − × ⋅ = × ⋅ ⋅ ⋅ 34 λ 1.3 10 m − = × Undetectable small

Electromagnetic Spectrum Gamma Ray X-Ray Ultraviolet Visible Infrared Microwave Radio Wave Type WWAA/ Gamma Ultraviolet Infrared Rays X-Rays Rays Rays Radar FM V Shortwave AM 1x1014 1×1012 1x10-8 1x104 1×102 1×102 1x104 Wavelength(in meters) m 元=1.3×10-34m Visible Light 400nm 500nm 600nm 700nm Wavelength
Electromagnetic Spectrum m 34 λ 1.3 10 m − = ×
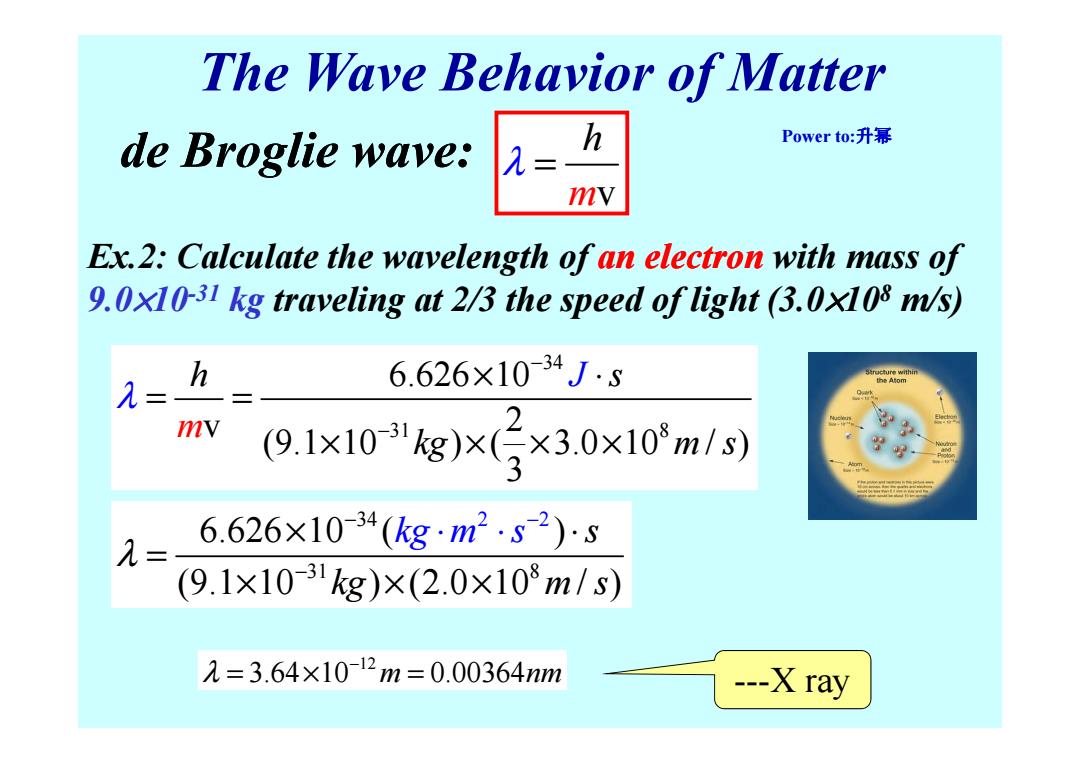
The Wave Behavior of Matter de Broglie wave: h Power to:升幂 = mv Ex.2:Calculate the wavelength of an electron with mass of 9.0x10-31 kg traveling at 2/3 the speed of light (3.0x108 m/s) h 6.626×10-34J5 λ= mv (9.1x10kg)x3×3.0×10m/s) 6.626×10-34(kgm2.82)s (9.1×1031kg)×(2.0×108m/s) 2=3.64×10-12m=0.00364m ---X ray
The Wave Behavior of Matter Ex.2: Calculate the wavelength of an electron with mass of 9.0×10-31 kg traveling at 2/3 the speed of light (3.0×10 8 m/s) m v h de Broglie wave: λ = 34 h s 6.626 10 J λ − × ⋅ = = Power to:升幂 31 8 6.626 10 v 2 (9.1 10 ) ( 3.0 10 / ) 3 h s kg J m m s λ − × ⋅ = = × × × × 34 3 2 2 1 8 6.626 10 ( ) (9.1 10 ) (2.0 10 / ) s kg m m s s kg λ − − − × ⋅ = × × × ⋅ ⋅ 12 λ 3.64 10 0.00364 m nm − = × = ---X ray