
Chapter Six Intergration
Chapter Six Intergration

The Area Problem Given a function fthat is continuous and nonnegative on an interval [a,b],find the area between the graph of f and the interval [a,b]on the x-axis
The Area Problem ⚫Given a function f that is continuous and nonnegative on an interval [a,b], find the area between the graph of f and the interval [a,b] on the x-axis

Antiderivative ●Definition A function F is called an antiderivative of a functionf on a given interval Iif F(x)=fx) for all x in the interval
Antiderivative ⚫Definition A function F is called an antiderivative of a function f on a given interval I if F’(x) =f(x) for all x in the interval
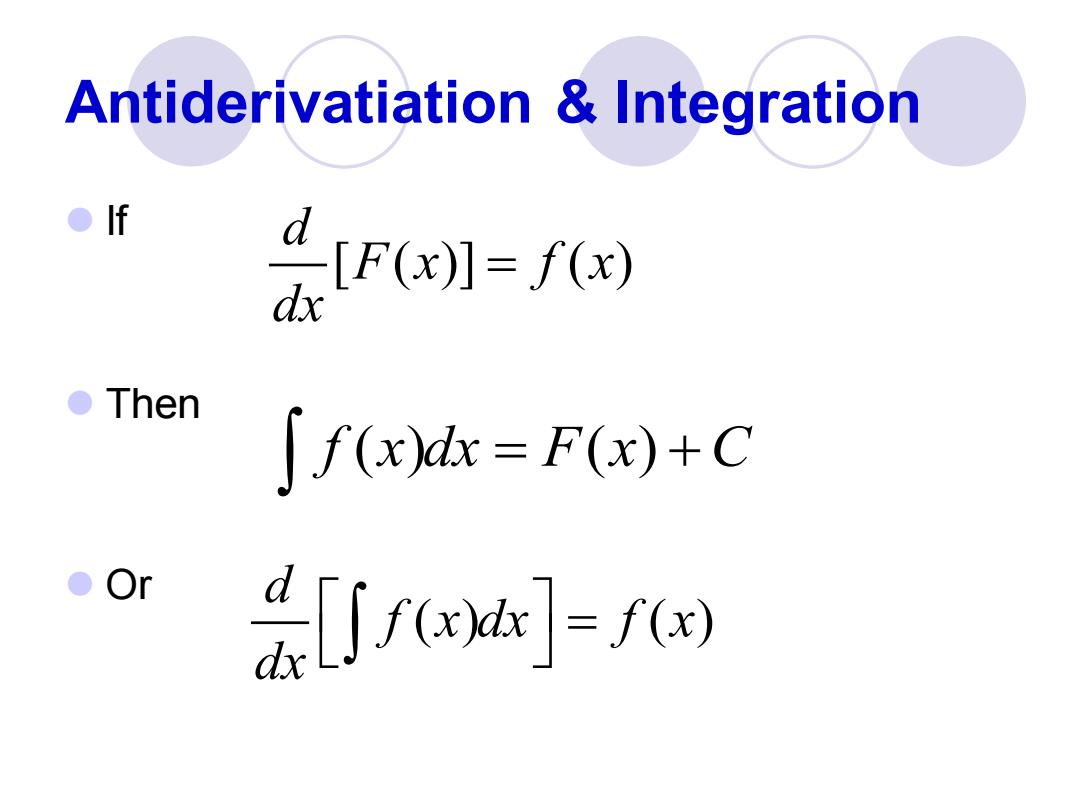
Antiderivatiation Integration ●f 2r0划0网 Then ∫f(x)dx=F(x)+C ●O ]-fo
Antiderivatiation & Integration ⚫ If ⚫ Then ⚫ Or [ ( )] ( ) d F x f x dx = f x dx F x C ( ) ( ) = + ( ) ( ) d f x dx f x dx =
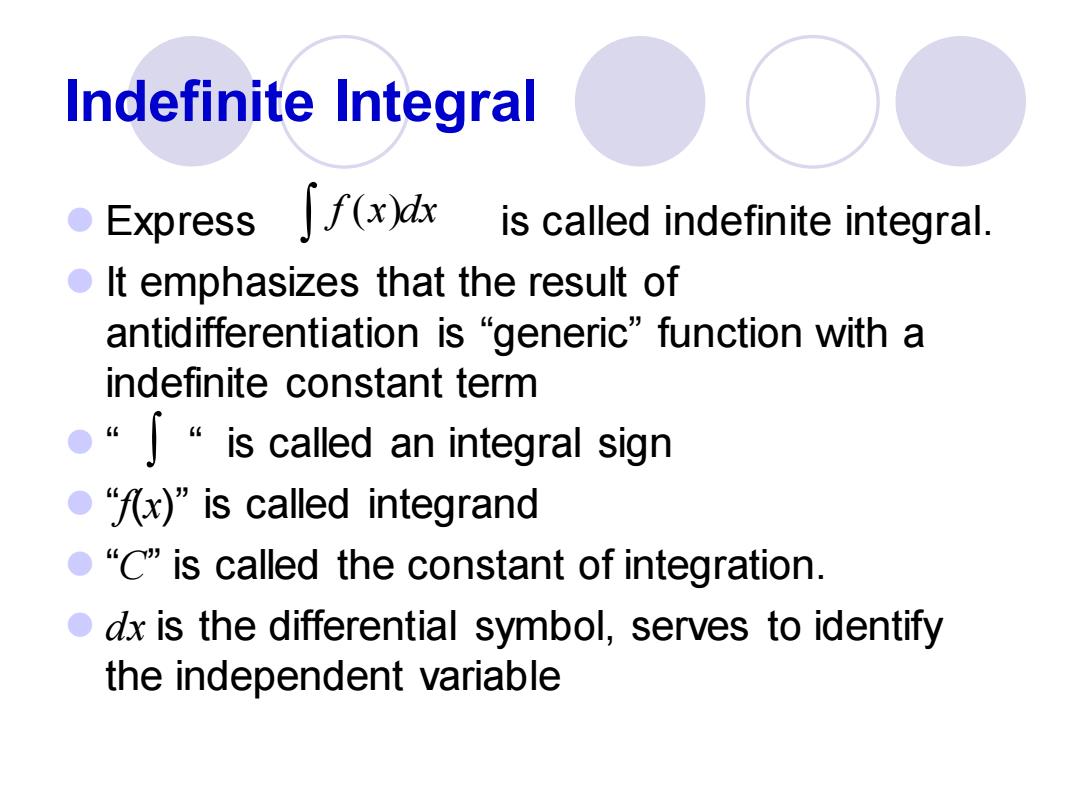
Indefinite Integral Express∫f(x)k is called indefinite integral. It emphasizes that the result of antidifferentiation is "generic"function with a indefinite constant term ●“J“is called an integral sign ●“fx)”is called integrand ●“C"is called the constant of integration. odx is the differential symbol,serves to identify the independent variable
Indefinite Integral ⚫ Express is called indefinite integral. ⚫ It emphasizes that the result of antidifferentiation is “generic” function with a indefinite constant term ⚫ “ “ is called an integral sign ⚫ “f(x)” is called integrand ⚫ “C” is called the constant of integration. ⚫ dx is the differential symbol, serves to identify the independent variable f x dx ( )
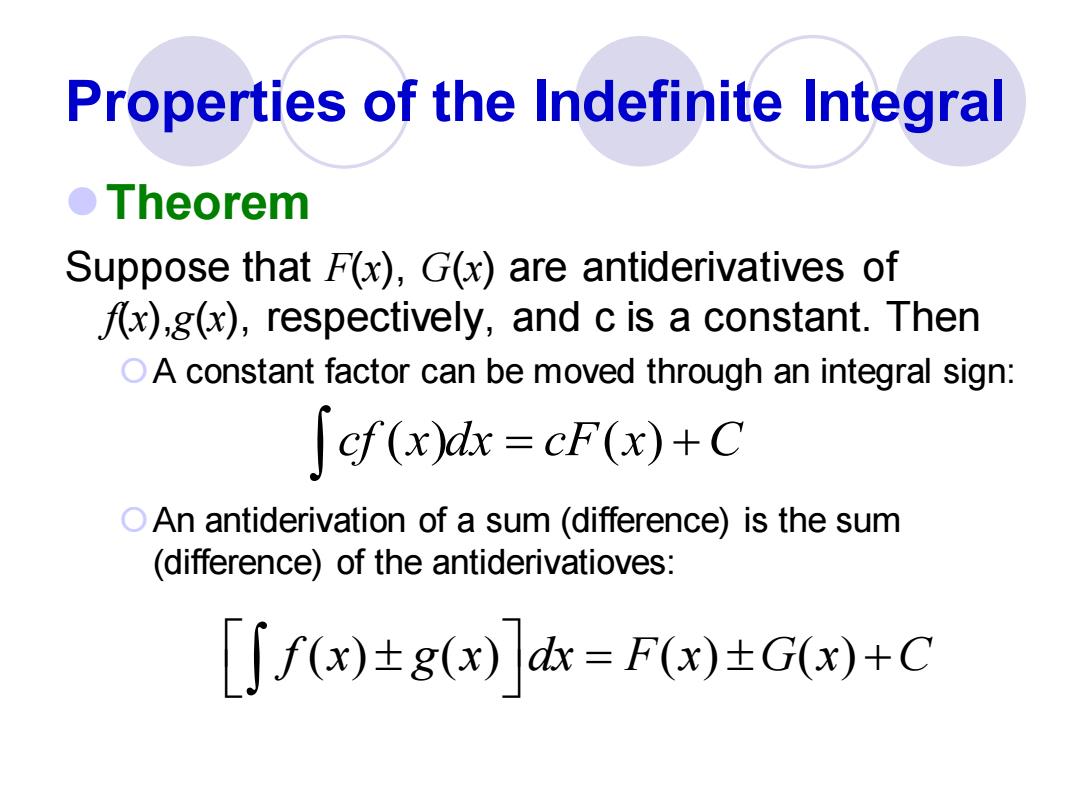
Properties of the Indefinite Integral ●Theorem Suppose that F(x),G(x)are antiderivatives of fx),g(x),respectively,and c is a constant.Then OA constant factor can be moved through an integral sign: ∫cf(xdx=cF(x)+C OAn antiderivation of a sum(difference)is the sum (difference)of the antiderivatioves: [Jf(x)±g(O)]=F(x)士G()+C
Properties of the Indefinite Integral ⚫Theorem Suppose that F(x), G(x) are antiderivatives of f(x),g(x), respectively, and c is a constant. Then A constant factor can be moved through an integral sign: An antiderivation of a sum (difference) is the sum (difference) of the antiderivatioves: cf x dx cF x C ( ) ( ) = + f x g x dx F x G x C ( ) ( ) ( ) ( ) = +
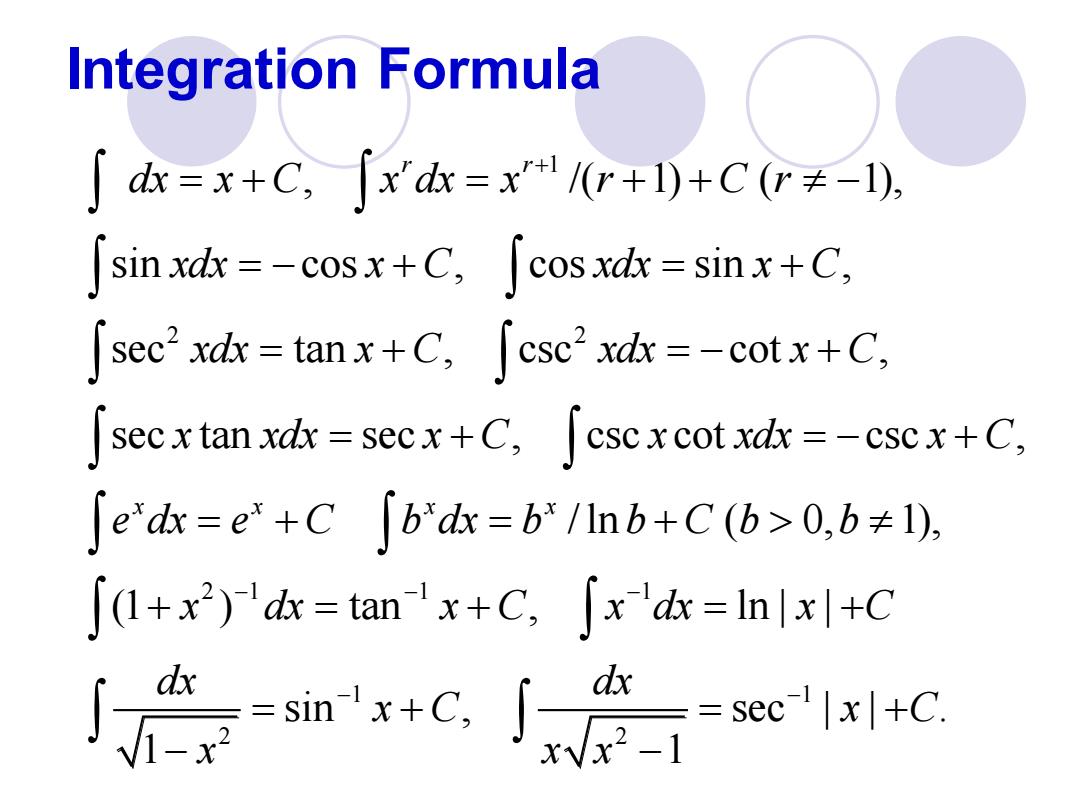
Integration Formula ∫c=x+C,∫xd=x+)+C≠-1)。 sin xdx=-cosx+C,[cos xdx=sinx+C, sec2xdx=tanx+C,[csc2 xdx =-cotx+C, secxtan xdx secx+C,cscx cotxdx =-cscx+C, edx=e+Cb'dx=b/lnb+C(b>0,b≠1), ∫1+x2)'dx=tan'x+C,「xdk=lnlx+C dx
Integration Formula 1 2 2 2 1 1 1 , /( 1) ( 1), sin cos , cos sin , sec tan , csc cot , sec tan sec , csc cot csc , / ln ( 0, 1), (1 ) tan , ln r r x x x x dx x C x dx x r C r xdx x C xdx x C xdx x C xdx x C x xdx x C x xdx x C e dx e C b dx b b C b b x dx x C x dx + − − − = + = + + − = − + = + = + = − + = + = − + = + = + + = + = 1 1 2 2 | | sin , sec | | . 1 1 x C dx dx x C x C x x x − − + = + = + − −
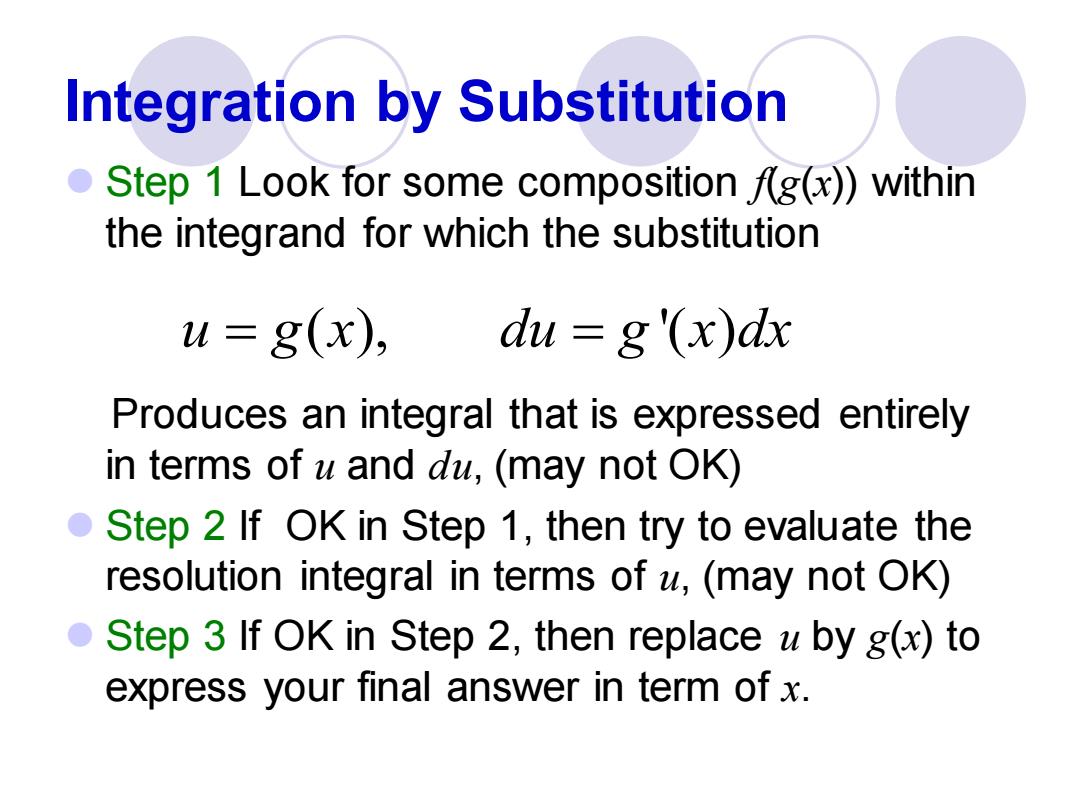
Integration by Substitution ● Step 1 Look for some composition fg(x))within the integrand for which the substitution u=g(x), du =g'(x)dx Produces an integral that is expressed entirely in terms of u and du,(may not OK) Step 2 If OK in Step 1,then try to evaluate the resolution integral in terms of u,(may not OK) ● Step 3 If OK in Step 2,then replace u by g(x)to express your final answer in term of x
Integration by Substitution ⚫ Step 1 Look for some composition f(g(x)) within the integrand for which the substitution Produces an integral that is expressed entirely in terms of u and du, (may not OK) ⚫ Step 2 If OK in Step 1, then try to evaluate the resolution integral in terms of u, (may not OK) ⚫ Step 3 If OK in Step 2, then replace u by g(x) to express your final answer in term of x. u g x du g x dx = = ( ), '( )
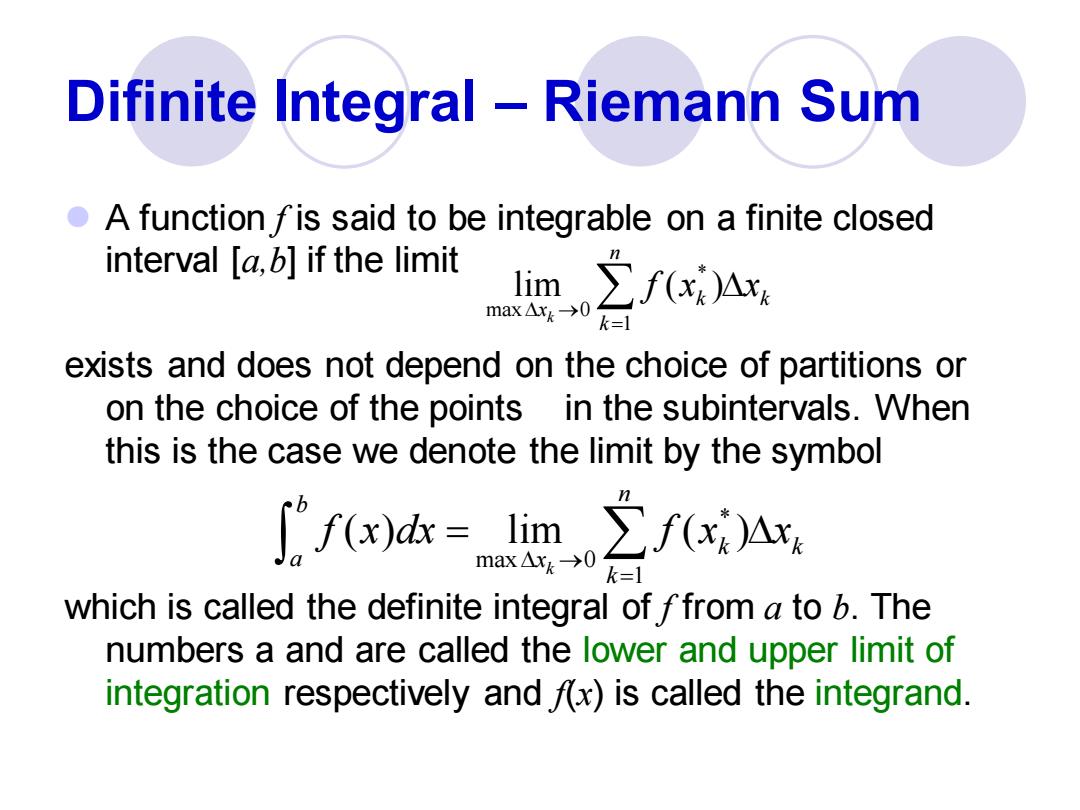
Difinite Integral -Riemann Sum A function fis said to be integrable on a finite closed interval [.if the limit limx max△xk→0 k=1 exists and does not depend on the choice of partitions or on the choice of the points in the subintervals.When this is the case we denote the limit by the symbol fx)dk=Iim,∑fx)Ax nax△xk→0 k=1 which is called the definite integral of ffrom a to b.The numbers a and are called the lower and upper limit of integration respectively andfx)is called the integrand
Difinite Integral – Riemann Sum ⚫ A function f is said to be integrable on a finite closed interval [a,b] if the limit exists and does not depend on the choice of partitions or on the choice of the points in the subintervals. When this is the case we denote the limit by the symbol which is called the definite integral of f from a to b. The numbers a and are called the lower and upper limit of integration respectively and f(x) is called the integrand. * max 0 1 lim ( ) k n k k x k f x x → = * max 0 1 ( ) lim ( ) k n b k k a x k f x dx f x x → = =
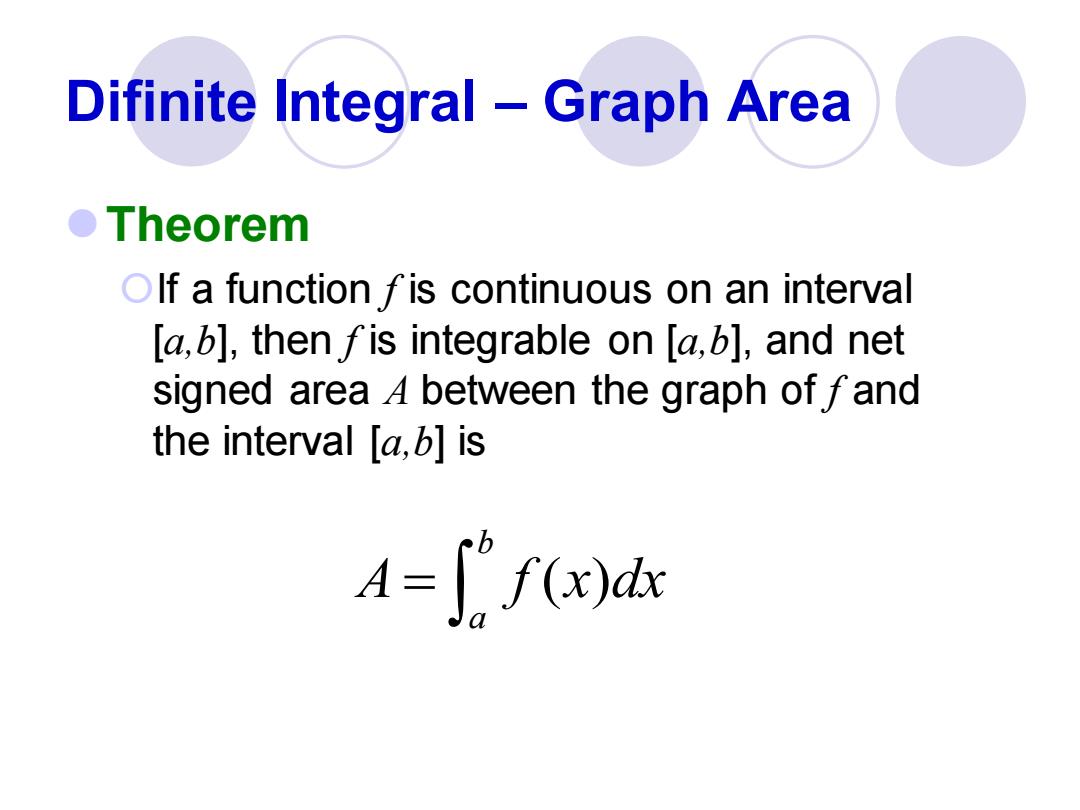
Difinite Integral-Graph Area ●Theorem OIf a function fis continuous on an interval [a,b],then fis integrable on [a,b],and net signed area 4 between the graph of fand the interval [a,b]is A=心((x)dz
Difinite Integral – Graph Area ⚫Theorem If a function f is continuous on an interval [a,b], then f is integrable on [a,b], and net signed area A between the graph of f and the interval [a,b] is ( ) b a A f x dx =