
Chapter Three The Derivatives
Chapter Three The Derivatives

Definition of The Derivative Function The function f defined by the formula f(x)=lim I(x+h)-f(x) h-→0 h which is called the derivative of fwith respect to x. The domain of f consists of all x in the domain of ffor which the limit exists
Definition of The Derivative Function ⚫ The function f’ defined by the formula which is called the derivative of f with respect to x. The domain of f’ consists of all x in the domain of f for which the limit exists. 0 ( ) ( ) '( ) limh f x h f x f x → h + − =
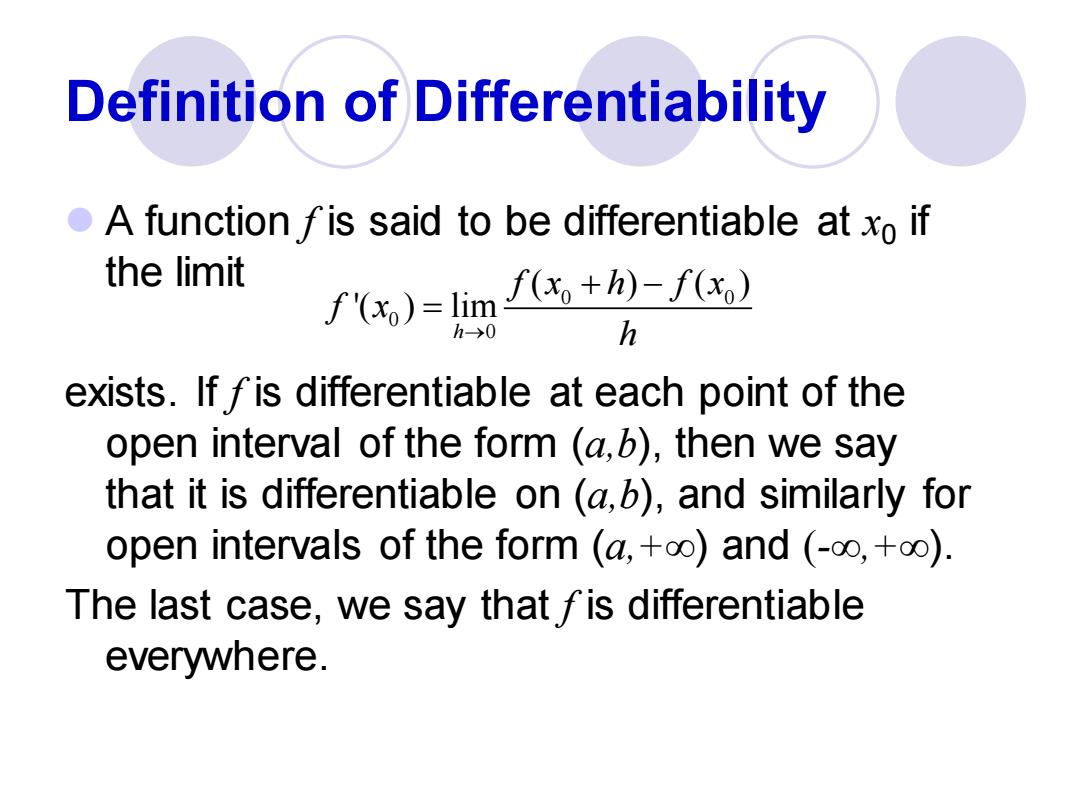
Definition of Differentiability A function fis said to be differentiable at xo if the limit f()=limh-f(x) h-→0 h exists.If fis differentiable at each point of the open interval of the form (a,b),then we say that it is differentiable on (a,b),and similarly for open intervals of the form (a,+oo)and (o,+o). The last case,we say that fis differentiable everywhere
Definition of Differentiability ⚫ A function f is said to be differentiable at x0 if the limit exists. If f is differentiable at each point of the open interval of the form (a,b), then we say that it is differentiable on (a,b), and similarly for open intervals of the form (a,+∞) and (-∞,+∞). The last case, we say that f is differentiable everywhere. 0 0 0 0 ( ) ( ) '( ) limh f x h f x f x → h + − =

Differentiable Point VS Continuous Point ●Theorem If a function fis differentiable at xo,then fis continuous at xo
Differentiable Point VS Continuous Point ⚫Theorem If a function f is differentiable at x0 , then f is continuous at x0

One-sided Derivative Left-hand derivatives L()=lim-f(xo) h>0 h Right-hand derivatives f.()=lim f(h)-f(xo) h>0 h
One-sided Derivative ⚫ Left-hand derivatives ⚫ Right-hand derivatives 0 0 0 0 ( ) ( ) '( ) lim h f x h f x f x h − → − + − = 0 0 0 0 ( ) ( ) '( ) lim h f x h f x f x h + → + + − =
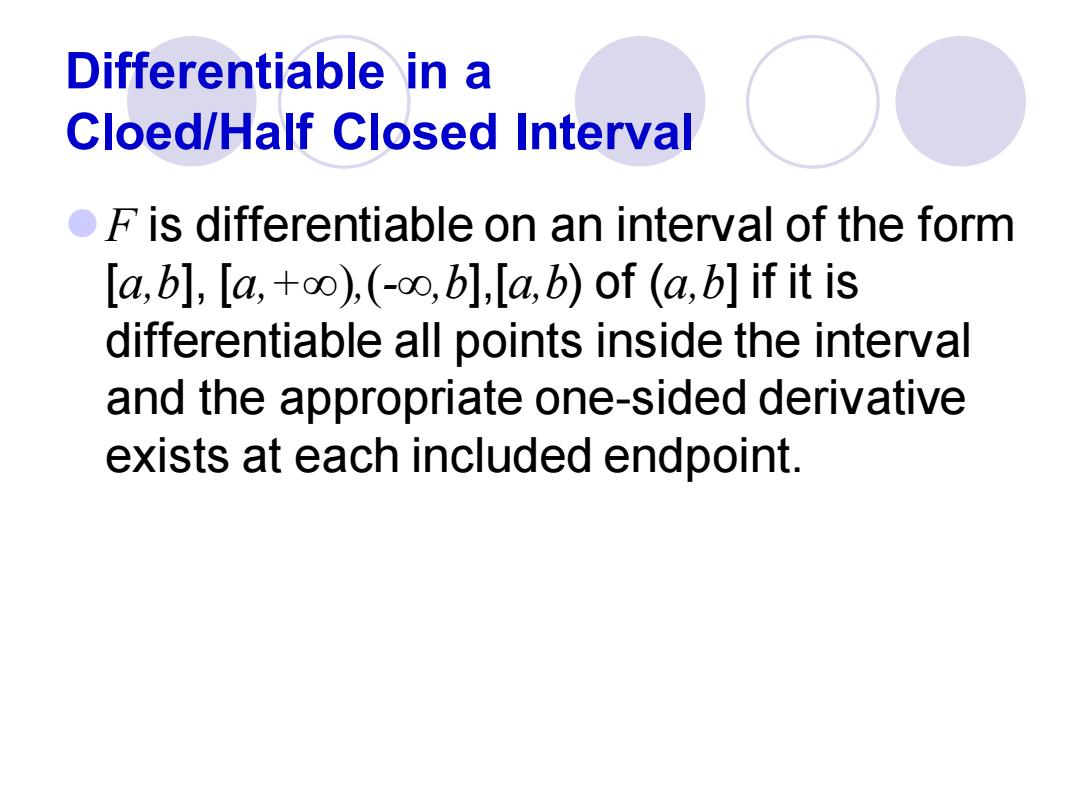
Differentiable in a Cloed/Half Closed Interval OF is differentiable on an interval of the form [a,b],[a,+),(-0,b],la,b)of (a,b]if it is differentiable all points inside the interval and the appropriate one-sided derivative exists at each included endpoint
Differentiable in a Cloed/Half Closed Interval ⚫F is differentiable on an interval of the form [a,b], [a,+∞),(-∞,b],[a,b) of (a,b] if it is differentiable all points inside the interval and the appropriate one-sided derivative exists at each included endpoint
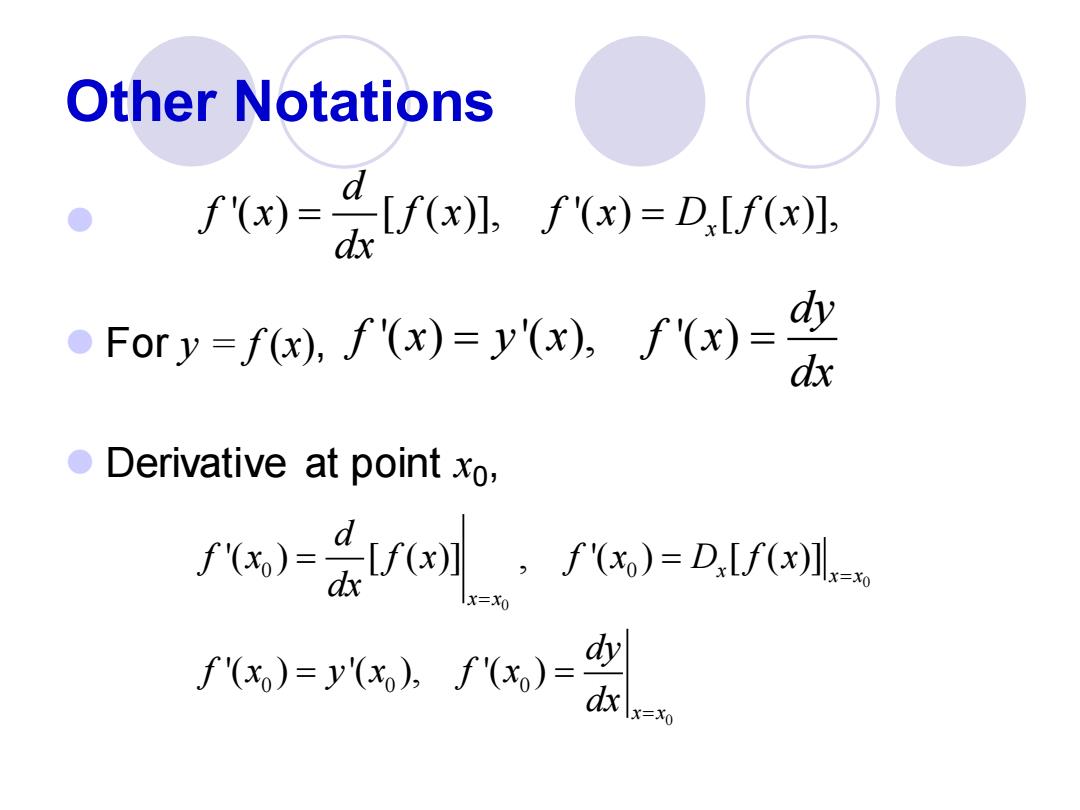
Other Notations f)-么[rlf=o[un Fory=f(x),f(x)=y(x),f(x)= dy dx Derivative at point xo, x)4Vw·x)=pUe ()-y(x).)=
Other Notations ⚫ ⚫ For y = f (x), ⚫ Derivative at point x0 , '( ) [ ( )], '( ) [ ( )], x d f x f x f x D f x dx = = '( ) '( ), '( ) dy f x y x f x dx = = 0 0 0 0 0 0 0 0 '( ) [ ( )] , '( ) [ ( )] '( ) '( ), '( ) x x x x x x x d f x f x f x D f x dx dy f x y x f x dx = = = = = = =
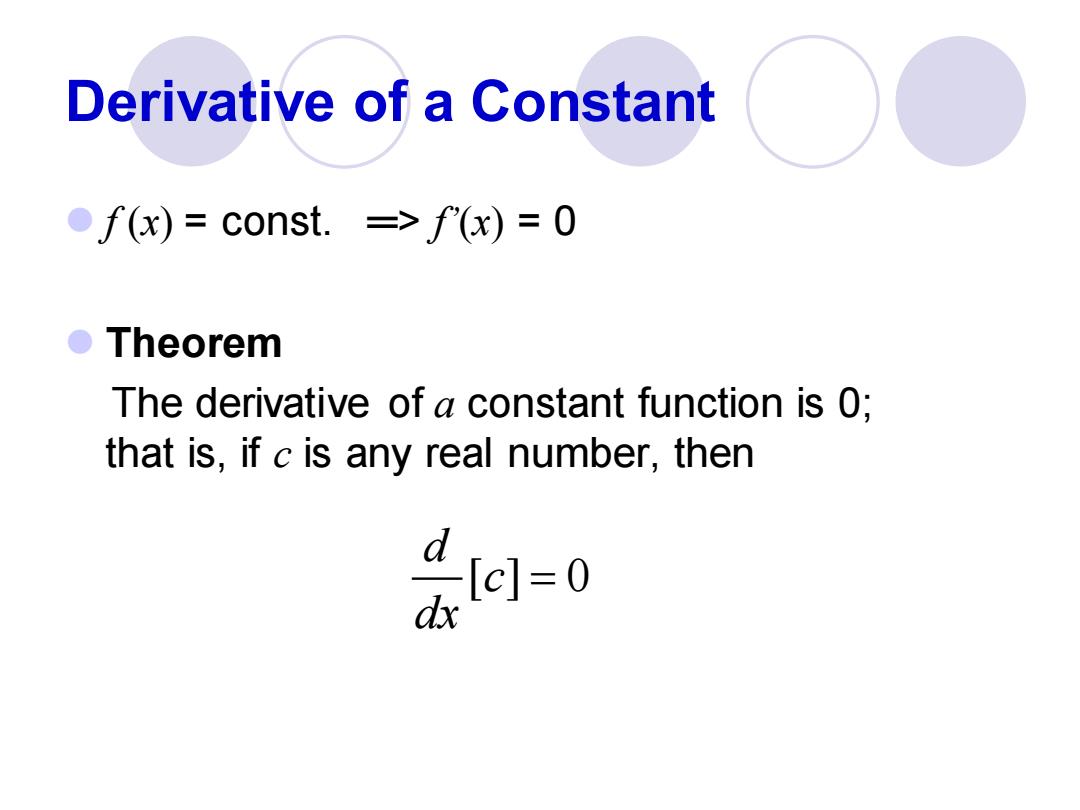
Derivative of a Constant f(x)=const.=>f(x)=0 ●Theorem The derivative of a constant function is 0; that is,if c is any real number,then 0
Derivative of a Constant ⚫ f (x) = const. ═> f’(x) = 0 ⚫ Theorem The derivative of a constant function is 0; that is, if c is any real number, then [ ] 0 d c dx =

Derivatives of Integer Powers of x Theorem (The Power Rule) If n is any integer,then 层Tm
Derivatives of Integer Powers of x ⚫ Theorem (The Power Rule) If n is any integer, then 1 [ ] d n n x nx dx − =
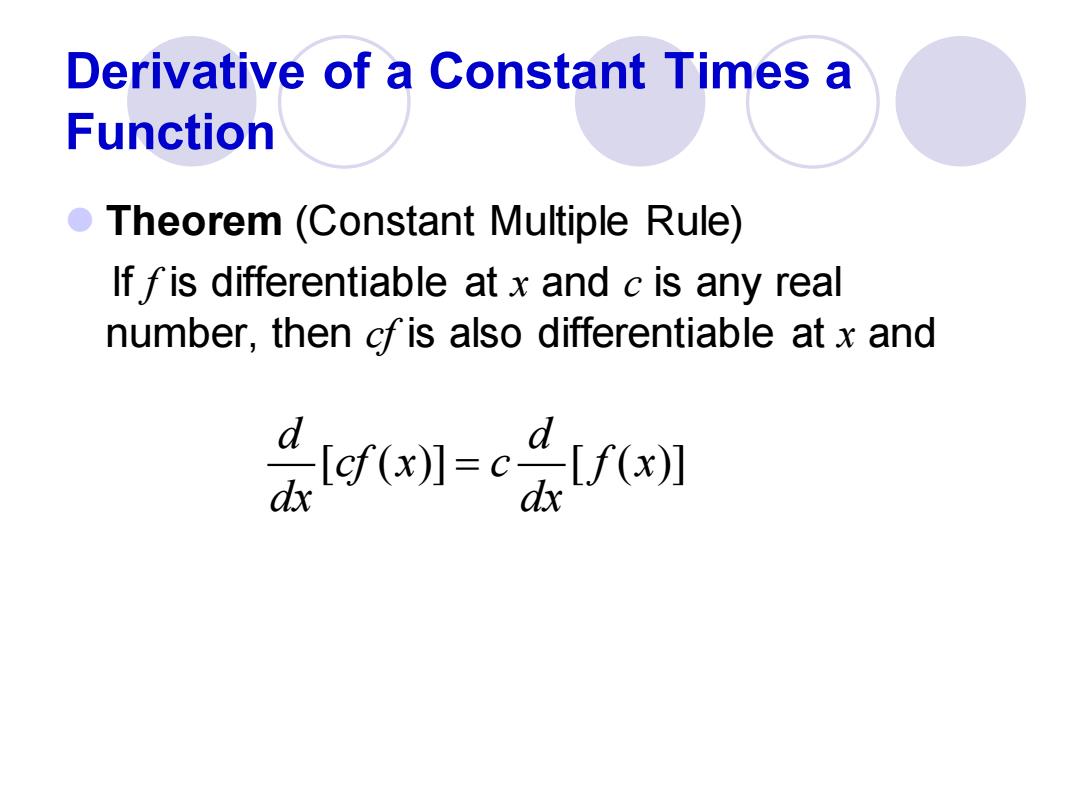
Derivative of a Constant Times a Function Theorem (Constant Multiple Rule) If fis differentiable at x and c is any real number,then cfis also differentiable at x and fw=c安e
Derivative of a Constant Times a Function ⚫ Theorem (Constant Multiple Rule) If f is differentiable at x and c is any real number, then cf is also differentiable at x and [ ( )] [ ( )] d d cf x c f x dx dx =