
Chapter Four The Derivatives in Graphing and Application
Chapter Four The Derivatives in Graphing and Application
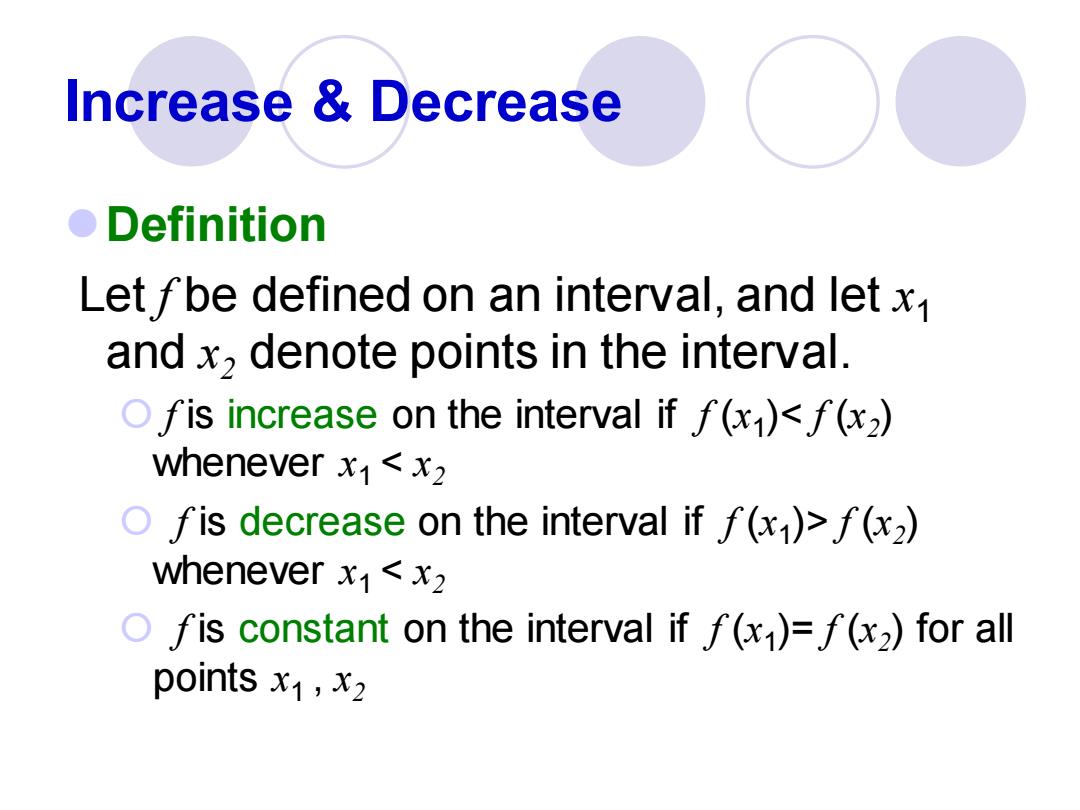
Increase Decrease Definition Let fbe defined on an interval,and let x1 and x,denote points in the interval. ofis increase on the interval if f(x1)f(x2) wheneverx1<x2 o fis constant on the interval if f(x)=f(x2)for all points x1,x2
Increase & Decrease ⚫Definition Let f be defined on an interval, and let x1 and x2 denote points in the interval. f is increase on the interval if f (x1 ) f (x2 ) whenever x1 < x2 f is constant on the interval if f (x1 )= f (x2 ) for all points x1 , x2
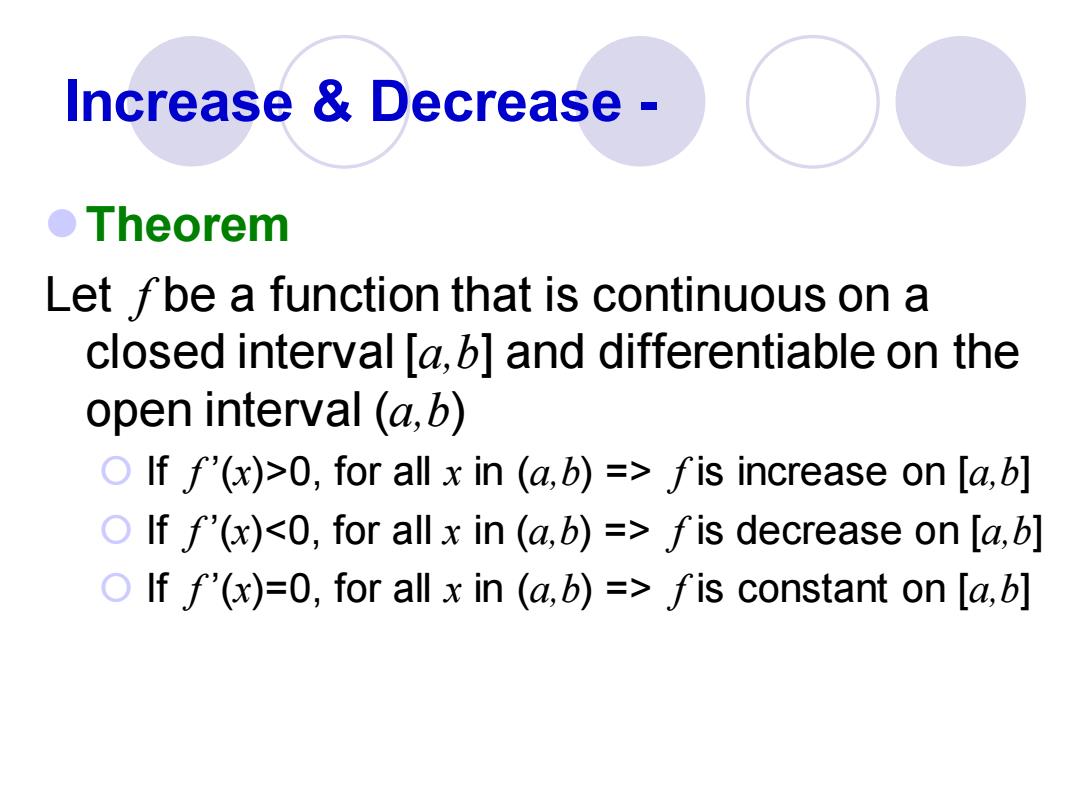
Increase Decrease ●Theorem Let fbe a function that is continuous on a closed interval [a,b]and differentiable on the open interval (a,b) O If f(x)>0,for all x in (a,b)=>fis increase on [a,b] O If f'(x)fis decrease on [a,b] O If f'(x)=0,for all x in (a,b)=>fis constant on [a,b]
Increase & Decrease - ⚫Theorem Let f be a function that is continuous on a closed interval [a,b] and differentiable on the open interval (a,b) If f ’(x)>0, for all x in (a,b) => f is increase on [a,b] If f ’(x) f is decrease on [a,b] If f ’(x)=0, for all x in (a,b) => f is constant on [a,b]

Concavity ●Definition O If fis differentiable on an open interval I,then f is said to be concave up on Tif f'is increasing on o fis said to be concave down on Iif f'is decreasing on I
Concavity ⚫Definition If f is differentiable on an open interval I, then f is said to be concave up on I if f ’ is increasing on I f is said to be concave down on I if f ’ is decreasing on I

Concavity ●Theorem O If f"(x)>0 for all value of x in I,then fis concave up on 2 O If f"(x)<0 for all value of x in I,then fis concave down on 2
Concavity - ⚫Theorem If f ’’(x)>0 for all value of x in I, then f is concave up on I If f ’’(x)<0 for all value of x in I, then f is concave down on I

Inflection Points o Definition If fis continuous on an open interval containing a value xo and if fchange the direction of concavity at the point (xo f(x)), then we say that fhas an inflection point at xo and we call the point (xo,f())on the graph of fan inflection point of f
Inflection Points ⚫Definition If f is continuous on an open interval containing a value x0 and if f change the direction of concavity at the point (x0 , f (x0 ) ), then we say that f has an inflection point at x0 and we call the point (x0 , f (x0 ) ) on the graph of f an inflection point of f

Relative Extrema Definition OA function fis said to have a relative maximum at xo if there is an open interval containing xo on which fo)is the largest value,i.e.fxo)x)for all x in the interval OA function f is said to have a relative minimum at xo if there is an open interval containing xo on which f(xo)is the smallest value,i.e.fxo)x)for all x in the interval
Relative Extrema ⚫Definition A function f is said to have a relative maximum at x0 if there is an open interval containing x0 on which f(x0 ) is the largest value, i.e. f(x0 )≥f(x) for all x in the interval A function f is said to have a relative minimum at x0 if there is an open interval containing x0 on which f(x0 ) is the smallest value, i.e. f(x0 )≤f(x) for all x in the interval

Relative Extrema O Theorem Suppose that fis a function defined on an open interval containing the point xo.If f has a relative extreme atx=xo,then x=xo is a critical point of f;that is,either f'(x)=0 or fis not differentiable at xo
Relative Extrema - ⚫Theorem Suppose that f is a function defined on an open interval containing the point x0 . If f has a relative extreme at x = x0 , then x= x0 is a critical point of f ; that is , either f ’(x0 )=0 or f is not differentiable at x0

First Derivative Test ●Theorem Suppose that fis continuous at a critical point xo O If f'(x)>0 on an open interval extending left from xo and f'(x)0 on an open interval extending right from xo,then f has a relative minimum at xo OIf f'(x)has the same sign on an open interval extending left from xo as it does on an open interval extending right from xo, then fdoes not have a relative extreme at xo
First Derivative Test ⚫ Theorem Suppose that f is continuous at a critical point x0 If f ’(x) >0 on an open interval extending left from x0 and f ’(x)0 on an open interval extending right from x0 , then f has a relative minimum at x0 If f ’(x) has the same sign on an open interval extending left from x0 as it does on an open interval extending right from x0 , then f does not have a relative extreme at x0

Second Derivative Test ●Theorem Suppose that fis twice differentiable at the point xo OIf f'(xo)=0 and f"(xo)>0,then f has a relative minimum at xo Olf f(x)=0 and f"(xo)<0,then f has a relative maximum at xo O If f'(xo)=0 and f"(xo)=0,then the test is inconclusive
Second Derivative Test ⚫Theorem Suppose that f is twice differentiable at the point x0 If f ’(x0 )=0 and f ’’(x0 )>0, then f has a relative minimum at x0 If f ’(x0 )=0 and f ’’(x0 )<0, then f has a relative maximum at x0 If f ’(x0 )=0 and f ’’(x0 )=0, then the test is inconclusive