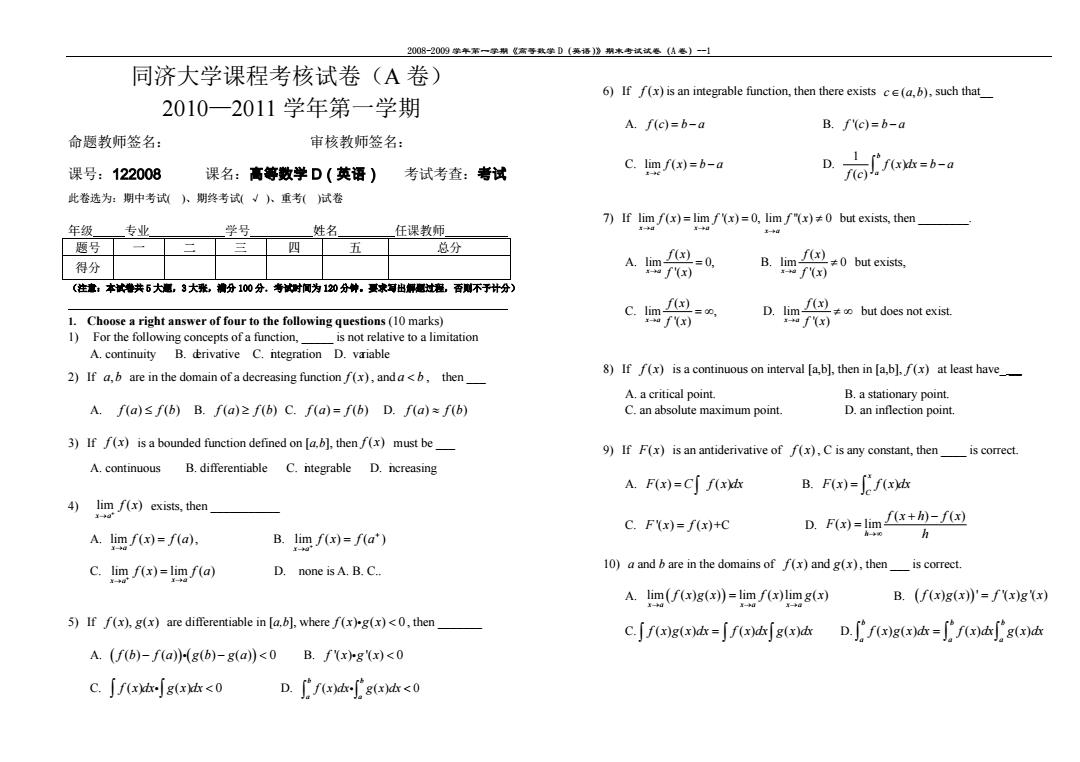
2008-2009学第一学期《高等学D(英语)》期季试试(墨)-」 同济大学课程考核试卷(A卷) 6)If f(x)is an integrable function,then there exists ce(a,b),such that 2010一2011学年第一学期 A.f(c)=b-a B.f'(c)=b-a 命题教师签名: 审核教师签名: 课号:122008 课名:高等数学D(英语)考试考查:考试 C.limf(x)=b-a 后a恤6-a D.1 此卷选为:期中考试(人、期终考试(√人重考(试卷 年级 专业 学号 姓名 任课教师 7)If lim/(x)lim()=0.lim/"(but exists,then 题号 三四 五 总分 得分 人得0 B=得0证es (注意:本试卷共5大愿,3大张槽分100分.考时间为120分钟.要录可出卿题过程,百则不子计分) 1.Choose a right answer of four to the following questions(10 marks) C. D=得版e做 1)For the following concepts of a function, is not relative to a limitation A.continuity B.derivative C.ntegration D.vaiable 2)If a,b are in the domain of a decreasing function f(x),anda<b,then 8)If f(x)is a continuous on interval [a,b],then in [a,b].f(x)at least have A.a critical point. B.a stationary point. A.fa)≤fb)B.f(a≥fb)c.f(a)=fb)D.fa)≈fb) C.an absolute maximum point. D.an inflection point 3)Iff(x)isa bounded function defined on [thenf(x)must be 9)If F(x)is an antiderivative of f(x),C is any constant,then is correct A.continuous B.differentiable C.ntegrable D.icreasing A.F(x)=Cf f(xyix B.F(x)=[f(xyds 4)limf(x)exists,then C.F(x)=f(x)+C Aimf田=fa, B.lim f(x)=f(a') D.F)=@+-因 h c.limf(x)=-limf()d) D.none is A.B.C.. 10)a and b are in the domains of f(x)and g(x),thenis correct. A.lim(f(x)g(x))=lim f(x)limg(x) B.(f(x)g(x))'=f(x)g(x) 5)If f(x),g(x)are differentiable in [a,b],where f(x)g(x)<0,then Cfx)gx)d=∫(x)dsg(x)ds.fx)gxd=∫fx)dgx)d A.(f(b)-f(a))(g(b)-g(a))<0 B.f(x)g'(x)<0 C.∫f(xyx-Jg(xdx<0 D.f(x)dxg(x)达<0
2008-2009 学年第一学期《高等数学 D(英语)》期末考试试卷(A 卷)--1 同济大学课程考核试卷(A 卷) 2010—2011 学年第一学期 命题教师签名:梁进 审核教师签名: 课号:122008 课名:高等数学 D(英语) 考试考查:考试 此卷选为:期中考试( )、期终考试( √ )、重考( )试卷 年级 专业 学号 姓名 任课教师 题号 一 二 三 四 五 总分 得分 (注意:本试卷共 5 大题,3 大张,满分 100 分.考试时间为 120 分钟。要求写出解题过程,否则不予计分) 1. Choose a right answer of four to the following questions (10 marks) 1) For the following concepts of a function, _____ is not relative to a limitation A. continuity B. derivative C. integration D. variable 2) If a b, are in the domain of a decreasing function f x( ) , and a b < , then ___ A. fa fb () () ≤ B. fa fb () () ≥ C. fa fb () () = D. fa fb () () ≈ 3) If f x( ) is a bounded function defined on [a,b], then f x( ) must be ___ A. continuous B. differentiable C. integrable D. increasing 4) lim ( ) x a f x → + exists, then ___________ A. lim ( ) ( ) x a fx fa → = , B. lim ( ) ( ) x a fx fa + + → = C. lim ( ) lim ( ) x a x a fx fa → + → = D. none is A. B. C.. 5) If f x gx ( ), ( ) are differentiable in [a,b], where f x gx () () 0 < , then _______ A. ( f b f a gb ga () () () () 0 − −< )( ) B. f xgx '( ) '( ) 0 < C. f x dx g x dx () () 0 < ∫ ∫ D. () () 0 b b a a f x dx g x dx < ∫ ∫ 6) If f x( ) is an integrable function, then there exists c ab ∈(,), such that__ A. fc b a ( ) = − B. fc ba '( ) = − C. lim ( ) x c fx b a → = − D. 1 ( ) ( ) b a f x dx b a f c = − ∫ 7) If lim ( ) lim '( ) 0, lim ''( ) 0 xa xa x a fx f x f x → → → = = ≠ but exists, then ________. A. ( ) lim 0, '( ) x a f x → f x = B. ( ) lim 0 '( ) x a f x → f x ≠ but exists, C. ( ) lim , '( ) x a f x → f x = ∞ D. ( ) lim '( ) x a f x → f x ≠ ∞ but does not exist. 8) If f x( ) is a continuous on interval [a,b], then in [a,b], f x( ) at least have_ A. a critical point. B. a stationary point. C. an absolute maximum point. D. an inflection point. __ 9) If F x( ) is an antiderivative of f x( ) , C is any constant, then ____ is correct. A. F x C f x dx () () = ∫ B. () () x C F x f x dx = ∫ C. Fx fx '( ) ( ) = +C D. ( ) () ( ) limh fx h fx F x →∞ h + − = 10) a and b are in the domains of f x gx ( ) and ( ) , then ___ is correct. A. lim ( ) ( ) lim ( )lim ( ) ( ) x a xa xa f xgx f x gx → → → = B. ( f xgx f xg x ( ) ( ) ' '( ) '( ) ) = C. f x g x dx f x dx g x dx ()() () () = ∫ ∫∫ D. ()() () () b bb a aa f x g x dx f x dx g x dx = ∫ ∫∫
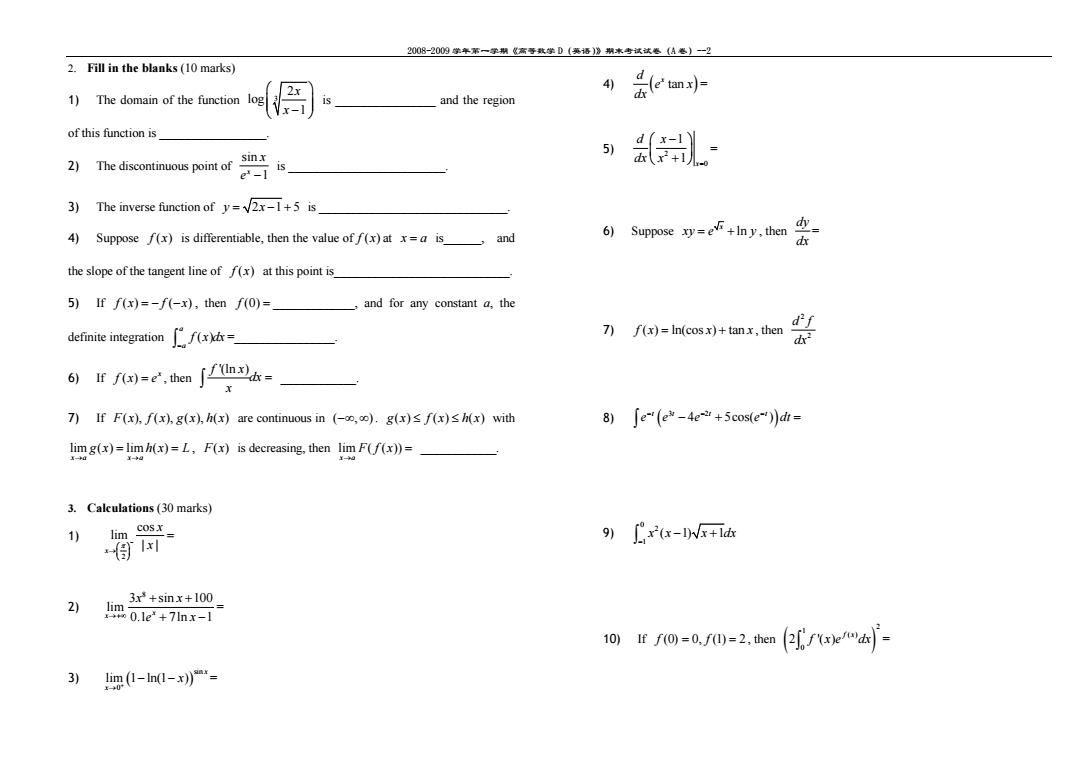
2008-200的学年常一学期《高等学D(语)》试试®()一2 2.Fill in the blanks (10 marks) 1)The domain of the function (2x os and the region of this function is 2)The discontinuous point of 3)The inverse function ofy=√2r-i+5is」 4)Suppose f(x)is differentiable,then the value of f(x)at x=a is,and 6sone-e+hy.m密 the slope of the tangent line of f(x)at this point is 5)If f(x)=-f(-x),then f(0)=_ and for any constant a,the definite integrationx- 7)f(x)=In(cosx)+tanx,then 6)rf)=e,hen∫ah。 x 7)If F(x),f(x),g(x),h(x)are continuous in (-o,).g(x)sf(x)sh(x)with 8)fe(e-4e+5cos(e-))di= limg(x)=limh(x)=L,F(x)is decreasing.then lim F(f(x)= 3.Calculations(30 marks) COSx ” 9)(x-1+id 2) 3x+sinx+100 lim o.le'+7Inx-1 10)I()=2.then (erus 3) 1io1-n0-x)=
2008-2009 学年第一学期《高等数学 D(英语)》期末考试试卷(A 卷)--2 2. Fill in the blanks (10 marks) 1) The domain of the function 3 2 log 1 x x − is ________________ and the region of this function is _________________. 2) The discontinuous point of sin 1 x x e − is _________________________. 3) The inverse function of y x = −+ 2 15 is ______________________________. 4) Suppose f x( ) is differentiable, then the value of f x( ) at x a = is______, and the slope of the tangent line of f x( ) at this point is____________________________. 5) If fx f x () ( ) =− − , then f (0) = _____________, and for any constant a, the definite integration ( ) a a f x dx ∫− =________________. 6) If ( ) x fx e = , then f x '(ln )dx x = ∫ ____________. 7) If Fx f x gx hx ( ), ( ), ( ), ( ) are continuous in ( ,) −∞ ∞ . gx f x hx () () () ≤ ≤ with lim ( ) lim ( ) xa xa gx hx L → → = = , F x( ) is decreasing, then lim ( ( )) x a Ffx → = ____________. 3. Calculations (30 marks) 1) 2 cos lim | | x x π x − → = 2) 8 3 sin 100 lim 0.1 7 ln 1 x x x x →+∞ e x + + + − = 3) ( ) sin 0 lim 1 ln(1 ) x x x → + − − = 4) ( tan ) d x e x dx = 5) 2 0 1 1 x d x dx x = − + = 6) Suppose ln x xy e y = + , then dy dx = 7) fx x x ( ) ln(cos ) tan = + , then 2 2 d f dx 8) ( ) 3 2 4 5cos( ) tt t t e e e e dt −− − − + ∫ = 9) 0 2 1 x x x dx ( 1) 1 − − + ∫ 10) If f f (0) 0, (1) 2 = = , then ( ) 2 1 ( ) 0 2 '( ) f x f x e dx ∫ =

2008-200的学平第一学期《高等数学D(英语)》期水季试试卷(A卷)-一3 4.Graph Analysis 5.Applications Analysis function f(x)=x+2x3-3x2: 1)(8 marks)Calculate the area of the region which is enclosed by functions 1)(3 marks)Write out all roots of f(x)if there exist; y-cosx and y1. 2)(3 marks)Write out all relative extreme points of f(x)if there exist, 2)(11 marks)A 3-m ladder is leaning against a wall.If the top of the ladder slips 3)(3 marks)Write out all inflection points of f(x)if there exist; down the wall at a constant rate of 0.3m/s, a)What is the rate function of the foot moving away from the wall,where the independent variable of the function is the distance between the bottom and the wall? b)What is the exact figure of the rate when the top is I m above the ground. 4)(3 marks)Write out the increase and decrease intervals of f(x); 5)(3 marks)Write out the concave up and concave down intervals of f(x) 3)(8 marks)A closed rectangular container with a square base is to have a volume of 6)(3 marks)Write out the infinite behaviors of f(x) 1000 cm'.It costs twice as much per square centimeter for the top as it does for the sides and bottom.Find the dimensions of the container of least cost. 7)(5 marks)Sketch the figure of the function
2008-2009 学年第一学期《高等数学 D(英语)》期末考试试卷(A 卷)--3 4. Graph Analysis Analysis function 4 32 fx x x x () 2 3 =+ − : 1) (3 marks) Write out all roots of f x( ) if there exist; 2) (3 marks) Write out all relative extreme points of f x( ) if there exist; 3) (3 marks) Write out all inflection points of f x( ) if there exist; 4) (3 marks) Write out the increase and decrease intervals of f x( ) ; 5) (3 marks) Write out the concave up and concave down intervals of f x( ) 6) (3 marks) Write out the infinite behaviors of f x( ) 7) (5 marks) Sketch the figure of the function 5. Applications 1) (8 marks) Calculate the area of the region which is enclosed by functions y x = cos and 2 y x| |1 π = − . 2) (11 marks) A 3-m ladder is leaning against a wall. If the top of the ladder slips down the wall at a constant rate of 0.3m/s, a) What is the rate function of the foot moving away from the wall, where the independent variable of the function is the distance between the bottom and the wall? b) What is the exact figure of the rate when the top is 1 m above the ground. 3) (8 marks) A closed rectangular container with a square base is to have a volume of 1000 cm3 . It costs twice as much per square centimeter for the top as it does for the sides and bottom. Find the dimensions of the container of least cost