Statistical Thermodynamics PDF文件使用"pdfFactory Pro”试用版本创建fm,fineprint.com,cn
Statistical Thermodynamics PDF 文件使用 "pdfFactory Pro" 试用版本创建 fwww.fineprint.com.cn
Introduction The equilibrium properties of matter may be considered from two points of view:the macroscopic and the microscopic. Thermodynamics is a macroscopic view which describes the behavior of large numbers of molecules in terms of pressure, volume,composition,and exchanges of heat and work.The quantitative relationships between various measured properties are not based on any model of the microscopic structure of matter. On the other hand,quantum mechanics provides a microscopic description for the structure and interactions of molecules. Ideally,we would like to be able to predict the thermodynamic behavior of substances using our knowledge about individual molecules obtained from spectroscopic measurements and from theoretical calculations of wavefunctions. PDF文件使用"pdfFactory Pro”试用版本创建fm,fineprint.com,cn
Introduction The equilibrium properties of matter may be considered from two points of view: the macroscopic and the microscopic. Thermodynamics is a macroscopic view which describes the behavior of large numbers of molecules in terms of pressure, volume, composition, and exchanges of heat and work. The quantitative relationships between various measured properties are not based on any model of the microscopic structure of matter. On the other hand, quantum mechanics provides a microscopic description for the structure and interactions of molecules. Ideally, we would like to be able to predict the thermodynamic behavior of substances using our knowledge about individual molecules obtained from spectroscopic measurements and from theoretical calculations of wavefunctions. PDF 文件使用 "pdfFactory Pro" 试用版本创建 ÿwww.fineprint.com.cn f

Statistical mechanics provides the needed bridge between microscopic mechanics(classical and quantum)and macroscopic thermodynamics. Statistical mechanics provides insight into the laws of thermodynamics,and through it we will see heat, work,temperature,irreversible processes,and state functions in a new light. PDF文件使用"pdfFactory Pro”试用版本创建fm,fineprint.com,cn
Statistical mechanics provides the needed bridge between microscopic mechanics( classical and quantum) and macroscopic thermodynamics. Statistical mechanics provides insight into the laws of thermodynamics, and through it we will see heat, work, temperature, irreversible processes, and state functions in a new light. PDF 文件使用 "pdfFactory Pro" 试用版本创建 ÿwww.fineprint.com.cn f

The Concepts 统计系统的分类 1独立子系统与相依子系统 独立子系统:粒子间相互作用力可以忽略的系统 For example:ideal gas 相依子系统:粒子间相互作用力不可忽略的系统 For example:real gas 我们只讨论独立子系统。 PDF文件使用"pdfFactory Pro”试用版本创建fwm,fineprint..com,cn
The Concepts 一、统计系统的分类 1.独立子系统与相依子系统 独立子系统:粒子间相互作用力可以忽略的系统 For example:ideal gas 相依子系统:粒子间相互作用力不可忽略的系统 For example:real gas 我们只讨论独立子系统。 PDF 文件使用 "pdfFactory Pro" 试用版本创建 fwww.fineprint.com.cn

2.离域子系统与定域子系统 离域子系统:粒子处于混乱状态,没有固定位置 ,各粒子彼此无法分辨。离域子也称为等同粒 子。 For example:gas,ideal gas,是独立离域子系统 •定域子系统粒子有固定的平衡位置,它们运动 是定域化的,对不同位置的粒子可以编号区分 。定域子也称为可辨粒子。 For example:crystal是定域子系统 PDF文件使用"pdfFactory Pro”试用版本创建fm,fineprint.com,cn
2. 离域子系统与定域子系统 •离域子系统:粒子处于混乱状态,没有固定位置 ,各粒子彼此无法分辨。离域子也称为等同粒 子。 For example:gas, ideal gas是独立离域子系统 •定域子系统:粒子有固定的平衡位置,它们运动 是定域化的,对不同位置的粒子可以编号区分 。定域子也称为可辨粒子。 For example :crystal 是定域子系统 PDF 文件使用 "pdfFactory Pro" 试用版本创建 ÿwww.fineprint.com.cn f

二、粒子的运动形式(The basic modes of motion) 1.平动(translation):分子在空间的整体运动。( )(motion through space in three dimensions) 2.转动(rotation):分子绕质心的转动。(二或三 维) 3.振动(vibration):原子在平衡位置附近的振动 4.电子运动(electron):分子内电子运动。 5.核运动(nucleus):分子内原子核运动。 若各种能量的运动形式彼此独立,则粒子的运动能 量为:e=e十e,+e,十ee十em PDF文件使用"pdfFactory Pro”试用版本创建酸能,fineprint.com,cn
二、粒子的运动形式(The basic modes of motion) 1.平动(translation):分子在空间的整体运动。( 三维)(motion through space in three dimensions) 2.转动(rotation):分子绕质心的转动。(二或三 维) 3.振动(vibration):原子在平衡位置附近的振动 4.电子运动(electron):分子内电子运动。 5.核运动(nucleus):分子内原子核运动。 若各种能量的运动形式彼此独立,则粒子的运动能 量为: e=et+er +ev +ee +en PDF 文件使用 "pdfFactory Pro" 试用版本创建 駿ÿ www.fineprint.com.cn

三、粒子运动的自由度数(Degrees of freedom of motion) 一个由N个原子组成的分子,运动的总自由度为3N。 自由度(3N) 平动 转动 振动 N原子 3 3 3N-6 单原子 3 0 0 双原子 3 2 1 PDF文件使用"pdfFactory Pro”试用版本创建晦.fineprint.com,cn
一个由N个原子组成的分子,运动的总自由度为3N。 三、粒子运动的自由度数(Degrees of freedom of motion) 自由度(3N) 平动 转动 振动 N原子 3 3 3N-6 单原子 3 0 0 双原子 3 2 1 PDF 文件使用 "pdfFactory Pro" 试用版本创建 ÿ昀www.fineprint.com.cn
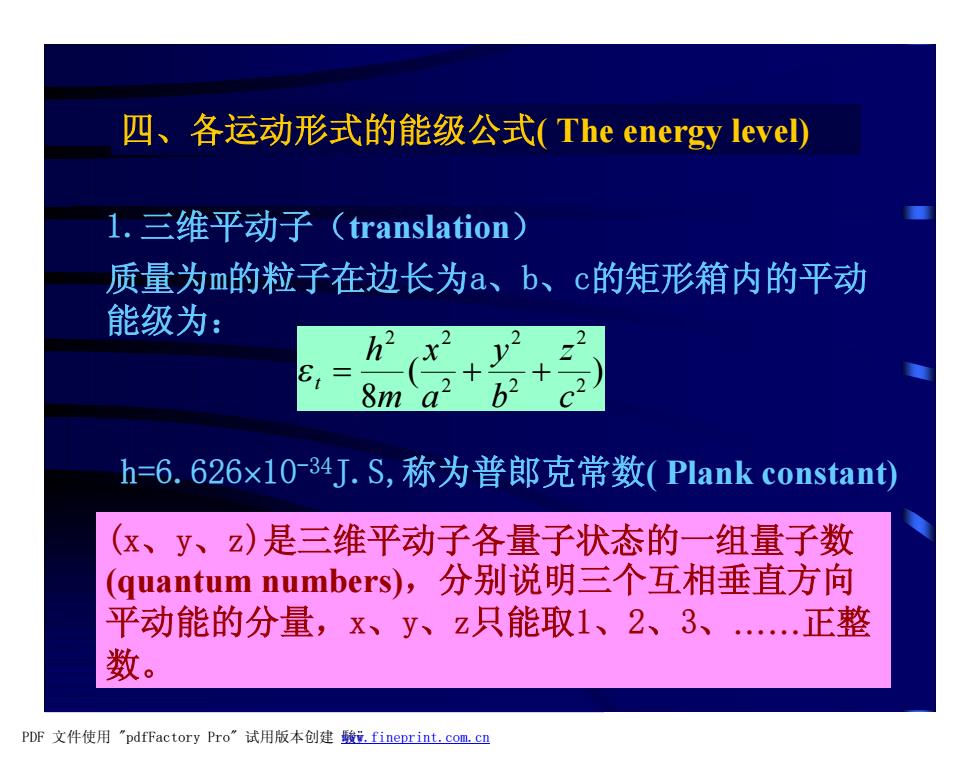
四、各运动形式的能级公式(The energy level) 1.三维平动子(translation) 质量为m的粒子在边长为a、b、c的矩形箱内的平动 能级为: 8m ae h=6.626x10-34J.S,称为普郎克常数(Plank constant) (x、y、z)是三维平动子各量子状态的一组量子数 (quantum numbers),分别说明三个互相垂直方向 平动能的分量,x、y、z只能取1、2、3、.正整 数。 PDF文件使用"pdfFactory Pro”试用版本创建駿能,fineprint.com,cn
四、各运动形式的能级公式( The energy level) 1.三维平动子(translation) 质量为m的粒子在边长为a、b、c的矩形箱内的平动 能级为: ( ) 8 2 2 2 2 2 2 2 c z b y a x m h et = + + h=6.626´10-34J.S,称为普郎克常数( Plank constant) .(x、y、z)是三维平动子各量子状态的一组量子数 (quantum numbers),分别说明三个互相垂直方向 平动能的分量,x、y、z只能取1、2、3、¼¼正整 数。 PDF 文件使用 "pdfFactory Pro" 试用版本创建 駿ÿ www.fineprint.com.cn

若a=b=c粒子在立方箱中则: h2 8: ++ 8m a- a 8mr2(r2+y2+z2 Discussion: 1)平动能基态(the lowest state):x,y,z=l,1,1,很小。 2)8=f(m,V) 3)有简并度(degenerate,.meaning that they correspond to the same energy)),例:第一微发态(the first excited state)可取(1,1,2),(1,2,1),(2,1, 1),g=3 4)△e/kT~10-19,量子效应(quantization)不明显,可近似 认为平动能量是连续的。 PDF文件使用"pdfFactory Pro”试用版本创建m,fineprint.com,c里
若a=b=c粒子在立方箱中则: ( ) 8 ( ) 8 2 2 2 2 / 3 2 2 2 2 2 2 2 2 x y z mV h a z a y a x m h et = + + = + + Discussion: 1)平动能基态( the lowest state):x,y,z=1,1,1,e0很小。 2)et =f(m,V) 3)有简并度(degenerate, meaning that they correspond to the same energy),例:第一激发态(the first excited state)可取(1,1,2),(1,2,1),(2,1, 1),gi=3 4) De/kT~10-19 ,量子效应(quantization)不明显,可近似 认为平动能量是连续的。 PDF 文件使用 "pdfFactory Pro" 试用版本创建 ÿwww.fineprint.com.cn ÿ

2.刚性直线转子(双原子分子)Rotational motion) 刚性直线转子是指原子间距离R不变的转子。 能级公式:8,=J(J+1)h2/8π2I J为转动量子数J=0,1,2,3...正整数 I=μR,2一转动惯量 μ=血m2/(m1+m2)--折合质量。 PDF文件使用"pdfFactory Pro”试用版本创建m,fineprint..com,cn
2.刚性直线转子(双原子分子)(Rotational motion) 刚性直线转子是指原子间距离R0不变的转子。 J为转动量子数 J=0,1,2,3¼¼正整数 I=mR0 2 ---转动惯量 m=m1m2/(m1+m2)---折合质量。 能级公式: er=J(J+1)h2/8p 2I PDF 文件使用 "pdfFactory Pro" 试用版本创建 f www.fineprint.com.cn Ì