
Bioheat Transfer 生物传热学 Lecture 张爱丽zhangailic@sjtu.ed山.cn TA: 忻颖 novelxiaoxin@yahoo.com.cn
Bioheat Transfer 生物传热学 Lecture :张爱丽 zhangaili@sjtu.edu.cn TA: 忻颖 novelxiaoxin@yahoo.com.cn

Course Description Homework assignment and personal presence: 20% Final examination:80%
Course Description Homework assignment and personal presence: 20% Final examination: 80%

Textbook: Thermal Dosimetry and Treatment Planning,JC Chato, KD Paulsen,RB Roemer,M.Gautherie (Ed.),Springer-Verlag Berlin Heidelberg New York, London,Paris,Tokyo,Hongkong,1990. ■Referred books: Heat transfer in Medicine and Biology,A.Shitzer, Robert C.Eberhart,Plenum Press,New York and London,1985 Heat Transfer Essentials,Latif M.Jiji,Begell House Inc.,Publishers,New York,1998
Textbook: Thermal Dosimetry and Treatment Planning, JC Chato, KD Paulsen, RB Roemer, M. Gautherie (Ed.), Springer-Verlag Berlin Heidelberg New York, London, Paris, Tokyo, Hongkong, 1990. Referred books: Heat transfer in Medicine and Biology, A. Shitzer, Robert C. Eberhart, Plenum Press, New York and London, 1985 Heat Transfer Essentials, Latif M. Jiji, Begell House Inc., Publishers, New York, 1998

Introduction
Introduction
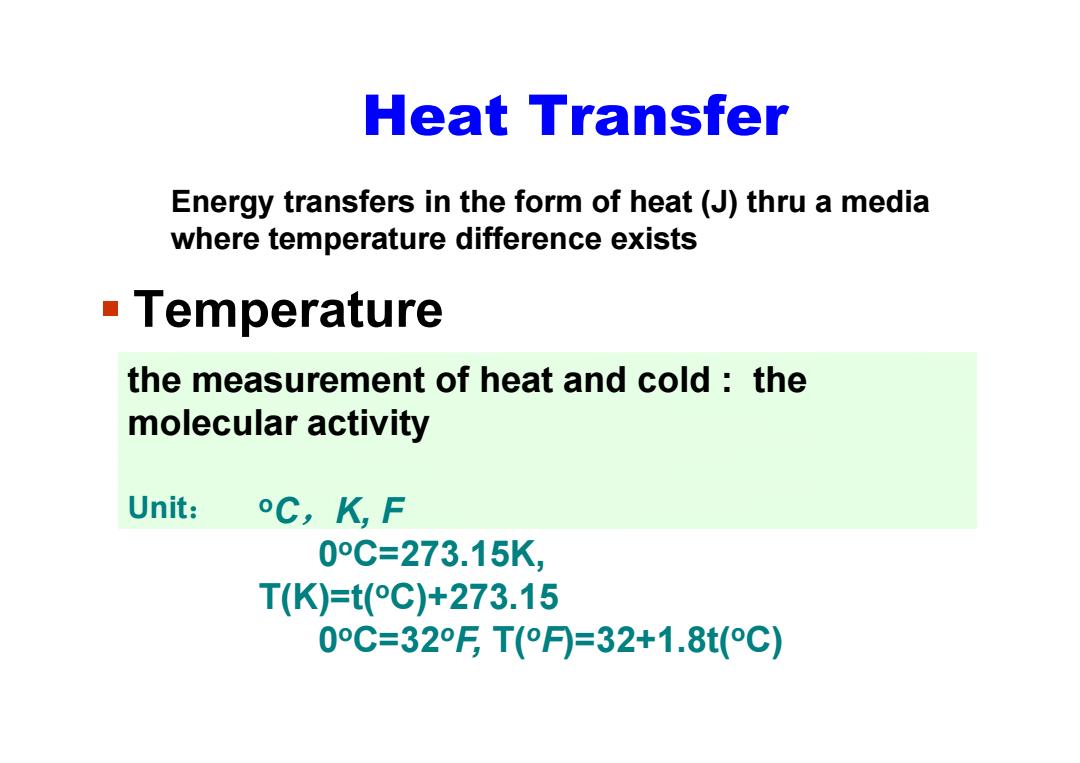
Heat Transfer Energy transfers in the form of heat(J)thru a media where temperature difference exists Temperature the measurement of heat and cold the molecular activity Unit: oC,K,F 0C=273.15K, T(K)=t(°C)+273.15 0C=32FT(°月=32+1.8t(oC)
Heat Transfer Temperature the measurement of heat and cold : the molecular activity Unit: Energy transfers in the form of heat (J) thru a media where temperature difference exists o C ,K, F 0 oC=273.15K, T(K)=t( oC)+273.15 0 oC=32 oF, T( o F)=32+1.8t( oC)

9.=-K40 Three Modes of Heat Transfer Conduction(Diffusion) a.Media:stationary solid,gas or liquid at diff b.Mechanism:molecular activities,free electrons or atomic vibrations c.Rate equ. d.=-kA E -Fourier's Law dx
Three Modes of Heat Transfer Conduction (Diffusion) a. Media: stationary solid, gas or liquid at diff. b. Mechanism: molecular activities, free electrons or atomic vibrations c. Rate equ. dx dT qx = −KA dx dT qx = −KA ——Fourier’s Law dx dT q x = − kA
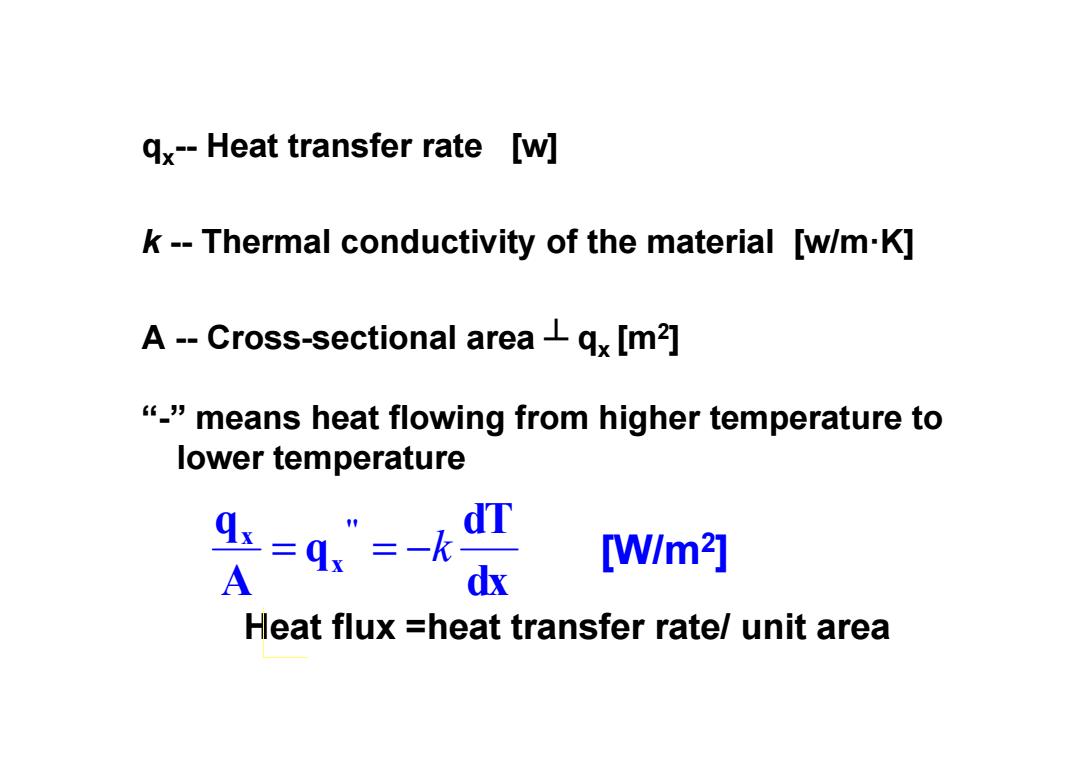
qx--Heat transfer rate [w] k--Thermal conductivity of the material [w/m-K] A--Cross-sectional areaqx [m2] "means heat flowing from higher temperature to lower temperature dT =q”=-k [W/m2] A dx Heat flux =heat transfer rate/unit area
qx-- Heat transfer rate [w] k -- Thermal conductivity of the material [w/m·K] A -- Cross-sectional area ┴ qx [m2] “-” means heat flowing from higher temperature to lower temperature dx dT q Aq '' x x = = −k [W/m2] Heat flux =heat transfer rate/ unit area

9.=-K4 4 Convection a Media:between solid moving fluid b.Mechanism:Bulk motion effects c.Rate equ. g=hA(T-T) Newton's law of cooling
Convection a Media: between solid & moving fluid b. Mechanism: Bulk motion effects c. Rate equ. dx dT qx = −KA Newton’s law of cooling hA(T T ) = s − ∞ q
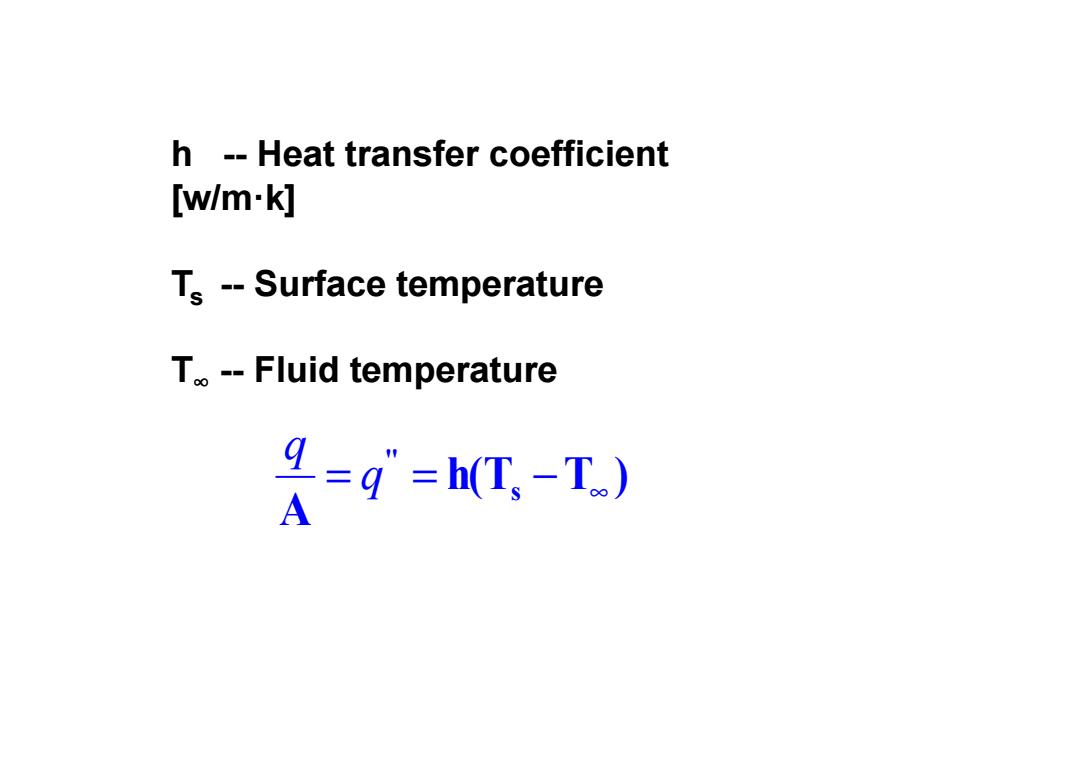
h--Heat transfer coefficient [w/m-k] Ts--Surface temperature T--Fluid temperature =q=h(T,-T) A
h -- Heat transfer coefficient [w/m·k] Ts -- Surface temperature T ∞ -- Fluid temperature h(T T ) A s '' = = − ∞ q q

Radiation a.Media:none b.Mechanism:electromagnetic waves (EM) Any surface at a finite temp.higher than zero K emits heat in the form of EM waves. The intensity of the wave is related to temp. c.Rate equation: g=80T A
Radiation a. Media: none b. Mechanism: electromagnetic waves (EM) Any surface at a finite temp. higher than zero K emits heat in the form of EM waves. The intensity of the wave is related to temp. c. Rate equation: As q 4s = εσT