
Lecture 6 Thermal Resistance Method
Lecture 6 Thermal Resistance Method
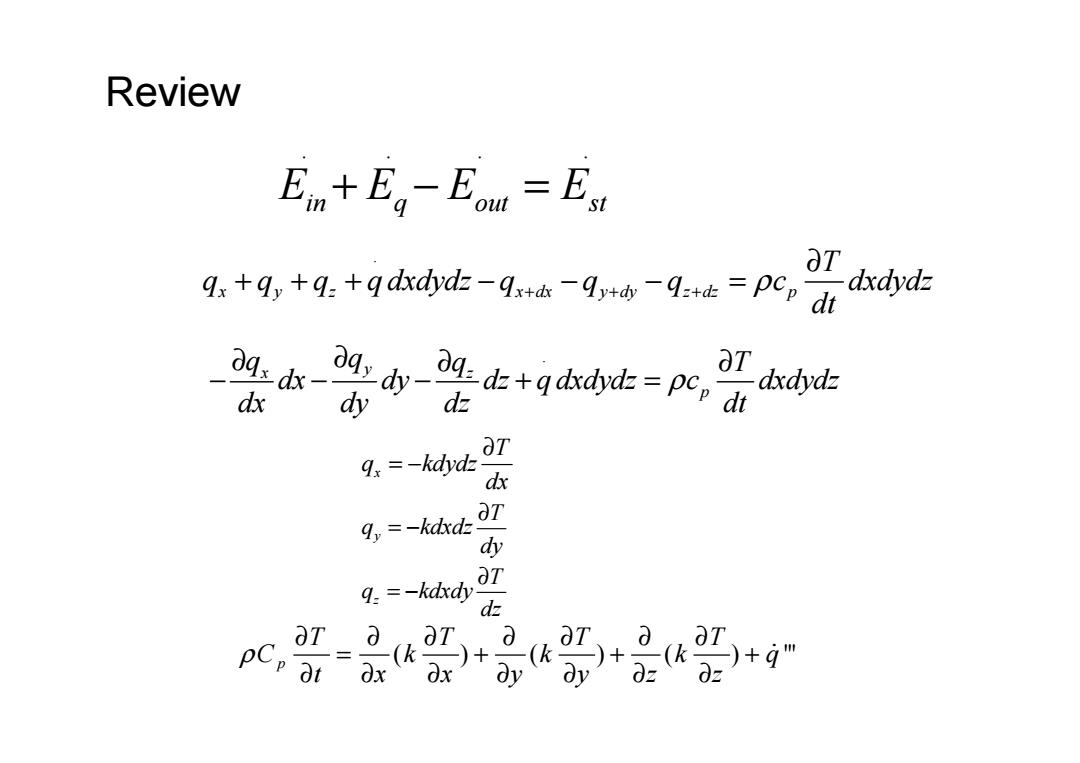
Review Eun+E-Eou Est xdyd gs+gy+g:+qdxdyd=-gxwih-gyw-g=PCp di ods dx dy T dxdyd dx ∂ay dy-d也 dqdxdyd=pc, aT 9s =-kdydz 9,=-hdbxd OT dy ∂T g:=-kaxdy dz aT∂,aT ∂T (k )+ (k ay dy )+ ∂z T)4" (k
.. . . Ein q out st +− = EE E Review . x y z x dx y dy z dz p T q q q q dxdydz q q q c dxdydz dt +++ ρ ∂ +++ − − − = x T q kdydz dx ∂ = − y T q kdxdz dy ∂ = − z T q kdxdy dz ∂ = − . x y z p q q q T dx dy dz q dxdydz c dxdydz dx dy dz dt ρ ∂ ∂ ∂ ∂ −−−+ = ( ) ( ) ( ) ''' p TT T T C k k kq txx yy zz ρ ∂ ∂∂ ∂∂ ∂∂ =+++ ∂∂ ∂ ∂ ∂ ∂ ∂
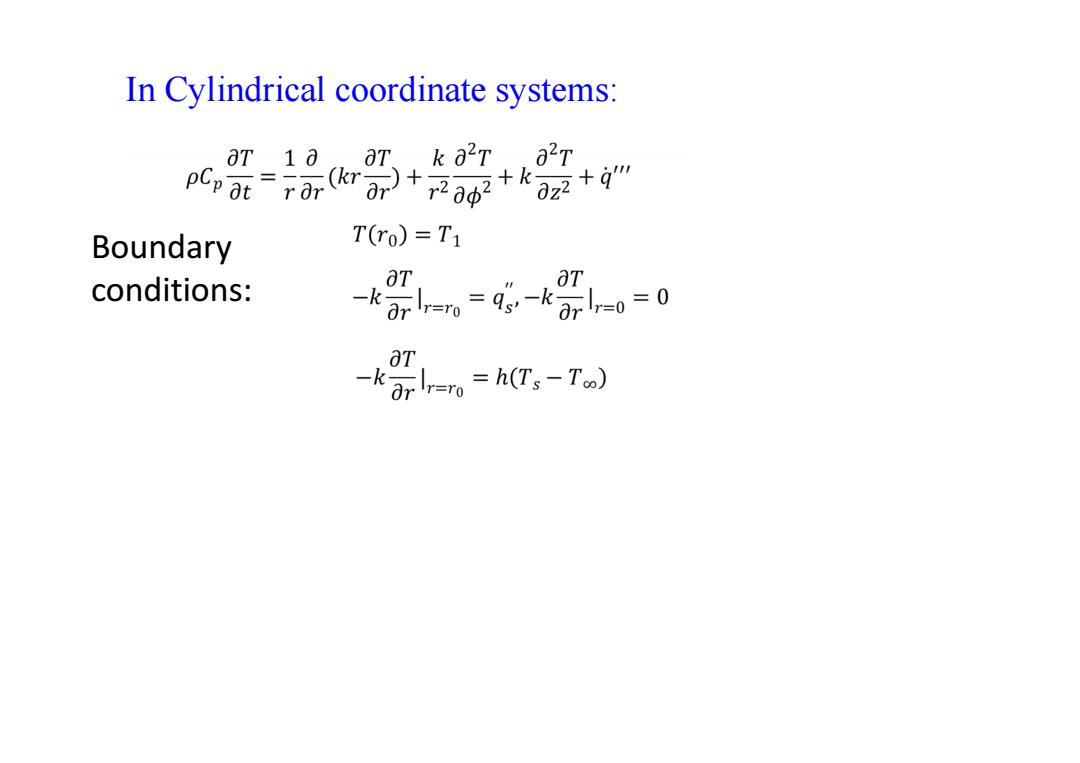
In Cylindrical coordinate systems: ∂T1∂ aT、,kaT,,∂T pcp-(ra+0+k2+g” Boundary T(ro)=T1 conditions: -k81n=4g-kn=0 OT aT -k8n=hs-T)
In Cylindrical coordinate systems: Boundary conditions:
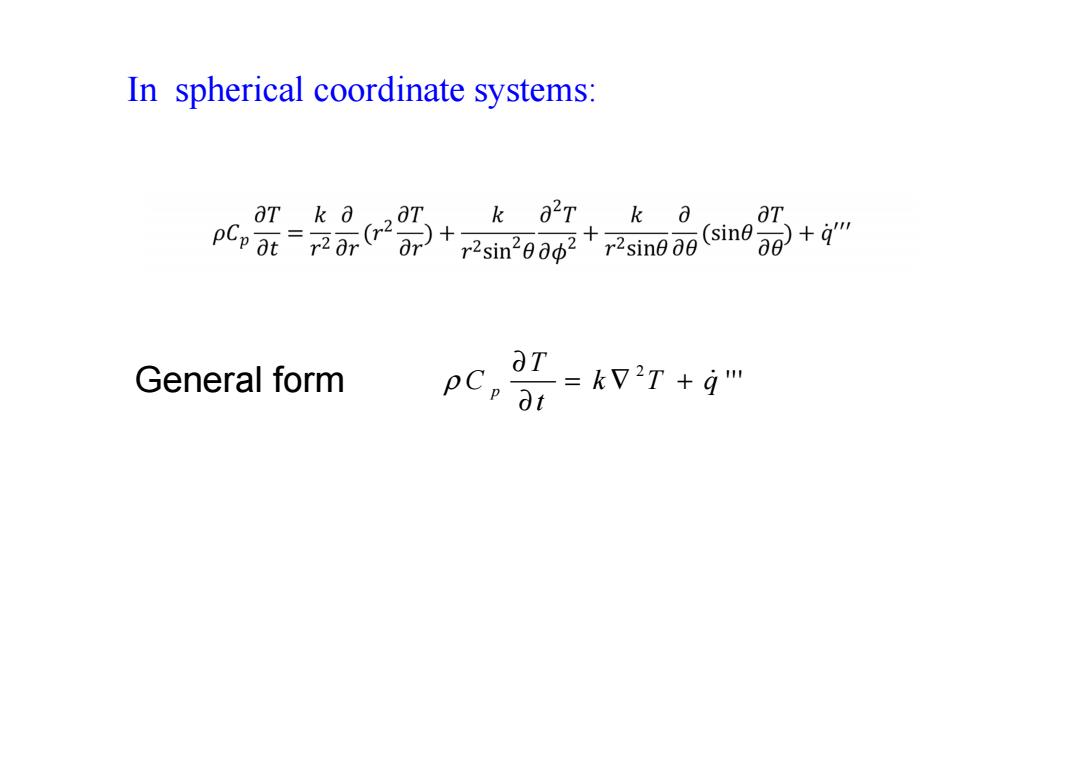
In spherical coordinate systems: pc部-点品r部+点+点品0+” k a2T k d OT General form ∂T=kV2T+g
In spherical coordinate systems: 2 ''' p T C kTq t ρ ∂ =∇ + ∂ General form
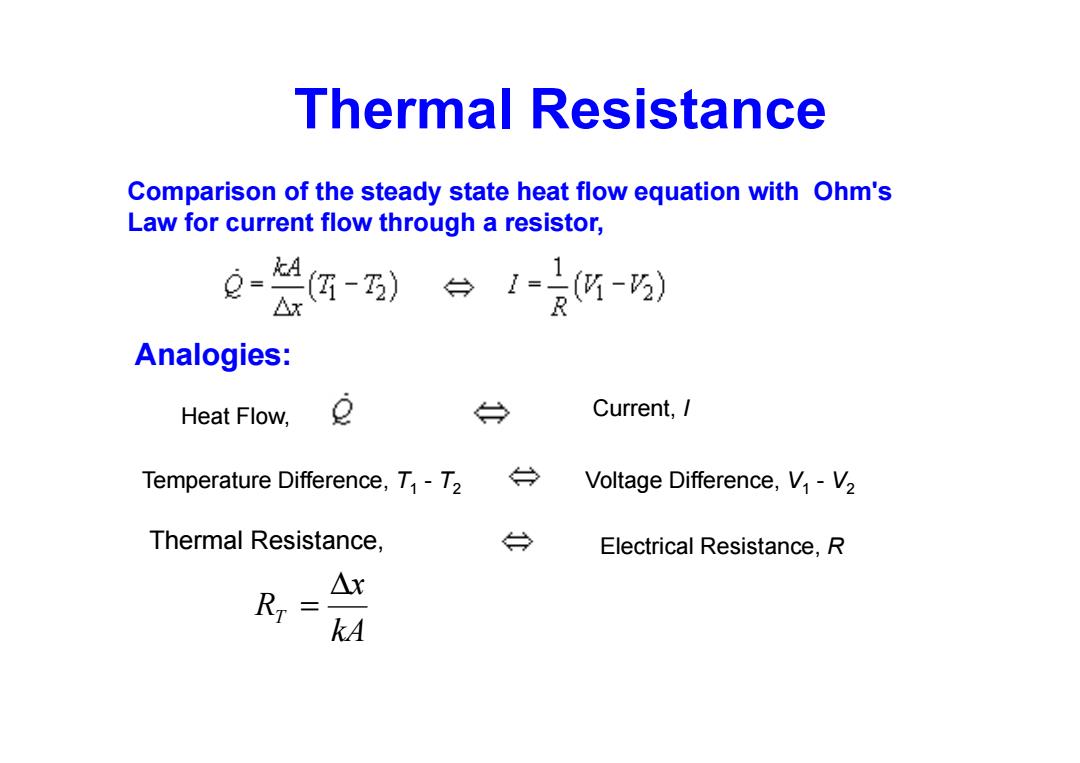
Thermal Resistance Comparison of the steady state heat flow equation with Ohm's Law for current flow through a resistor, Q-4图-) △x 白1最-) Analogies: Heat Flow, 台 Current, Temperature Difference,T1-T2 台 Voltage Difference,V -V2 Thermal Resistance, 告 Electrical Resistance,R △x RT= kA
Thermal Resistance Analogies: Comparison of the steady state heat flow equation with Ohm's Law for current flow through a resistor, Heat Flow, Current, I Temperature Difference, T1 - T2 Voltage Difference, V1 - V2 Thermal Resistance, Electrical Resistance, R kA x R T Δ =

1-D wall,Composite Wall Contact resistance Heating source Thermal Resistance in Radial Systems
1-D wall, Composite Wall Contact resistance Heating source Thermal Resistance in Radial Systems
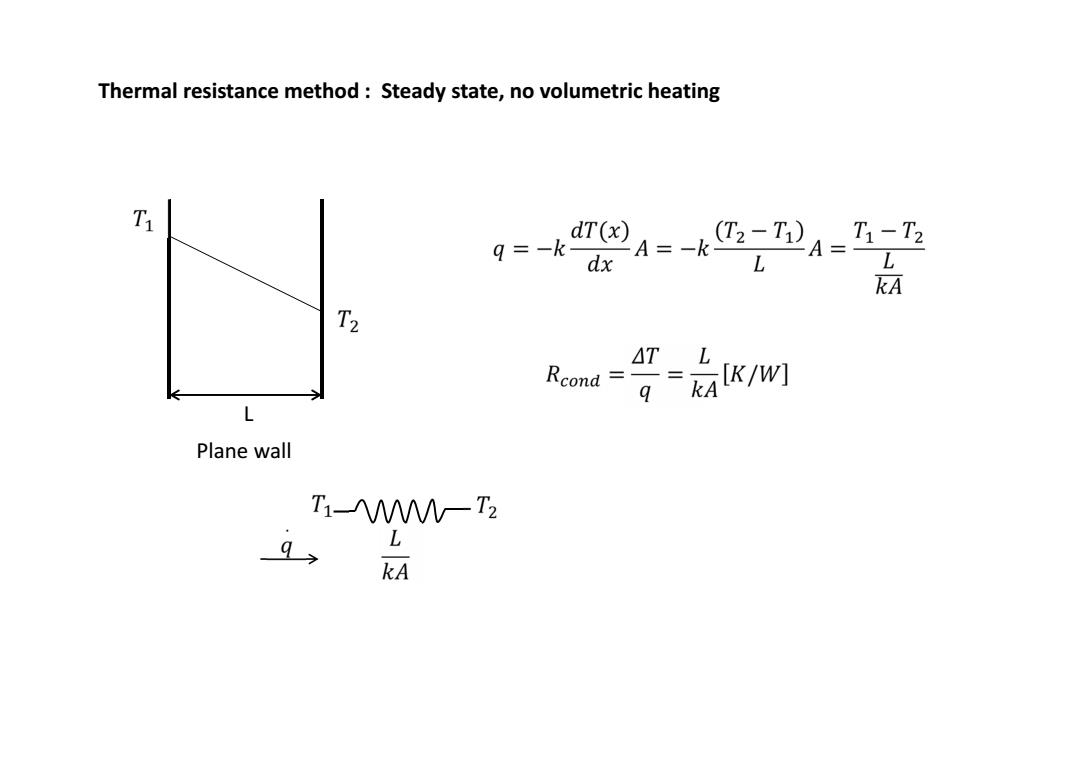
Thermal resistance method:Steady state,no volumetric heating arC四A=-k,T型A=-2 q=-k-dx L kA T2 △TL 9-kAl K/W] L Plane wall T--T2 L kA
Thermal resistance method : Steady state, no volumetric heating L Plane wall
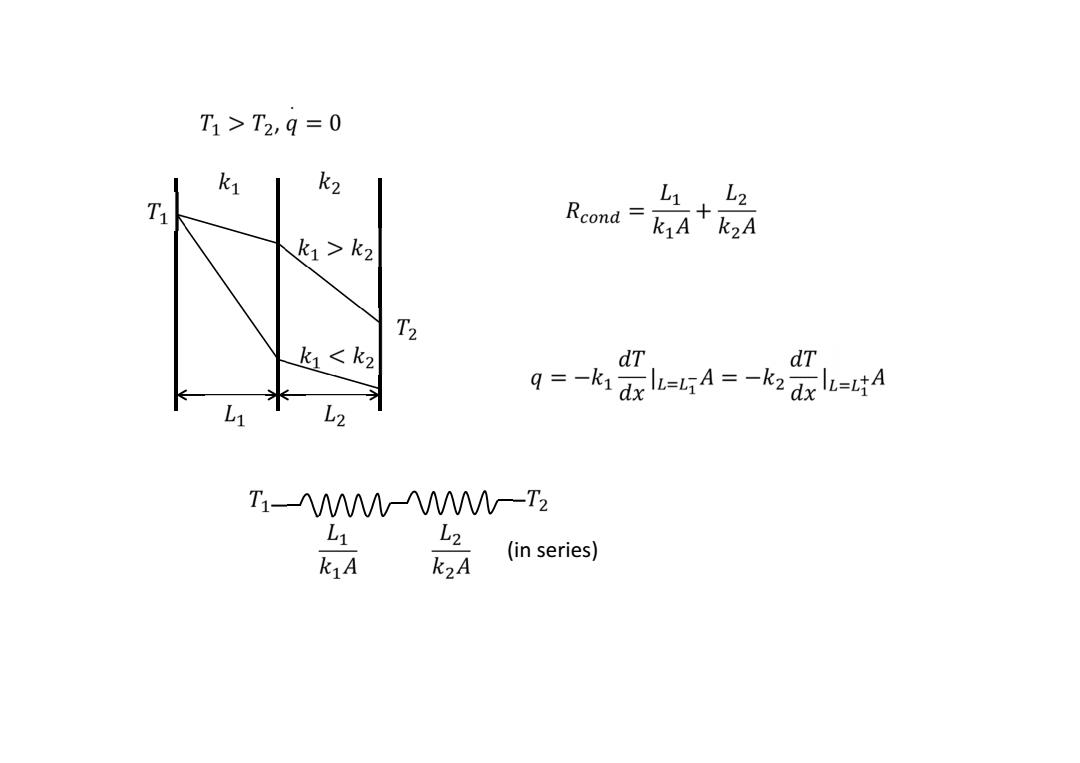
T1>T2,q=0 Ky k2 2 T Rcond= L1+ k A k2A k1>k2 T2 k1<k2 g=-le54=-0-4 L2 T--T2 L1 L2 k1A A (in series)
(in series)

9=0 k K2 T2 A A2 A2+A3=A1 q2+q3=91 3 q3 L2 名4 T- T2 M Ls In parallel k3A
In parallel

Contact Resistance T-T哇 Rt.c= 7 L T2 q"A Unit area contact resistance is: k2 R2-,m2-Km Thus T-MT2 L1 RtcA L2 kA k2A
Contact Resistance Unit area contact resistance is: Thus