
Matrix Theory School of Mathematical Sciences Teaching Group Main Reference books Fuzhen Zhang.Matrix Theory-Basic Results and Techniques,Second Edition. Springer,2011. llse C.F.Ipsen,Numerical Matrix Analysis:Linear Systems and Least Squares. SIAM,2009. Reference books: Roger A.Horn and Charles A.Johnson:Matrix Analysis.Cambridge University Press,1985. Gene H.Golub and Charles F.Van Loan:Matrix Computations,Third Edition. Johns Hopkins Press,1996. Nicholas J.Higham.Accuracy and Stability of Numerical Algorithms,Second Edition.SIAM,2002. Y.Saad.Iterative Methods for Sparse Linear Systems,Second Edition.SIAM, Philadelphia,2003. Matrix Theory Matrices Maintained by Yan-Fei Jing
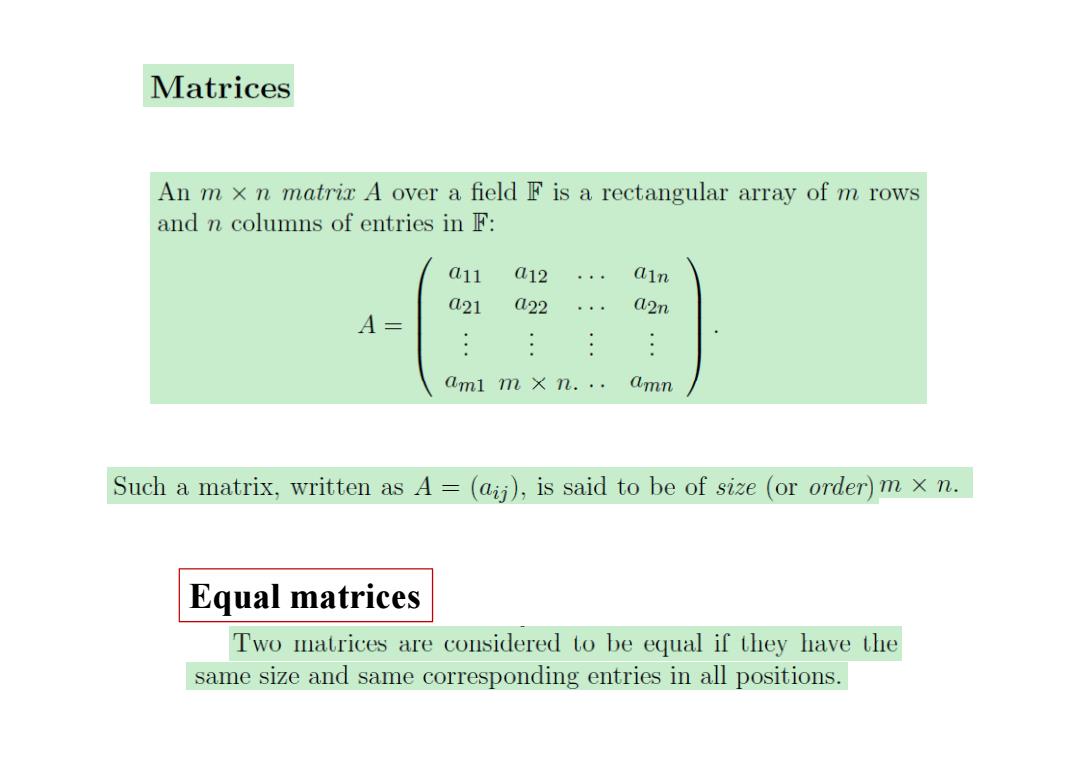
Matrices An m x n matrix A over a field F is a rectangular array of m rows and n columns of entries in F: 11 a12 ain a21 a22 02m A= .. : : am1m×n.·· amn Such a matrix,written as A=(aij),is said to be of size (or order)m x n. Equal matrices Two matrices are considered to be equal if they have the same size and same corresponding entries in all positions
Equal matrices
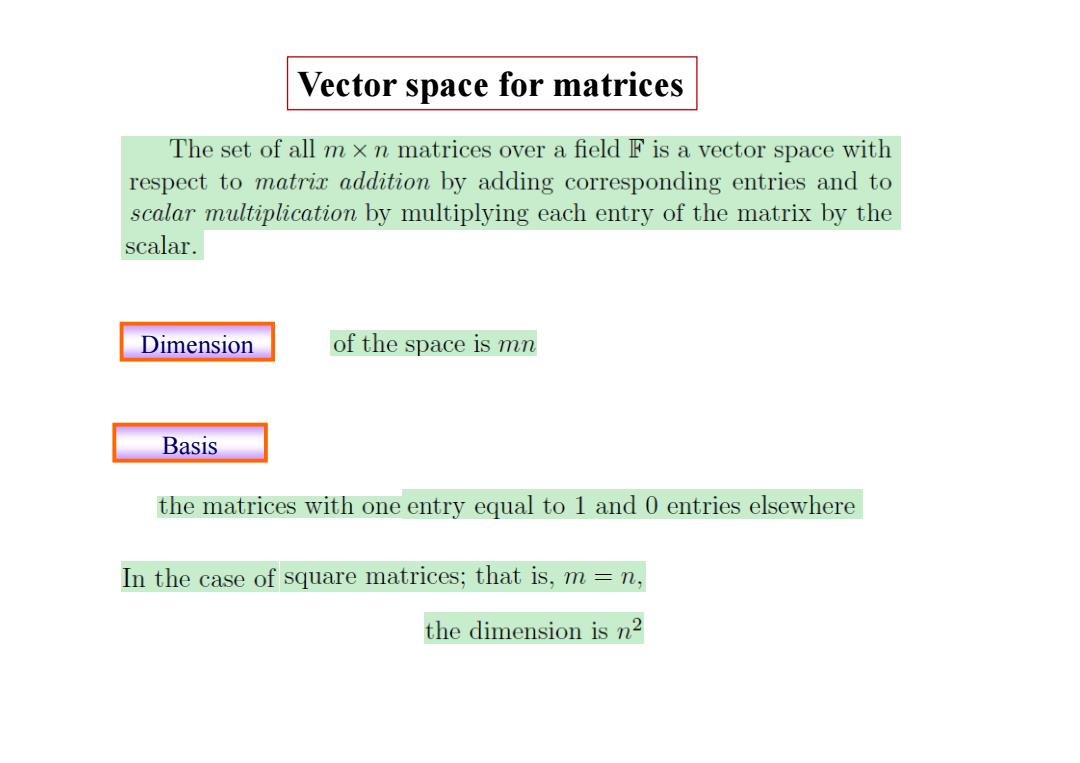
Vector space for matrices The set of all m x n matrices over a field F is a vector space with respect to matrix addition by adding corresponding entries and to scalar multiplication by multiplying each entry of the matrix by the scalar. Dimension of the space is mn Basis the matrices with one entry equal to 1 and 0 entries elsewhere In the case of square matrices;that is,m =n, the dimension is n2
Vector space for matrices Dimension Basis
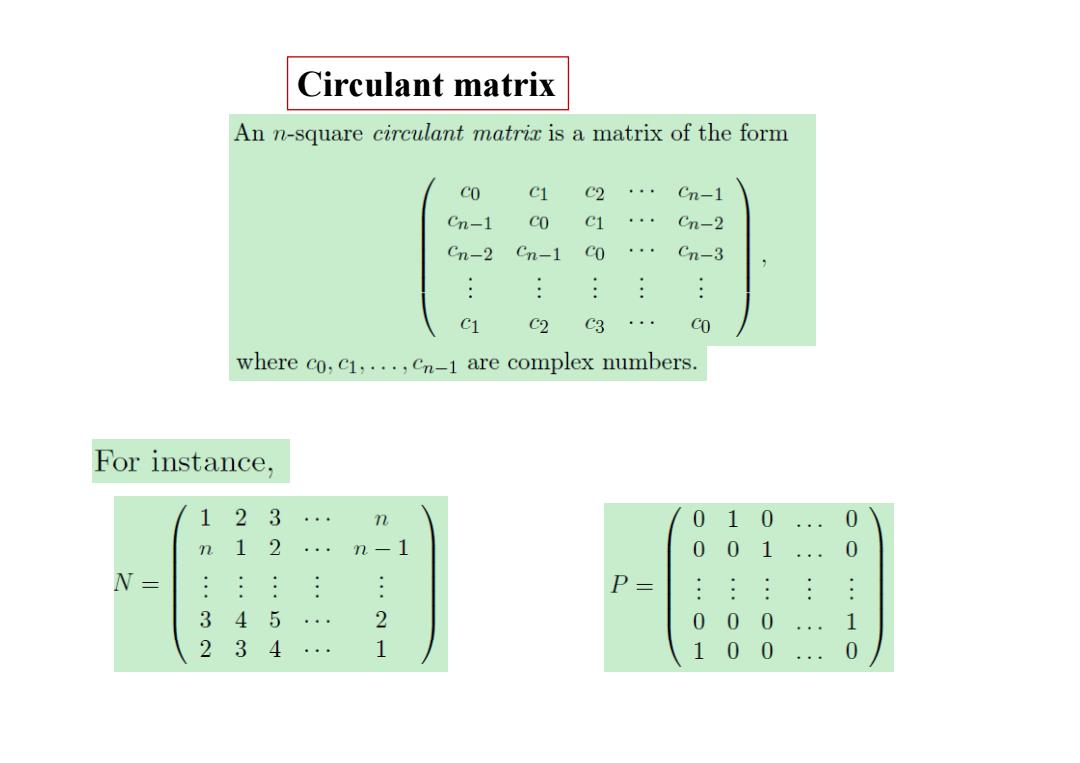
Circulant matrix An n-square circulant matrix is a matrix of the form Co C1 c2 Cn-1 Cn-1 CO c1 Cn-2 Cn-2 Cn-1 C0 Cn-3 C1 C2 C3·· Co where co,c1,...,cn-1 are complex numbers. For instance, 1 23 n 0 1 0 0 n 12 n-1 0 0 1 0 N= .. P= : 3 4 6 2 0 0 0 1 2 34 1 1 0 0 0
Circulant matrix
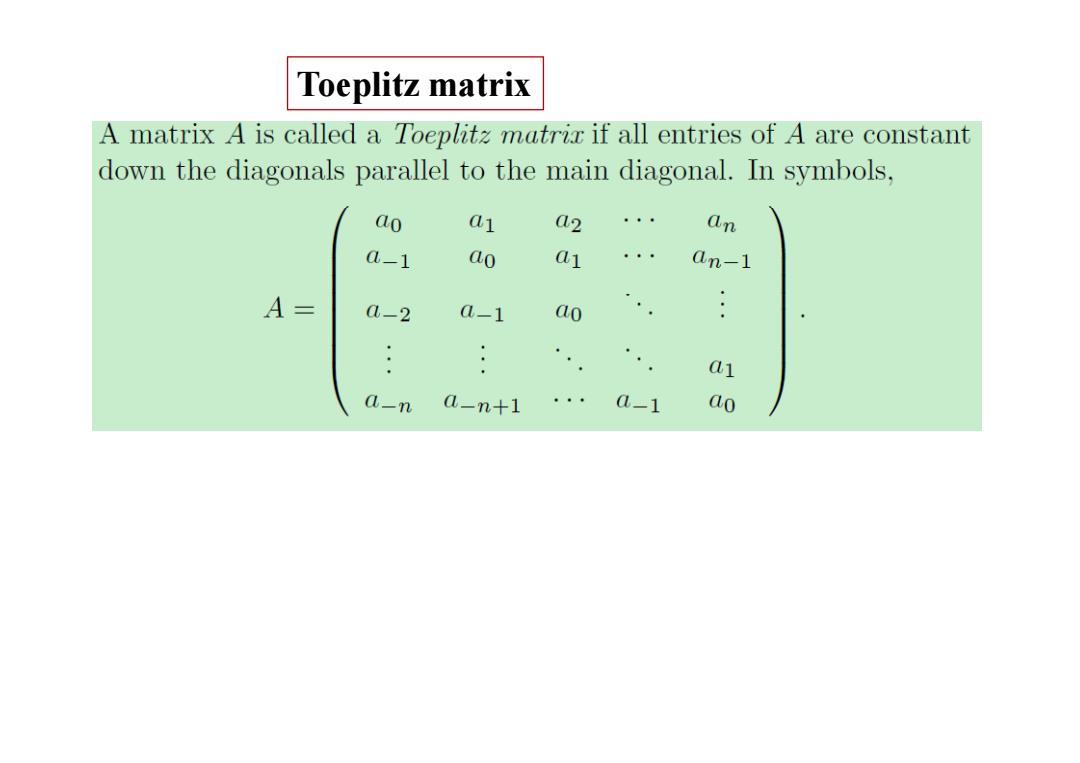
Toeplitz matrix A matrix A is called a Toeplitz matrix if all entries of A are constant down the diagonals parallel to the main diagonal.In symbols, ao al a2 an a-1 ao ai an-1 A= a-2 a-1 ao al a-n a-n+1 a-1 ao
Toeplitz matrix

Example of Toeplitzmatrix F=(fii)with fi+=1,i=1,2,...,n-1,and 0 elsewhere, is a Toeplitz matrix. Show that (i)a matrix A is a Toeplitz matrix if and only if A can be written in the form A=∑a-k(Fr)+∑。 k k=1 k三0 (ii)the sum of two Toeplitz matrices is a Toeplitz matrix (iii) a circulant matrix is a Toeplitz matrix
Example of Toeplitzmatrix

F=(fii)with fi.+1=1,i=1,2,...,n-1,and 0 elsewhere, is a Toeplitz matrix. A=∑a-k(FT)h+∑aFh k=1 k=0 (iv)BA is a symmetric matrix, known as a Hankel matriz,where B is the backward identity
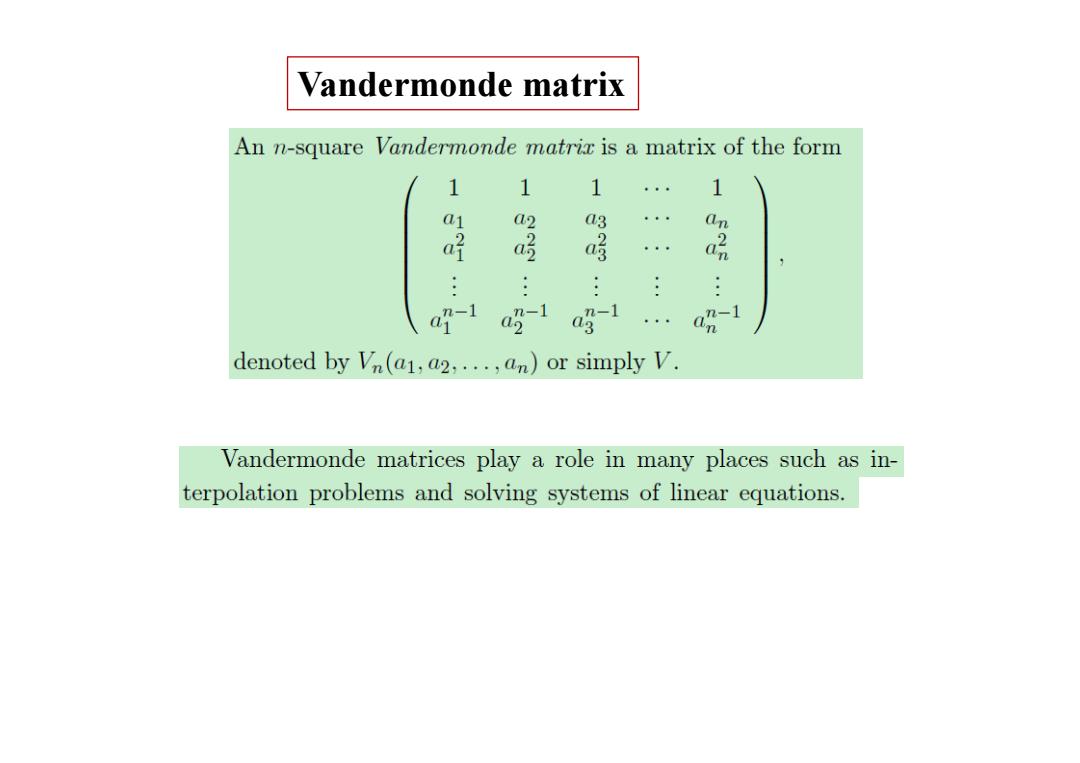
Vandermonde matrix An n-square Vandermonde matrix is a matrix of the form 1 1 1 1 a a3 a喝 a喝 a . ,n-1 ,n-1 on-1 0 denoted by Vn(a1,a2,...,an)or simply V. Vandermonde matrices play a role in many places such as in- terpolation problems and solving systems of linear equations
Vandermonde matrix