上海交通大学：《材料热力学 Thermodynamics of materials》课程教学资源（课件讲稿）lecture 5 property relation（1/2）

Contents of Today S.J.T.0. Phase Transformation and Applications Review previous Second law Functions F and G Property relation Property relation derived from U,H.F,and G etc. SJTU Physical Chemistry of Materials Spring2008©X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I Contents of Today Review previous Second law Functions F and G Property relation Property relation derived from U, H, F, and G etc

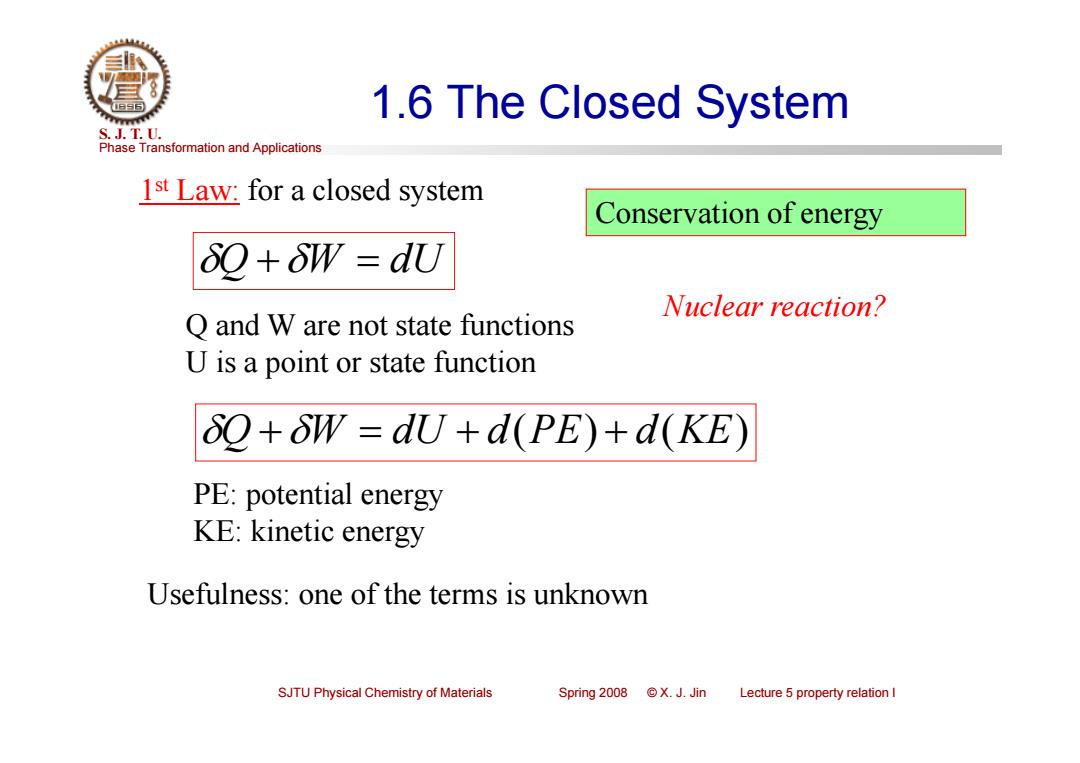
1.6 The Closed System S.J.T.0. Phase Transformation and Applications 1st Law:for a closed system Conservation of energy 6Q+δW=dU Nuclear reaction? Q and W are not state functions U is a point or state function 2+δW=dU+d(PE)+d(KE) PE:potential energy KE:kinetic energy Usefulness:one of the terms is unknown SJTU Physical Chemistry of Materials Spring2008©X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I 1.6 The Closed System 1st Law: for a closed system Q W  dU Q and W are not state functions U is a point or state function Q W  dU  d(PE)  d(KE) PE: potential energy KE: kinetic energy Usefulness: one of the terms is unknown Conservation of energy Nuclear reaction?

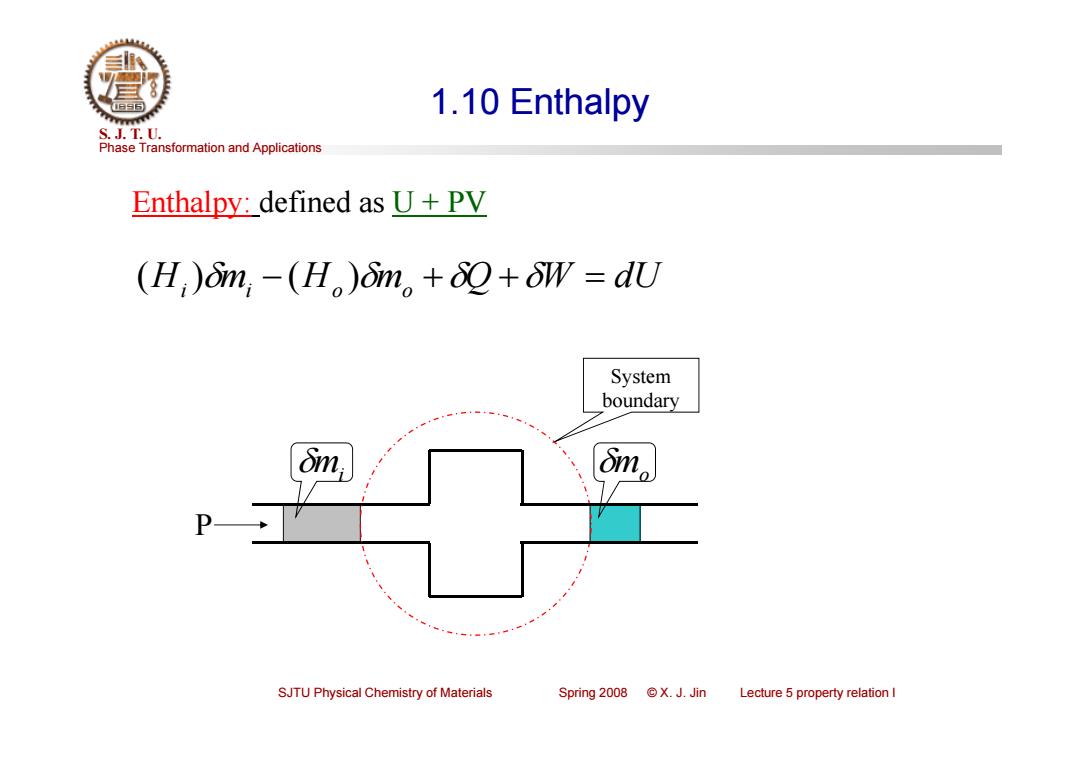
1.10 Enthalpy S.J.T.0. Phase Transformation and Applications Enthalpy:defined as U+PV (H;)6im,-(H。)n。+2+oW=dU System boundary SJTU Physical Chemistry of Materials Spring2008©X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I 1.10 Enthalpy (Hi)mi  (Ho )mo Q W  dU Enthalpy: defined as U + PV System boundary P mi mo

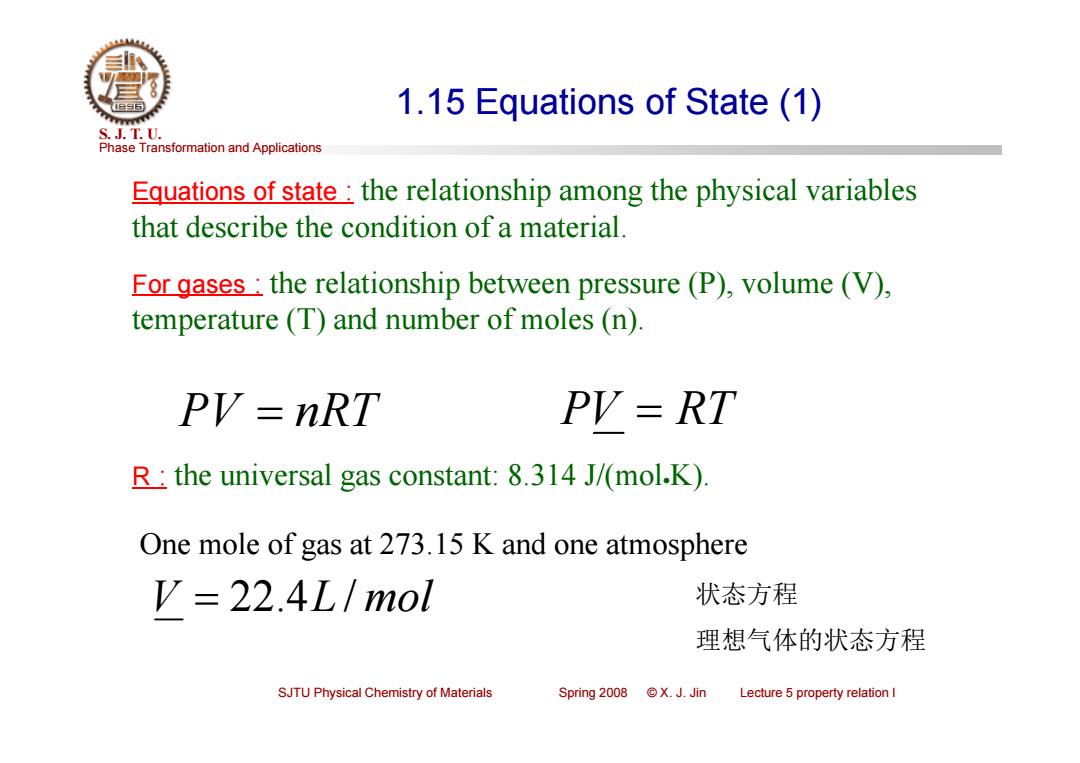
1.15 Equations of State (1) S.J.T.0. Phase Transformation and Applications Equations of state:the relationship among the physical variables that describe the condition of a material. For gases:the relationship between pressure(P),volume (V), temperature (T)and number of moles (n). PV=nRT PV=RT R:the universal gas constant:8.314 J/(mol.K) One mole of gas at 273.15 K and one atmosphere V=22.4L/mol 状态方程 理想气体的状态方程 SJTU Physical Chemistry of Materials Spring 2008 X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I 1.15 Equations of State (1) Equations of state : the relationship among the physical variables that describe the condition of a material. For gases : the relationship between pressure (P), volume (V), temperature (T) and number of moles (n). PV  nRT PV  RT R : the universal gas constant: 8.314 J/(molK). V  22.4L / mol One mole of gas at 273.15 K and one atmosphere 状态方程 理想气体的状态方程

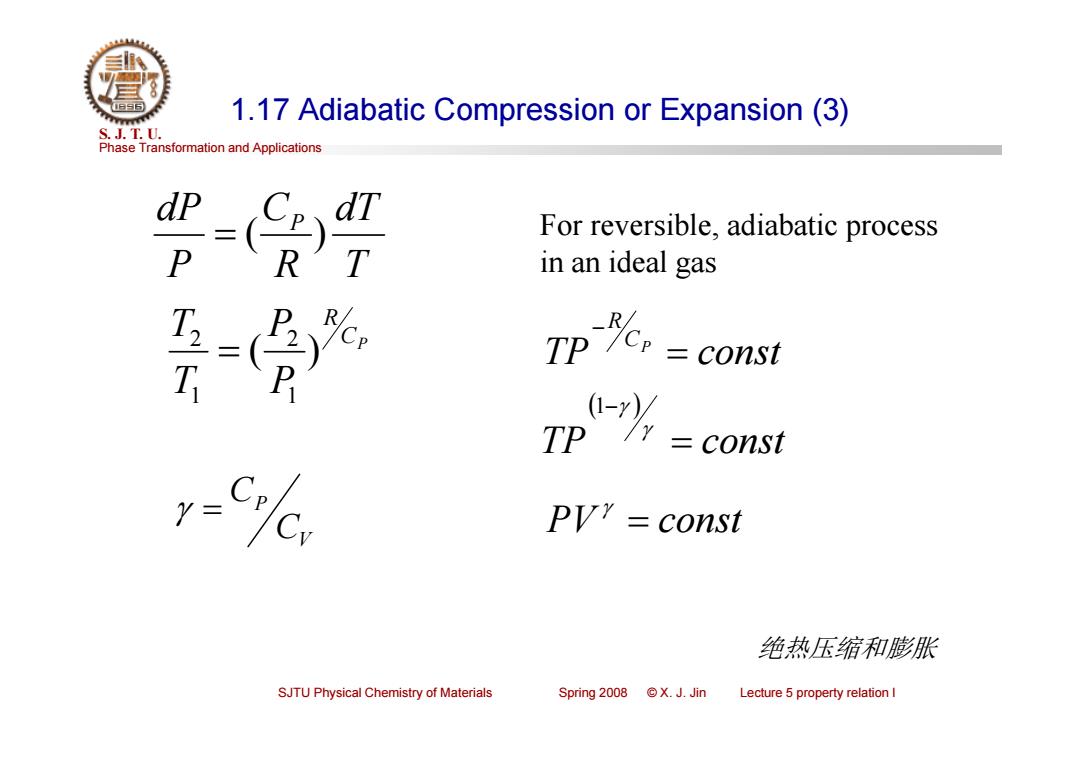
1.17 Adiabatic Compression or Expansion(3) S.J.T.0. Phase Transformation and Applications dl For reversible,adiabatic process in an ideal gas T 7p% T const const PV?=const 绝热压缩和膨胀 SJTU Physical Chemistry of Materials Spring 2008 X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I 1.17 Adiabatic Compression or Expansion (3) T dT R C P dP P  ( ) CP R P P T T ( ) 1 2 1 2  For reversible, adiabatic process in an ideal gas TP const CP R     TP  const   1  PV  const  V P C C   绝热压缩和膨胀

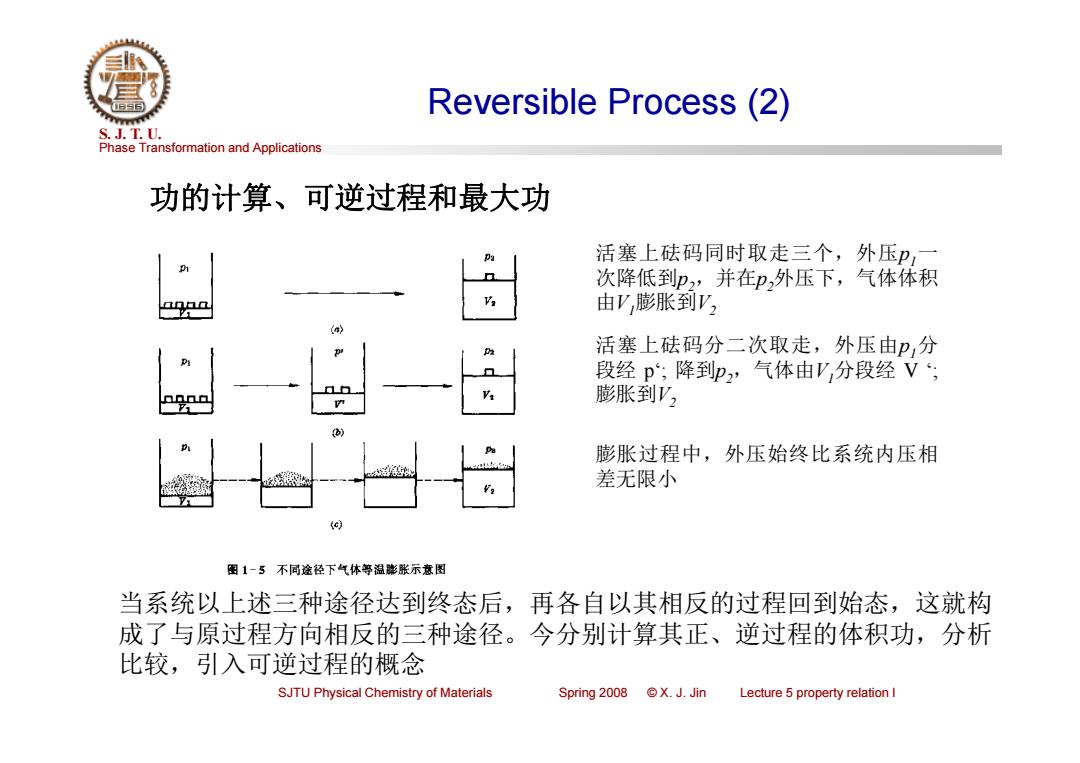
Reversible Process(2) S.J.T.0. Phase Transformation and Applications 功的计算、可逆过程和最大功 活塞上砝码同时取走三个，外压P,一 次降低到p2,并在p,外压下，气体体积 t口aa 由V,膨胀到V, 活塞上砝码分二次取走，外压由卫，分 段经p,降到p2,气体由'，分段经V； ona▣ 膨胀到V2 膨胀过程中，外压始终比系统内压相 差无限小 图1-5不同途径下气体等温膨张示意图 当系统以上述三种途径达到终态后，再各自以其相反的过程回到始态，这就构 成了与原过程方向相反的三种途径。今分别计算其正、逆过程的体积功，分析 比较，引入可逆过程的概念 SJTU Physical Chemistry of Materials Spring2008©X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I Reversible Process (2) 功的计算、可逆过程和最大功 活塞上砝码同时取走三个，外压p1一 次降低到p2，并在p2外压下，气体体积 由V1膨胀到V2 活塞上砝码分二次取走，外压由p1分 段经 p‘; 降到p2，气体由V1分段经 V ‘; 膨胀到V2 膨胀过程中，外压始终比系统内压相 差无限小 当系统以上述三种途径达到终态后，再各自以其相反的过程回到始态，这就构 成了与原过程方向相反的三种途径。今分别计算其正、逆过程的体积功，分析 比较，引入可逆过程的概念

Reversible Process (7) S.J.T.0. Phase Transformation and Annlicatinns 妻1-1不同途径功的比较 途 径 I及I 亚及I Ⅲ及I' W正 -1.87(I) -2.49(亚) -3.46(正) W/KJ W逆 +7.48(1') +4.99(1') +3.46(Ⅲ') W正+W递 +5.61 +2.50 0 由表可知系统由一个状态变化到另一状态，可以通过不同的途径来实现。 当系统状态变化后，再使系统恢复到始态，环境不一定能复原，只有途径 Ⅲ，当系统复原时环境中没有留下功变热的痕迹，此过程为可逆过程。而 另一种情况是系统经过途径、Ⅱ后，系统虽复原，但环境中留下功变热的 痕迹，途径I、Ⅱ为不可逆过程。 当系统由始态I经过某一过程变到终态Ⅱ后，如能使系统再回到原态，同时 也消除了原过程对环境所产生的一切影响，则原来过程称为可逆过程。 反之，某过程进行后，如果用任何方法都不可能使系统和环境完全复原， 则此过程称为不可逆过程。 SJTU Physical Chemistry of Materials Spring2008©X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I Reversible Process (7) 由表可知系统由一个状态变化到另一状态，可以通过不同的途径来实现。 当系统状态变化后，再使系统恢复到始态，环境不一定能复原，只有途径 III，当系统复原时环境中没有留下功变热的痕迹，此过程为可逆过程。而 另一种情况是系统经过途径I、II后，系统虽复原，但环境中留下功变热的 痕迹，途径I、II为不可逆过程。 当系统由始态I经过某一过程变到终态II后，如能使系统再回到原态，同时 也消除了原过程对环境所产生的一切影响，则原来过程称为可逆过程。 反之，某过程进行后，如果用任何方法都不可能使系统和环境完全复原， 则此过程称为不可逆过程

Heat Engine S.J.T.0. Phase Transformation and Applications A D D B B Pressure OA C Volume SJTU Physical Chemistry of Materials Spring 2008 X.J.Jin Lecture 5 property relation I

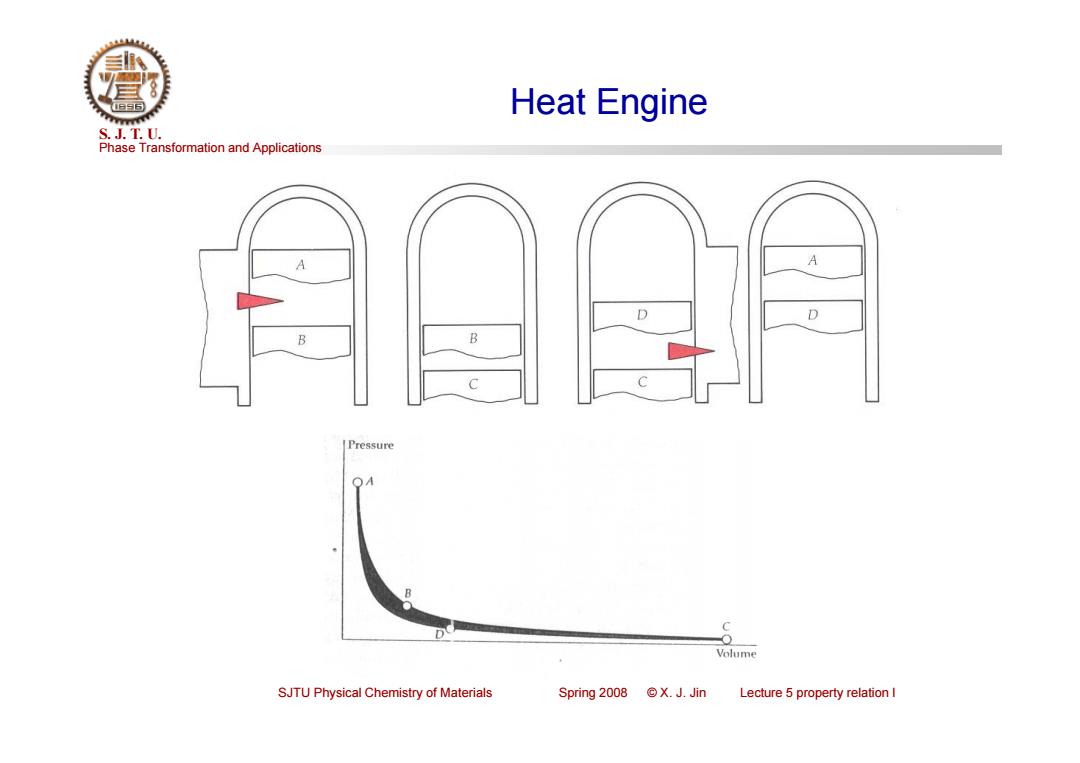
Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I Heat Engine

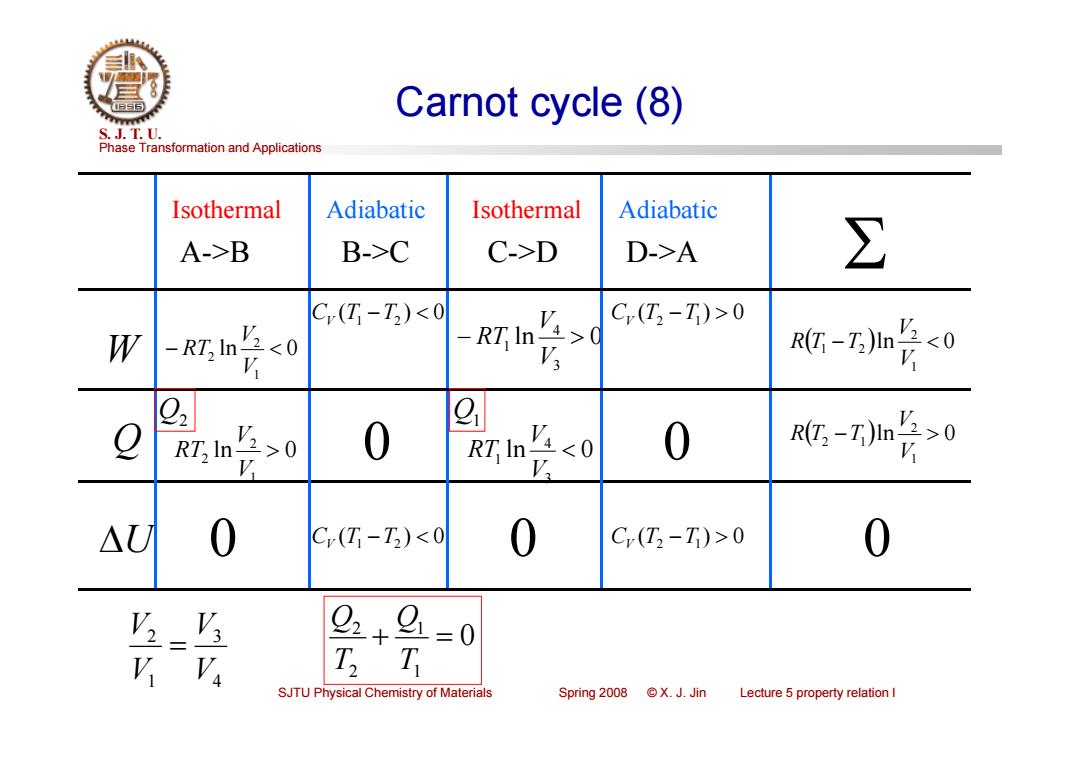
Carnot cycle (8) S.J.T.0. Phase Transformation and Applications Isothermal Adiabatic Isothermal Adiabatic A->B B->C C->D D->A ∑ C(T-T)0 W -RT In V20 RT In V Rt-Z)n点0 0 RT In V0 0 :-7m长0 △U 0 C(T-T)0 0 +9 =0 SJTU Physical Chemistry of Materials Spring2008©X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I Carnot cycle (8) W ln 0 3 4 1  V V Q ln 0 RT 1 2 2  V V RT ln 0 1 2  2  V V RT U 0 A->B B->C C->D D->A 0 Isothermal Isothermal Adiabatic Adiabatic ln 0 3 4  1  V V RT 0 0 CV (T1 T2 )  0 CV (T2 T1)  0 CV (T1 T2 )  0 CV (T2 T1)  0  0  ln 0 1 2 1  2  V V R T T  ln 0 1 2 2  1  V V R T T Q2 Q1 0 1 1 2 2   T Q T Q 4 3 1 2 V V V V 

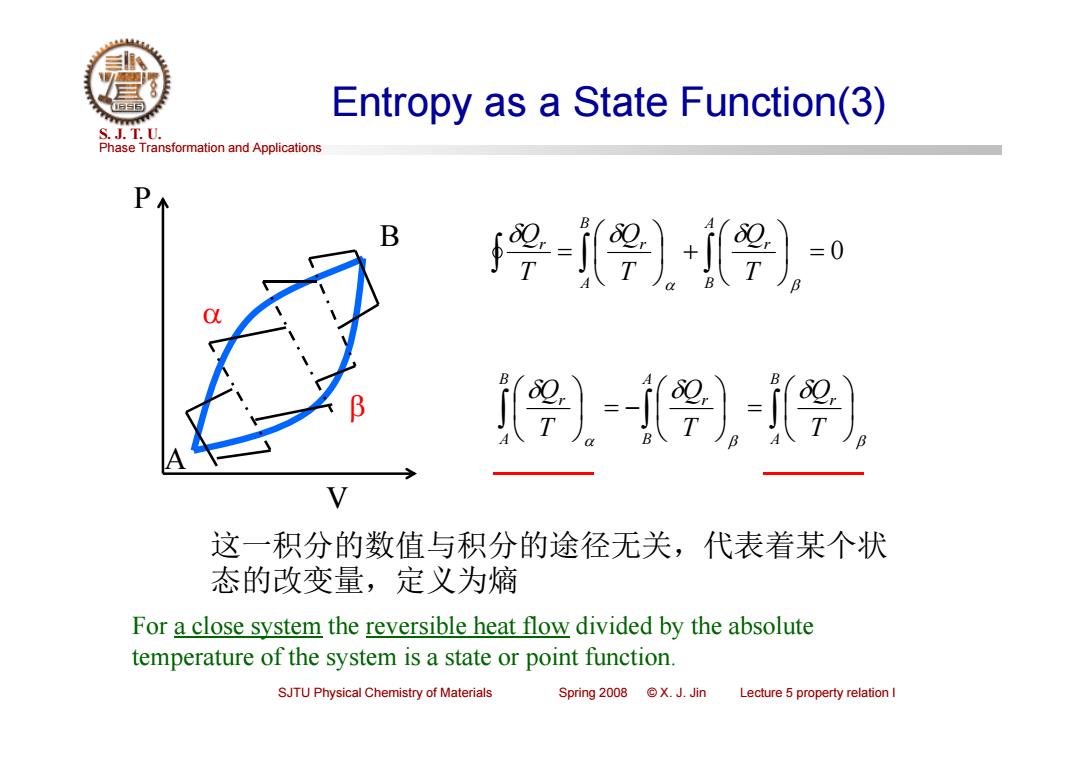
Entropy as a State Function(3) S.J.T.0. Phase Transformation and Applications -))= i〔婴)-婴-i婴) 这一积分的数值与积分的途径无关，代表着某个状 态的改变量，定义为熵 For a close system the reversible heat flow divided by the absolute temperature of the system is a state or point function. SJTU Physical Chemistry of Materials Spring2008©X.J.Jin Lecture 5 property relation I

Phase Transformation and Applications S. J. T. U. SJTU Physical Chemistry of Materials Spring 2008 © X. J. Jin Lecture 5 property relation I Entropy as a State Function(3)   0                AB r BA r r TQ TQ TQ                     BA r AB r BA r TQ TQ TQ P V A B   这一积分的数值与积分的途径无关，代表着某个状 态的改变量，定义为熵 For a close system the reversible heat flow divided by the absolute temperature of the system is a state or point function