西安电子科技大学：《场论与复变函数》课程教学资源（课件讲义）第四章 级数 Series

第四章级数 历些毛子种技大学 XIDIAN UNIVERSITY Series 第一节 复数项级数 第二节 幂级数 第三节 泰勒级数 第四节 罗朗级数 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 2 第一节 复数项级数 第二节 幂级数 第三节 泰勒级数 第四节 罗朗级数 第四章 级数 Series

第四章级数 历些毛子代枚大票 XIDIAN UNIVERSITY Series 第一节 复数项级数 四1.复数列的极限 日2.级数的概念 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 3  1. 复数列的极限  2. 级数的概念 第一节 复数项级数 第四章 级数 Series

第四章级数 历安毛子代枝大学 XIDIAN UNIVERSITY Series 一.复数列极限 定义 已知复数列{cn}={an+bn}(n=1,2) a=a+b为复常数. 若Ve>0,N>0,)n>N,恒有an-<6, 那么a称为复数列an当n→o时的极限， 记作lima=a,或当n→o时，an→o, 此时，也称复数bn收敛于a. 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 4 一.复数列极限 定义 已知复数列 ， n n n     a ib n 1,2    a ib    为复常数. 那 么 称为复数列 当 时的极限， 若 恒 有 ，           n N n N n n { } 0, 0, ,       { } . lim , ,       此时，也称复数列 收敛于 记 作 或 当 时 ， n n n n  n     第四章 级数 Series

第四章级数 历些毛子代枚大票 XIDIAN UNIVERSITY Series 定理1（数列收敛的充要条件） 数列{an}收敛于a合lima,=a,limb=b 证明 →.e>O,3N,当n>N时，an-a<8 即(an+ib)-(a+ib)=（a。-a+i(b。-b<ε 又lan-d≤an-a<e, bn-bl≤an-d<e ∴.lima,=a,libn=b n-→oo 11)o0 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 5 数列 收敛于  n n n  n n α α lima a, lim b b      n 又 a a  n n n n lima a, limb b      证明 定理1(数列收敛的充要条件) n        ε 0, N n N α α ε ,当 时，     n n 即 a ib a ib        a a i b b n n     ε n n b b     α α ε  ε,   αn α 第四章 级数 Series

第四章级数 历安毛子代枝大学 XIDIAN UNIVERSITY Series 定理1（数列收敛的充要条件） 数列{an}收敛于a令lima=a,limb=b 1->co 证明←←lima=4,libn=b n->oo n->oo ∴.Ve>0,3N,当n>N时， a-aa∞ 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 6 数列 收敛于  n n n  n n α α lima a, lim b b      证明 定理1(数列收敛的充要条件) n n n n lima a, limb b           0 , N , n N 当 时，        α α a a b b ε n n n n n limα α  即  2 2 a a , b b n n       第四章 级数 Series

第四章级数 历些毛子代枚大学 XIDIAN UNIVERSITY Series 定理1（数列收敛的充要条件） 数列{an}收敛于a台lima=a,limb=b 00 定理一说明：可将复数列的敛散性转化为 判别两个实数列的敛散性： 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 7 数列 收敛于  n n n  n n α α lima a, lim b b      定理1(数列收敛的充要条件) 定理一说明: 可将复数列的敛散性转化为 判别两个实数列的敛散性. 第四章 级数 Series

第四章级数 历些毛子代枚大学 XIDIAN UNIVERSITY Series 课堂练习： 下列数列是否收敛？如果收敛，求出其极限 1+i (1)zm= 1-i (2)zn=(-1)”+i +1 (4)zn=e2'; (5)zn= 2 n 场论与复变函数Field Theory and Complex Variable Functions

场论与复变函数 Field Theory and Complex Variable Functions 8 课堂练习: 下列数列是否收敛? 如果收敛, 求出其极限. ; 1 1 (1) ni ni zn    ; 1 (2) ( 1)     n i z n n 2 1 (5) . n i n z e n    (3) (1 ) . 3 n n i z    2 (4) ; n i n z e    第四章 级数 Series

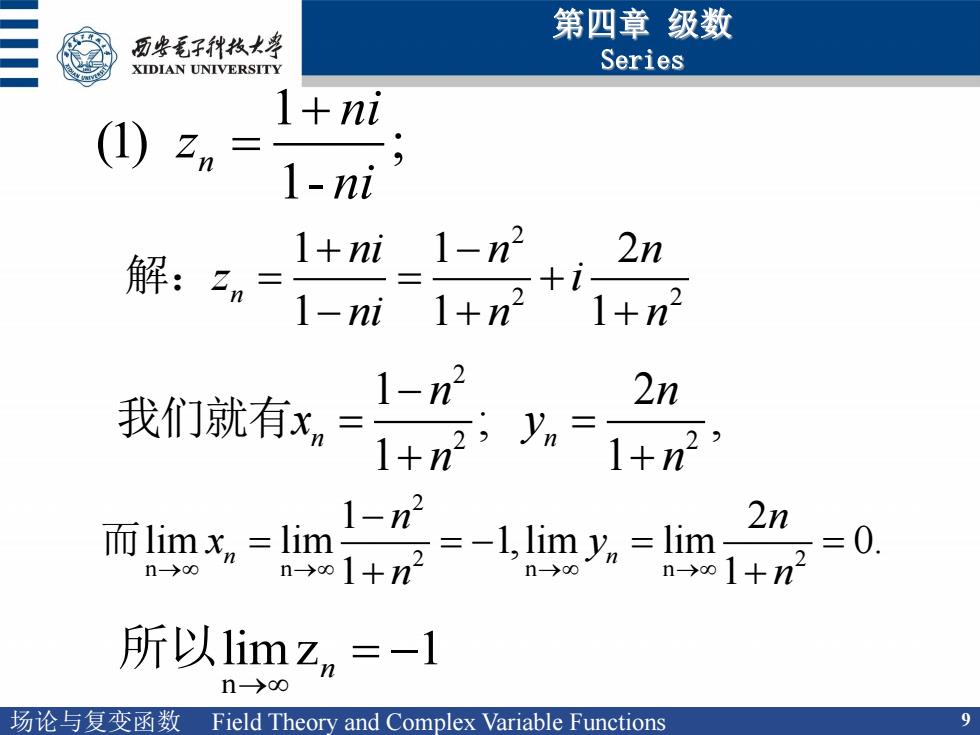
第四章级数 历些毛子代枚大票 XIDIAN UNIVERSITY Series I+ni (1) 2n 二 1-ni 2n 解：2n= 1+ni 1-n2 1-ni i++ 1+n 1-n2 我们就有xn 2n 1+ 1+n 1-n2 n 而limx,=lim 2 =-Llimy lim. =0 n→0 n-→o1+n n1+n2 所以1imzn=-l n>∞ 场论与复变函数Field Theory and Complex Variable Functions 9

场论与复变函数 Field Theory and Complex Variable Functions 9 2 2 2 1 1 2 1 1 1         n ni n n z i ni n n 解： 1 (1) ; 1 - n ni z ni   2 2 2 1 2 ; , 1 1      n n n n x y n n 我们就有 2 2 2 n n n n 1 2 lim lim 1,lim lim 0.     1 1         n n n n x y n n 而 n lim z 1 n  所以   第四章 级数 Series

第四章级数 历安毛子代枚大等 XIDIAN UNIVERSITY Series (2)zm=(-1)”+ n+ (3)n=(1+ -n 解9+ can eos(-名)+isin(-gxl 场论与复变函数Field Theory and Complex Variable Functions 10

场论与复变函数 Field Theory and Complex Variable Functions 10 (3) (1 ) . 3 n n i z   3 3 1 z ( ) 2 2 2 n n n i            解： 3 (cos sin ) 2 6 6 n n i              3 [cos( ) sin( )] 2 6 6 n n n   i             ; 1 (2) ( 1)     n i z n n 第四章 级数 Series

第四章级数 历安毛子代枚大学 XIDIAN UNIVERSITY Series 1 (3) 乙n=(1+ m,9r-98agn limx=0,lim y =0 n0 n->oc →limz=0, n→o 场论与复变函数Field Theory and Complex Variable Functions 11

场论与复变函数 Field Theory and Complex Variable Functions 11 (3) (1 ) . 3 n n i z   3 3 1 z ( ) 2 2 2 n n n i            解： 3 [cos( ) sin( )] 2 6 6 n n n   i             3 3 ( ) cos , ( ) sin 2 6 2 6 n n n n n n     x y   n n lim 0, lim 0 n n x y     n lim z 0, n    第四章 级数 Series