
《微分几何续论》课程教学大纲 课程基本信息(Course Information) 课程代码 学时 (Cours MATH4603 Code) Hours) *课程名称 (中文)微分几何续论 (Course Name) (英文)Differential Geometry II 课程类型本课程为数学学科各专业本科生的专业方向选修课。同时也可作为 (Course Type)物理学、力学等专业研究生的选修课。 授课对缘 (Target 数学系本科生,研究生:物理、力学等专业研究生 授课语言 (Language of中文、英文(依赖于学生的要求) Instruction) “开课院系 数学科学学院 (School) 先修课程 (Prerequisite初等微分几何,拓扑学 后续课程 (post) 黎曼几何 课程负责人 课程网址 杨义虎 (Course (Instructor) Wehnage) (中文300-500字,含课程性质、主要教学内容、课程学目标等 这是初等微分几何(3维欧氏空间中曲线与曲面的微分几何)的后续课程。本 课程主要讲授如下内容。 Pat上3维欧氏空间中曲线和曲面的整体理论,包括平面上的等周不等式以 及四顶点定理等:球面的刚性,Hilbert定理,关于常平均曲率闭曲面的Hop 定理和AleX androv定理 ,以及极小曲面和B nstein定理 课程简介(中Pat:这部分的内容将依赖于主讲人的选择学生的要求,这里建议三个课题 文)1)(非参数)正则曲面内蕴几何的整体方面(或两维黎曼几何):(非参数 (Description) 正则曲面和两维黎曼流形,黎曼联络和曲率,测地线和Hopf.-Rinow定理, acobi场和共轭点,第一、二变分公式和Bonnet-.Myers定理,Cartan-Hadama 定理,与Laplace算子相关的几何分析等 2)黎曼曲面初步 正则参数曲面或两维黎曼流形的(局部)等温坐标一复坐标 复结构(黎曼度量的共形类)和黎曼曲面,全纯函数(Cauchy-Riemann 程,d-bar算子),(反)全纯(余)切丛及全纯映射,一致化定理等 3)Alexandrov几何初步
《微分几何续论》课程教学大纲 课程基本信息(Course Information) 课程代码 (Course Code) MATH4603 *学时 (Credit Hours) 48 *学分 (Credits) 3 *课程名称 (Course Name) (中文)微分几何续论 (英文)Differential Geometry II 课程类型 (Course Type) 本课程为数学学科各专业本科生的专业方向选修课。同时也可作为 物理学、力学等专业研究生的选修课。 授课对象 (Target Audience) 数学系本科生,研究生;物理、力学等专业研究生 授课语言 (Language of Instruction) 中文、英文(依赖于学生的要求) *开课院系 (School) 数学科学学院 先修课程 (Prerequisite ) 初等微分几何,拓扑学 后续课程 (post) 黎曼几何 *课程负责人 (Instructor) 杨义虎 课程网址 (Course Webpage) *课程简介(中 文) (Description) (中文 300-500 字,含课程性质、主要教学内容、课程教学目标等) 这是初等微分几何(3 维欧氏空间中曲线与曲面的微分几何)的后续课程。本 课程主要讲授如下内容。 Part I: 3 维欧氏空间中曲线和曲面的整体理论, 包括平面上的等周不等式以 及四顶点定理等;球面的刚性,Hilbert 定理,关于常平均曲率闭曲面的 Hopf 定理和 Alexandrov 定理,以及极小曲面和 Bernstein 定理。 Part II:这部分的内容将依赖于主讲人的选择学生的要求,这里建议三个课题。 1) (非参数)正则曲面内蕴几何的整体方面(或两维黎曼几何):(非参数) 正则曲面和两维黎曼流形,黎曼联络和曲率,测地线和 Hopf-Rinow 定理, Jacobi场和共轭点,第一、二变分公式和Bonnet-Myers定理,Cartan-Hadamard 定理,与 Laplace 算子相关的几何分析等; 2) 黎曼曲面初步:正则参数曲面或两维黎曼流形的(局部)等温坐标--复坐标, 复结构(黎曼度量的共形类)和黎曼曲面,全纯函数(Cauchy-Riemann 方 程, d-bar 算子),(反)全纯(余)切丛及全纯映射,一致化定理等 3) Alexandrov 几何初步
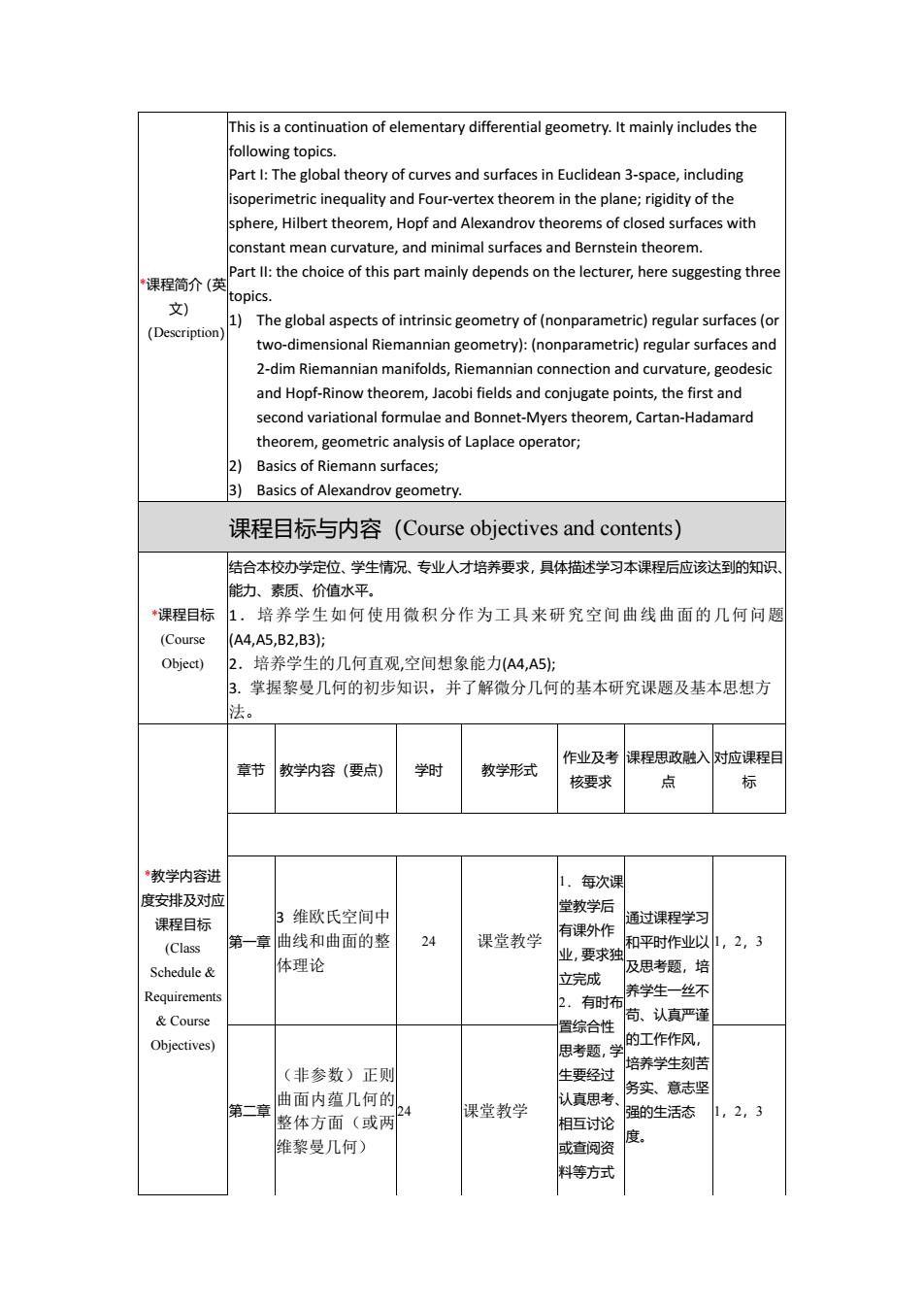
This elementary differential geometry Itmainly incudes the folowing top Part :The global theory of curves and surfaces in Euclidean 3-space,including isoperimetric inequality and Four-vertex theorem in the plane;rigidity of the sphere,Hilbert theorem,Hopf and Alexandrov theorems of closed surfaces with constant mean curvature,and minimal surfaces and Bernstein theorem. 课程简介( Part l:the choice of this part mainly dependson the lecturer,here suggesting thre 文) 1)The global aspects of intrinsic geometry of (nonparametric)regular surfaces(or (Description) two-dimensional Riemannian geometry):(nonparametric)regular surfaces and 2-dim Riemannian manifolds,Riemannian connection and curvature,geodesic and Hopf-Rinow theorem,Jacobi fields and conjugate points,the first and secnd variational for and Bonnet-Myers theorem,Cartan-Hadamard theorem,geometric analysis of Laplace operator; 2)Basics of Riemann surfaces; 3)Basics of Alexandrov geometry. 课程目标与内容(Course objectives and contents) 结合本校办学定位、学生情况、专业人才培养要求,具体描述学习本课程后应该达到的知识, 能力、素质。价值水平。 课程标1.培养学生如何使用微积分作为工具来研究空间曲线曲面的几何问题 A4,A5,B2,B3 2.培养学生的几何直观,空间想象能力A4,A5: 3.掌握黎曼几何的初步知识,并了解微分几何的基本研究课题及基本思想方 法。 章节教学内容(要点) 学时 作业及考课程思政融入对应课程目 救学形式 核要求 点 *教学内容进 1,每次课 度安排及对应 课程目标 3维欧氏空间叶 通过课程学 (Class 第一章曲线和曲面的整 课堂教学 有课外州 和平时作业 2,3 业,要求 Schedule& 体理论 及思考题,培 立完成 Requirement 养学生一丝不 2.有时 &Course 置综合性 简、认严道 Objectives) 思考 的工作作风 (非参数)正则 培养学生 生要经 务实、意志些 认真思考 第二章 曲面内蕴几何的 棵堂教学 强的生活态 ,2.3 整体方面(或两 相互讨论 维黎曼几何) 或音阅溶 料等方式
*课程简介(英 文) (Description) This is a continuation of elementary differential geometry. It mainly includes the following topics. Part I: The global theory of curves and surfaces in Euclidean 3-space, including isoperimetric inequality and Four-vertex theorem in the plane; rigidity of the sphere, Hilbert theorem, Hopf and Alexandrov theorems of closed surfaces with constant mean curvature, and minimal surfaces and Bernstein theorem. Part II: the choice of this part mainly depends on the lecturer, here suggesting three topics. 1) The global aspects of intrinsic geometry of (nonparametric) regular surfaces (or two-dimensional Riemannian geometry): (nonparametric) regular surfaces and 2-dim Riemannian manifolds, Riemannian connection and curvature, geodesic and Hopf-Rinow theorem, Jacobi fields and conjugate points, the first and second variational formulae and Bonnet-Myers theorem, Cartan-Hadamard theorem, geometric analysis of Laplace operator; 2) Basics of Riemann surfaces; 3) Basics of Alexandrov geometry. 课程目标与内容(Course objectives and contents) *课程目标 (Course Object) 结合本校办学定位、学生情况、专业人才培养要求,具体描述学习本课程后应该达到的知识、 能力、素质、价值水平。 1.培养学生如何使用微积分作为工具来研究空间曲线曲面的几何问题 (A4,A5,B2,B3); 2.培养学生的几何直观,空间想象能力(A4,A5); 3. 掌握黎曼几何的初步知识,并了解微分几何的基本研究课题及基本思想方 法。 *教学内容进 度安排及对应 课程目标 (Class Schedule & Requirements & Course Objectives) 章节 教学内容(要点) 学时 教学形式 作业及考 核要求 课程思政融入 点 对应课程目 标 第一章 3 维欧氏空间中 曲线和曲面的整 体理论 24 课堂教学 1.每次课 堂教学后 有课外作 业,要求独 立完成 2.有时布 置综合性 思考题,学 生要经过 认真思考、 相互讨论 或查阅资 料等方式 通过课程学习 和平时作业以 及思考题,培 养学生一丝不 苟、认真严谨 的工作作风, 培养学生刻苦 务实、意志坚 强的生活态 度。 1,2,3 第二章 (非参数)正则 曲面内蕴几何的 整体方面(或两 维黎曼几何) 24 课堂教学 1,2,3
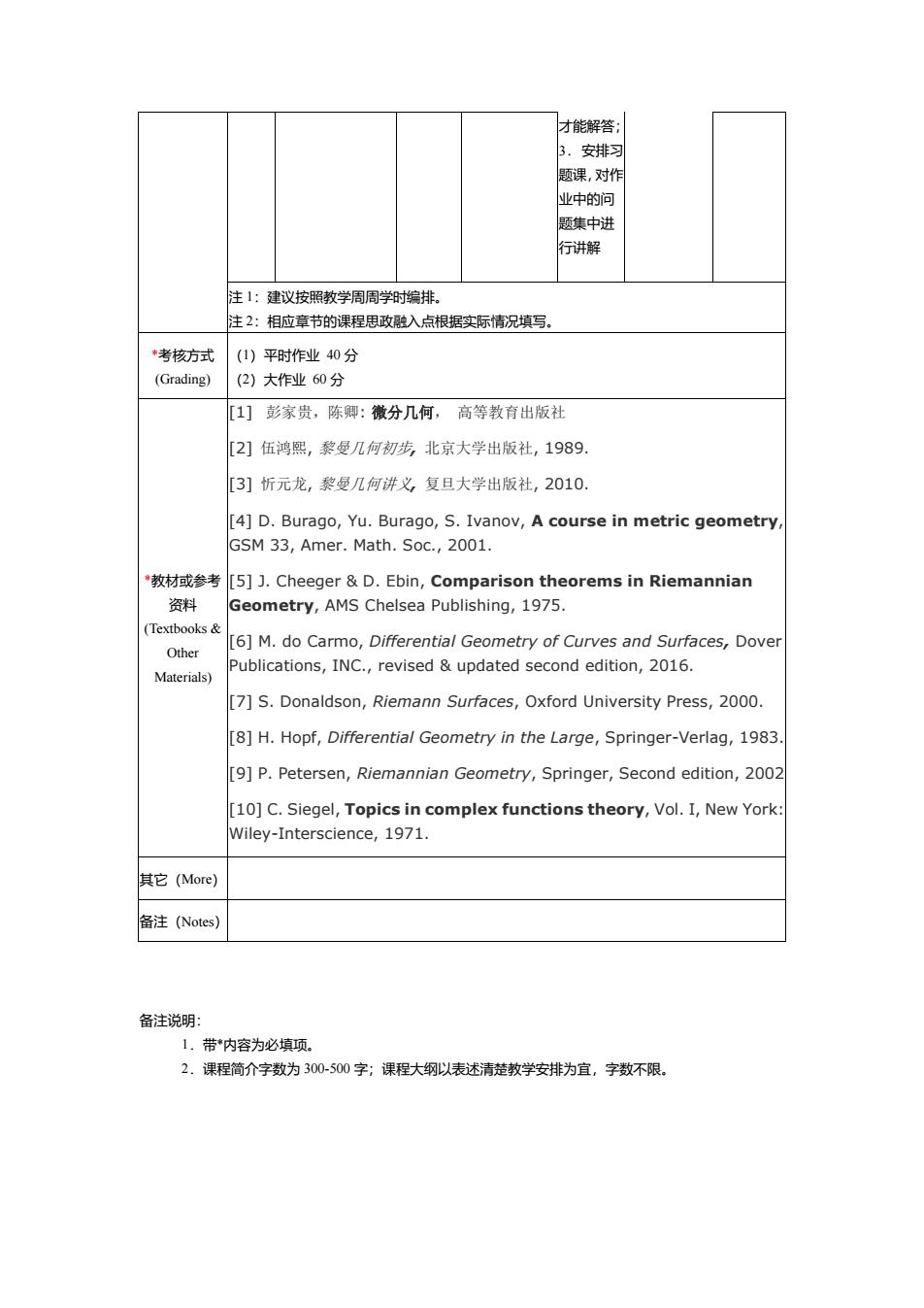
才能解答: 安排 题课,对 业中的问 频生中讲 行讲解 注1:建议技照牧学周周学时编排。 注2:相应章节的课程思政融入点根据实际情况填与。 考核方式(1)平时作业40分 (Grading)(2)大作y业60分 [1]彭家贵,陈卿:徽分几何,高等教育出版社 2]伍鸿熙,黎曼儿何初步北京大学出版社,1989。 [3]忻元龙,黎曼几何讲义复旦大学出版社,2010 [4]D.Burago,Yu.Burago,S.Ivanov,A course in metric geometry. GSM 33,Amer.Math.Soc.,2001. *教材或参考[5]J.Cheeger&D.Ebin,Comparison theorems in Riemannian 资料 Geometry,AMS Chelsea Publishing,1975 (Textbooks Other [6]M.do Carmo,Differential Geometry of Curves and Surfaces,Dover Materials) Publications,INC.,revised updated second edition,2016. [7]S.Donaldson,Riemann Surfaces,Oxford University Press,2000. []H.Hopf,Differential Geometry in the Large,Springer-Verlag,1983 [9]P.Petersen,Riemannian Geometry,Springer,Second edition,200 [10]C.Siegel,Topics in complex functions theory,Vol.I,New York: Wiley-Interscience,1971. 其它(More) 备注(Notes) 备注说明: 1.带内容为必填项。 2.课程简介字数为300500字:课程大纲以表述清楚教学安排为宜,字数不限
才能解答; 3.安排习 题课,对作 业中的问 题集中进 行讲解 注 1:建议按照教学周周学时编排。 注 2:相应章节的课程思政融入点根据实际情况填写。 *考核方式 (Grading) (1)平时作业 40 分 (2)大作业 60 分 *教材或参考 资料 (Textbooks & Other Materials) [1] 彭家贵,陈卿: 微分几何, 高等教育出版社 [2] 伍鸿熙, 黎曼几何初步, 北京大学出版社, 1989. [3] 忻元龙, 黎曼几何讲义, 复旦大学出版社, 2010. [4] D. Burago, Yu. Burago, S. Ivanov, A course in metric geometry, GSM 33, Amer. Math. Soc., 2001. [5] J. Cheeger & D. Ebin, Comparison theorems in Riemannian Geometry, AMS Chelsea Publishing, 1975. [6] M. do Carmo, Differential Geometry of Curves and Surfaces, Dover Publications, INC., revised & updated second edition, 2016. [7] S. Donaldson, Riemann Surfaces, Oxford University Press, 2000. [8] H. Hopf, Differential Geometry in the Large, Springer-Verlag, 1983. [9] P. Petersen, Riemannian Geometry, Springer, Second edition, 2002 [10] C. Siegel, Topics in complex functions theory, Vol. I, New York: Wiley-Interscience, 1971. 其它(More) 备注(Notes) 备注说明: 1.带*内容为必填项。 2.课程简介字数为 300-500 字;课程大纲以表述清楚教学安排为宜,字数不限