
习题课函数与极限
习题课 函数与极限

极限1.极限定义2.极限运算法则3.无穷小常用等价无穷小:x→01- cos x ~ ix2sinx~xtanx~xIn(1+x)~xarctanx~xarcsin x ~ xer-1~xa-1~xlna(1+x)"-1~μux4.极限存在准则及两个重要极限sinx(1+=lim7/lim=1Xx-0xr85.连续
极限 1. 极限定义 2. 极限运算法则 3. 无穷小 常用等价无穷小: 𝑥 → 0 sin x ~ x 1− cos x ~ 2 2 1 x arcsin x ~ x e −1~ x x (1+ ) −1~ x x 4.极限存在准则及两个重要极限 lim 𝑥→0 𝑠𝑖𝑛𝑥 𝑥 = 1 lim 𝑥→∞ 1 + 1 𝑥 𝑥 = 𝑒 5.连续
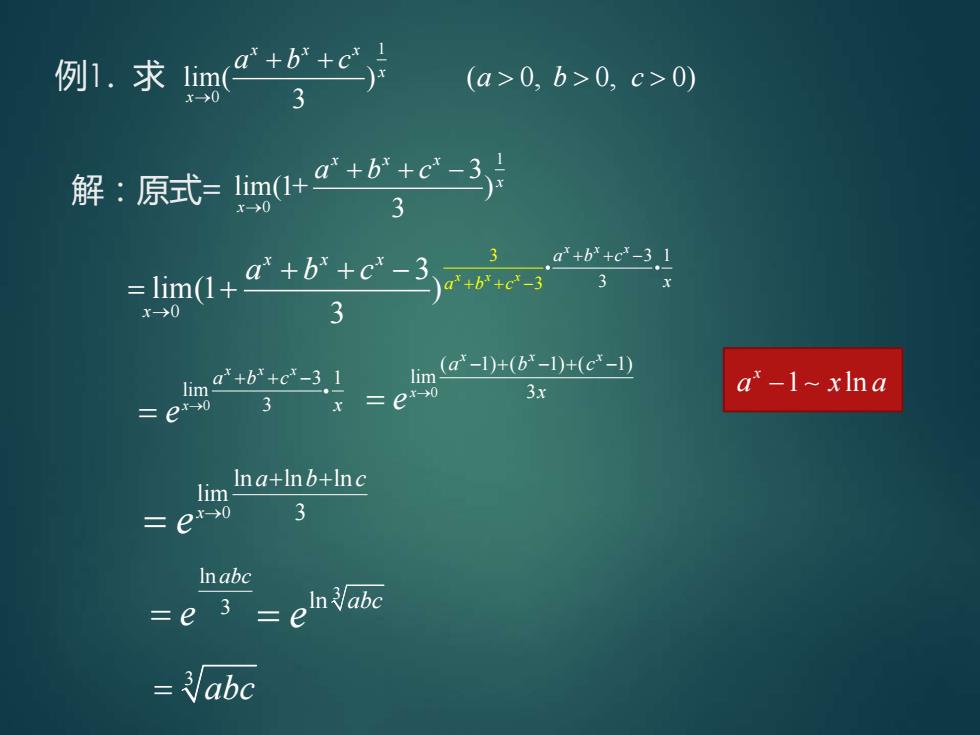
a'+b"+ct!例1.求lim(a>0, b>0, c>0)3解:原式=lim(1+g+b*+c*-3)3a"+b*+c*-313=lim(l+a'+b*+c*-3a+b+c-33Y3x-0m(d-)+(6*-1)+(c-1)a+b'+c-31α-1~xlnalim3xer-03二XX0e二Ina+Inb+Inclim-3=er->0InabcInabc3=e?=abc
例1. 求 1 0 lim( ) ( 0, 0, 0) 3 x x x x x abc a b c → + + 解:原式= 1 0 3 lim(1+ ) 3 x x x x x abc → + + − 3 1 3 0 3 3 3 lim(1 ) 3 x x x x x x a b x x x a b c x c x abc + + − + + − → + + − = +0 3 1 lim 3 x x x x a b c x e → + + − = 0 ( 1) ( 1) ( 1) lim 3 x x x x a b c x e → − + − + − = 0 ln ln ln lim x 3 a b c e → + + = ln 3 abc = e 3 = abc 1 ln x a x a − 3 ln abc = e

例2,当x→0时,etanx-esin*是xk的高阶无穷小,确定k的范围解:sinxetanxetanx-sinx _1Plimesinxlim中中仁X-0X-→0tanx-sinxtanx(l-cosx)etanx-sinx=limlim=lim二中X→0X-0x-→0YXx2=limlim=0中个12x-→0X->0k0又x是无穷小
例2 . 当 x → 0 时, 是 的高阶无穷小,确定 的范围. tan sin x x e e − k x k 解: tan sin 0 lim x x k x e e → x− tan sin sin 0 1 lim x x x k x e e x − → − = tan sin 0 1 lim x x k x e x − → − = 0 tan sin lim k x x x → x− = 0 tan (1 cos ) lim k x x x → x− = 2 0 1 2 lim k x x x → x = 3 0 1 lim 2 k x x → x = = 0 k 3 k 又 x 是无穷小 k 0 0 3 k
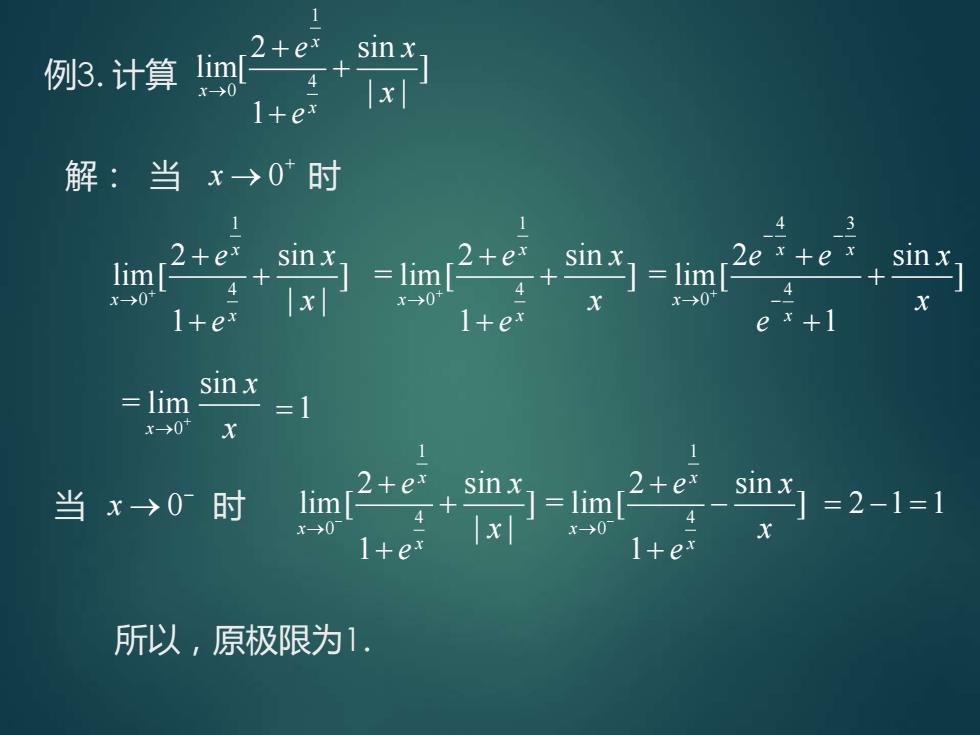
12+ersinxlim例3.计算X4X-→0x1+ex解:当X→0时11C+er2+er2e-sinxsinxsinxtelim[=lim[=lim|十444X-0X->0X-→0+x中x1+ex1+exX(+)esinx=lim三x→0+x2+ex2+ersinxsinxlim=lim=2-1=1当x→0时44/xX->0X-0x1+er1+er所以,原极限为1
例3. 计算 1 4 0 2 sin lim[ ] | | 1 x x x e x x e → + + + 解: 1 4 0 2 sin lim[ ] | | 1 x x x e x x e → + + + + 当 时 + x → 0 1 4 0 2 sin = lim[ ] 1 x x x e x x e → + + + + 4 3 4 0 2 sin = lim[ ] 1 x x x x e e x x e + − − → − + + + 0 sin = lim x x x → + =1 当 x 0 时 → − 1 4 0 2 sin lim[ ] | | 1 x x x e x x e → − + + + 1 4 0 2 sin = lim[ ] 1 x x x e x x e → − + − + = − = 2 1 1 所以,原极限为1

22n+1例3.求极限 lim[-(n+1)2)n→n+1n+2解:222n+12n+112n+1..+...+2n+1n+2(n+1)(n+1)2n2+1n+ln+1(n+1)2(n+1)222n+1(2n+1)(2n+2)1(2n+1)(2n+2)(n+1)2n+1n2+22(n+1)2(n+1)222n+12n+3n+1(2n+1)+·.+0022n+1liml=2由夹逼准则有(n+1)2n2+2n-n+1
例3. 求极限 2 2 2 1 2 2 1 lim[ ]. 1 2 ( 1) n n → n n n + + + + + + + 解: 2 2 2 1 2 2 1 + 1 2 ( 1) n n n n + + + + + + 2 2 2 1 2 2 1 + 1 1 1 n n n n + + + + + + 2 2 2 1 2 2 1 + ( 1) ( 1) ( 1) n n n n + + + + + +2 (2 1)(2 2) 2( 1) n n n + + + 2 (2 1)(2 2) 2( 1) n n n + + + 2 2 2 1 2 2 1 + 1 2 ( 1) n n n n + + + + + + (2 1) 1 n n + + 2 2 2 3 1 1 n n n + + + 2 2 2 1 2 2 1 + 1 2 ( 1) n n n n + + + + + + (2 1) lim 2 n 1 n → n + = + 2 2 2 3 1 lim 2 n 1 n n → n + + = + 2 2 2 1 2 2 1 lim[ ] 2 1 2 ( 1) n n → n n n + + + + = + + + 由夹逼准则有

例4.求数列V2,V2+V2,2+V2+V2,…的极限解:x=V2, x2=/2+xx,=2+x-数列有极限X>假设x,>-1则X+1=/2+x,>/2+×m-l单增X00limx,=lim(2+x-)=A?=2+A则n-n→o=A=-1(舍)A=2
例4. 求数列 2, 2+ 2 2+ 2+ 2 , , 的极限 解: 1 2 1 1 2, 2 , , 2 n n x x x x x = = + = + − 2 1 x x , 假设 1 , n n x x − 则 1 2 n n x x + = + 1 2 n x + − n = x 1 2 x 2 n 设 x 1 2 2 2 2 n n x x = + + = + 有界 单增 由 1 2 n n x x = + − 2 1 2 n n x x = + − lim n n x A → 设 = 则 2 1 lim lim(2 ) n n n n x x − → → = + 2 = + A A 2 = − = A A 1 ( ) 2 舍 , 数列有极限

1+x例5.f(x)=limlim +,求{()的间断点,并说明其类型。1+x解:当「xkl时,f(x)=lim=1+xn-01+x2n.2n-11+x中X=lim当「x>1时,f(x)= lim0n-→+01+x2n1n-→+o0+1松(1+x1x/1可能间断点x=1, x=-1f(x)=x=10X+100X=-1lim f(x)=0,x=-1是连续点(1) x=-1 时, lim f(x)=0X--limf(x)=0,x=1跳跃间断点limf(x)=2(2) x=1 时,x
例5. 2 ,求 的间断点,并说明其类型。 1 ( ) lim 1 n n x f x →+ x + = + f x( ) 解: 当 | | 1 x 时, 2 1 ( ) lim 1 1 n n x f x x →+ x + = = + + 当 | | 1 x 时, 2 2 1 2 2 1 1 1 ( ) lim lim 1 1 1 n n n n n n x x x f x x x − →+ →+ + + = = + + = 0 1 | | <1 0 | | 1 ( )= 1 1 0 1 x x x f x x x + = = − 可能间断点 x x = = − 1, 1 (1) 1 , x = − 时 1 lim ( ) 0 x f x → − − = +1 lim ( ) 0 x f x → − = , x = −1 是连续点 (2) 1 , x = 时 1 lim ( ) 2 x f x → − = +1 lim ( ) 0 x f x → = , x =1 跳跃间断点x −1 1 0 x +1 0

例6.设f(x)在[a,bl上连续a<x<x,<b.证明存在[a,b]使得5f()=2f(x)+3f(x)证::f(x)在[a,b]上连续m≤f(x)≤M.M,m分别为f(x)在[a,b]上的最大最小值2m+3m2f(x)+3f(x)2M+3M555即m≤2(x)+3/(2)≤M5由介值定理 3 e(a,b) 使得 (2)=27(x)+3/(±)得证.5
例 6. 设 f x( ) 在 [ , ] a b 上连续, a x x b 1 2 . 证明存在 [ , ] a b 使得 1 2 5 ( ) 2 ( ) 3 ( ). f f x f x = + f x( ) 在 [ , ] a b 上连续. m f x M ( ) . M m, 分别为 f x( ) 在 [ , ] a b 上的最大最小值 1 2 2 3 2 3 2 ( ) 3 ( ) 5 5 5 m m M M + + f x f x + 证: 1 2 2 ( ) 3 ( ) 5 f x f x m M + 即 由介值定理 ( , ) a b 使得 1 2 2 ( ) 3 ( ) ( ) 5 f x f x f + = 得证
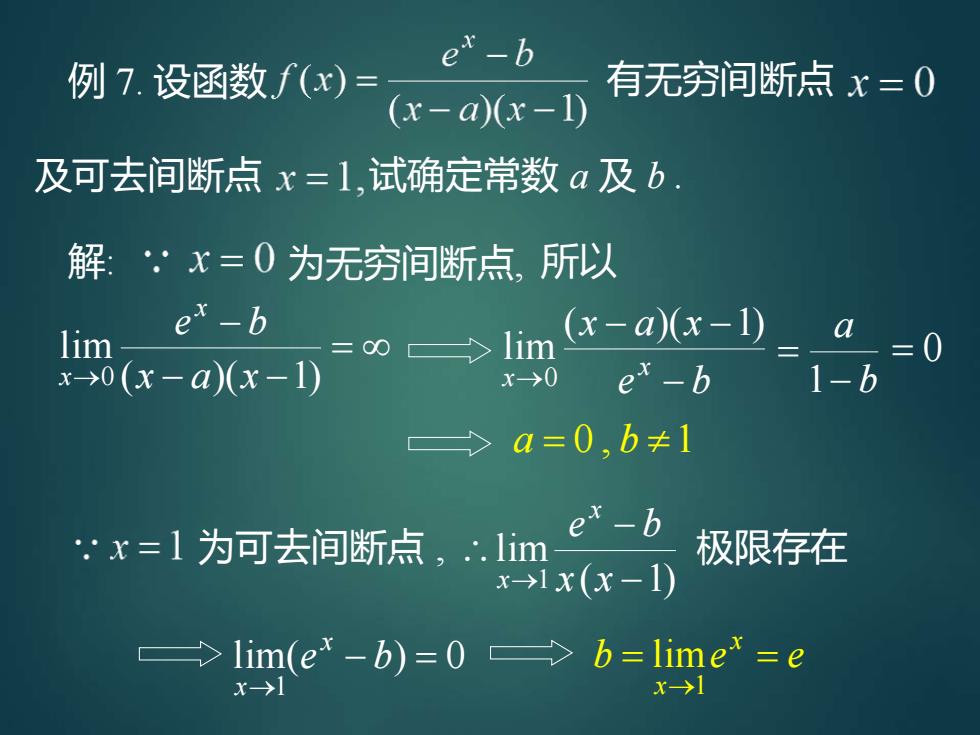
e"-b有无穷间断点x=0例7.设函数f(x)=(x-a)(x-1)及可去间断点x=1,试确定常数α及b解::x=0为无穷间断点,所以er-b(x-a)(x-1)alimlim=0=8x-0(x-a)(x-l)e-b1-bX-0α=0,b±1ex-b:x=1为可去间断点,.lim极限存在x-1x(x-1)>lim(ex-b)=0 b=lime*=ex-1x-→>1
有无穷间断点 及可去间断点 解: 为无穷间断点, = − − − → ( )( 1) lim 0 x a x e b x x 所以 e b x a x x x − − − → ( )( 1) lim 0 b a − = 1 = 0 a = 0 , b 1 为可去间断点 , ( 1) lim 1 − − → x x e b x x 极限存在 lim( ) 0 1 − = → e b x x b e e x x = = →1 lim 例 7. 设函数 试确定常数 a 及 b