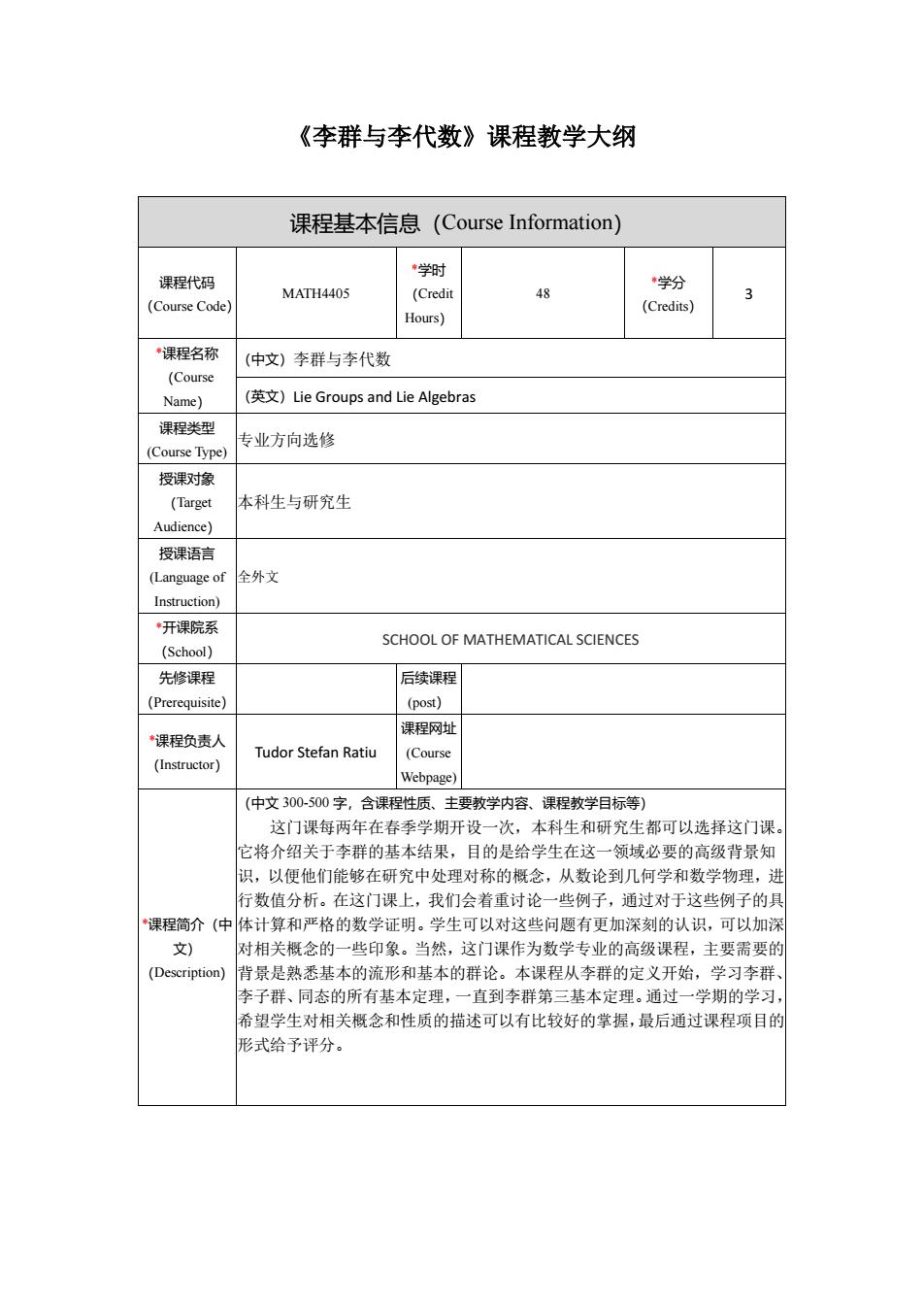
《李群与李代数》课程教学大纲 课程基本信息(Course Information) 课程代码 学时 (Course Code MATH4405 48 学分 (Credits) 课程名称 (中文)李群与李代数 (Course Name) (英文)Lie Groups and Lie Algebras 课程类型 专业方向选修 (Course Type 授课对家 (Target 本科生与研究生 授课语言 (Language of全外文 Instruction) +开课院系 (School) SCHOOL OF MATHEMATICAL SCIENCES 先修课程 后续课程 (Prerequisite) (nost) *课程负责人 课程网址 Tudor Stefan Ratiu (Course Instructor) Webpage) 中文300-500字,含课程性质、主要教学内容.课程教学目标等) 合学生在这 领域必要的高级背景知 识,以便他们能够在研究中处理对称的概念,从数论到几何学和数学物理,进 行数值分析。在这门课上,我们会者重讨论一些例子,通过对于这些例子的具 课程简介(中体计算和严格的数学证明。学生可以对这些间题有更加深刻的认识,可以加深 对相关得今的一此印象。当城,这门里作为数学专业的高级里得主要需要 (Description) 背景是熟悉基木的流形和基本的群论 本课程从李群的定义开始,学习李群 李子群、同态的所有基本定理,一直到李群第三基本定理。通过一学期的学习 希望学生对相关概念和性质的描述可以有比较好的掌握,最后通过课程项目的 形式给予评分
《李群与李代数》课程教学大纲 课程基本信息(Course Information) 课程代码 (Course Code) MATH4405 *学时 (Credit Hours) 48 *学分 (Credits) 3 *课程名称 (Course Name) (中文)李群与李代数 (英文)Lie Groups and Lie Algebras 课程类型 (Course Type) 专业方向选修 授课对象 (Target Audience) 本科生与研究生 授课语言 (Language of Instruction) 全外文 *开课院系 (School) SCHOOL OF MATHEMATICAL SCIENCES 先修课程 (Prerequisite) 后续课程 (post) *课程负责人 (Instructor) Tudor Stefan Ratiu 课程网址 (Course Webpage) *课程简介(中 文) (Description) (中文 300-500 字,含课程性质、主要教学内容、课程教学目标等) 这门课每两年在春季学期开设一次,本科生和研究生都可以选择这门课。 它将介绍关于李群的基本结果,目的是给学生在这一领域必要的高级背景知 识,以便他们能够在研究中处理对称的概念,从数论到几何学和数学物理,进 行数值分析。在这门课上,我们会着重讨论一些例子,通过对于这些例子的具 体计算和严格的数学证明。学生可以对这些问题有更加深刻的认识,可以加深 对相关概念的一些印象。当然,这门课作为数学专业的高级课程,主要需要的 背景是熟悉基本的流形和基本的群论。本课程从李群的定义开始,学习李群、 李子群、同态的所有基本定理,一直到李群第三基本定理。通过一学期的学习, 希望学生对相关概念和性质的描述可以有比较好的掌握,最后通过课程项目的 形式给予评分

(英文300-500字) This course is given every secnd year in the Spring Semester t will present the fundamental results on Lie groups,with the goal to give the students the necessary advanced background so that they can deal with the notion of symmetry in their research,all the way from number theory,through geometry and mathematical physics,to numerical analysis.Examples will be sized and corete,as well as full proofs,will be given.The only background needed isbasic famillarity with group *课程简介(英theory.The necessary background will be always reviewed.The course starts 文) with the definition of Lie groups,goes through all fundamental theorems on Lie (Description)groups,Lie subgroups,homomorphisms,all the way to Lie'sThird Fundamental rem. 课程目标与内容(Course objectives and contents) 1.回顾拓扑和微分几何的基本知识(B1) 课程目标 2介绍李群与李代数的基本概念和基本定理(B2,B4》 3.掌握紧李群的表示理论(82,B4) Course Object) 4.学会一些的分类标准(B2,B4,C3,) 章节教学内容(要点) 学时 教学形式 作业及考 课程思政融 对应课程 核要求 点 标 示例 教学内容讲度】 安排及对应海 每次23 程目标(Ca Schedule&第一拓扑群和微分几 道题目, 深厚的基 1 Requirements&音 今 3 授课 掌握基 础理论 Course 本概念 Objectives) 及计算 每次2-3 第 李群及其李代数 授课 道题日,深厚的基 章 掌握基础理论 本概念
*课程简介(英 文) (Description) (英文 300-500 字) This course is given every second year in the Spring Semester. It will present the fundamental results on Lie groups, with the goal to give the students the necessary advanced background so that they can deal with the notion of symmetry in their research, all the way from number theory, through geometry and mathematical physics, to numerical analysis. Examples will be emphasized and concrete computations, as well as full proofs, will be given. The only background needed is basic familiarity with manifolds and elementary group theory. The necessary background will be always reviewed. The course starts with the definition of Lie groups, goes through all fundamental theorems on Lie groups, Lie subgroups, homomorphisms, all the way to Lie’s Third Fundamental Theorem. 课程目标与内容(Course objectives and contents) *课程目标 (Course Object) 1.回顾拓扑和微分几何的基本知识(B1) 2.介绍李群与李代数的基本概念和基本定理(B2,B4) 3.掌握紧李群的表示理论(B2,B4) 4.学会一些的分类标准(B2,B4,C3,) *教学内容进度 安排及对应课 程目标 (Class Schedule & Requirements & Course Objectives) 章节 教学内容(要点) 学时 教学形式 作业及考 核要求 课程思政融入 点 对应课程目 标 示例: 第一 章 拓扑群和微分几 何 3 授课 每次 2-3 道题目, 掌握基 本概念 及计算 深厚的基 础理论 1 第 二 章 李群及其李代数 33 授课 每次 2-3 道题目, 掌握基 本概念 深厚的基 础理论 2
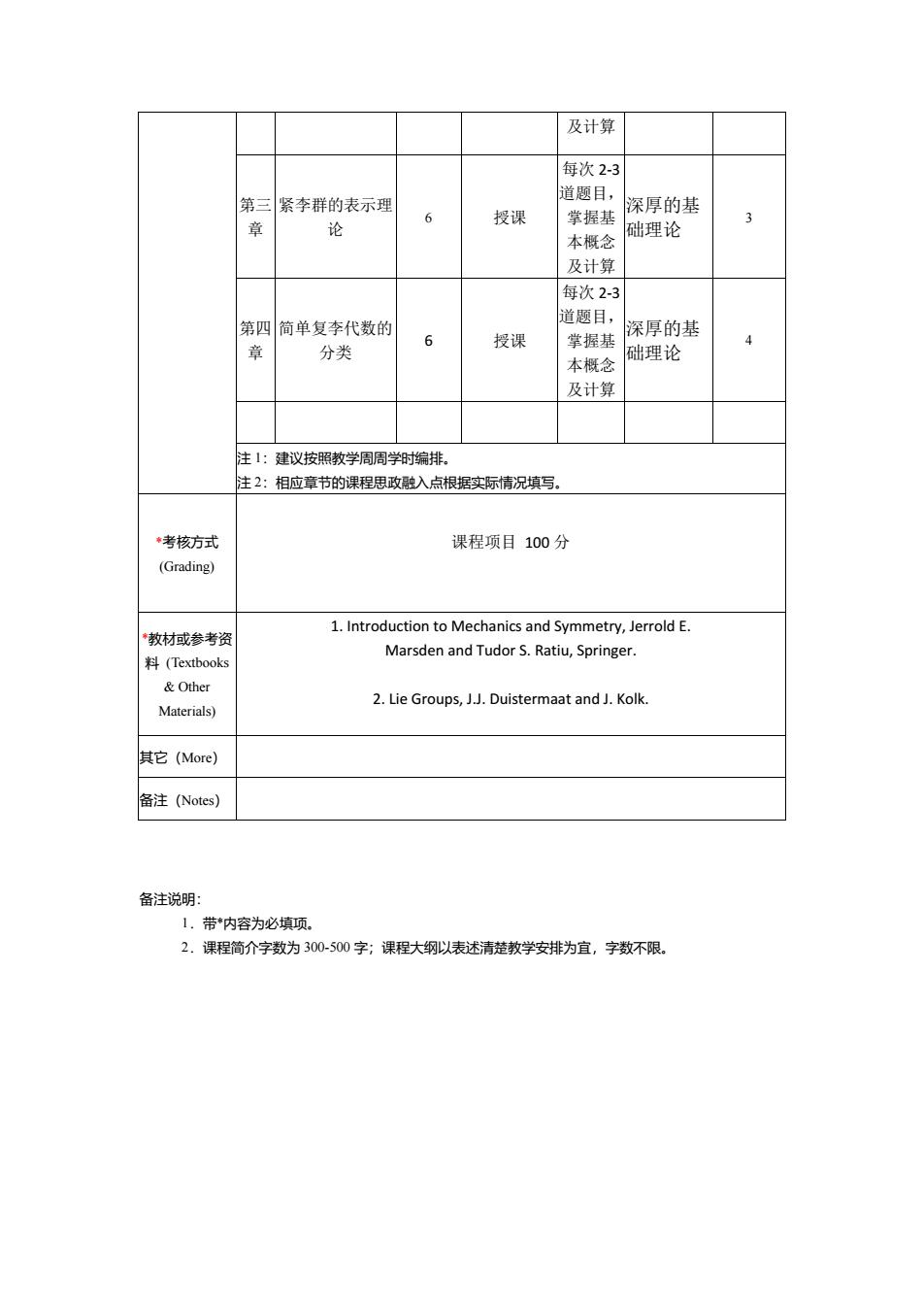
及计算 每次2-3 道题目 第三紧李群的表示理 6 授课 深厚的基 3 本概 础理论 及计算 每次2.3 道题目 掌握基 深厚的基 分类 6 授课 本概急 础理论 及计算 注1:建议按照教学周周学时综排, 注2:相应章节的课程思政融入点根据实际情况填与 考核方式 课程项目100分 (Grading) 教材或参考资 1.Introduction to Mechanics and Symmetry,Jerrold E. Mar sden and TudorS.Ratiu,Springer &Other 2.Lie Groups,J.J.Duistermaat and J.Kolk. Materials) 其它(Morc) 备注(Notes) 备注说明: 1.带内容为必填项。 2.课程简介字数为300-500字;课程大纲以表述清楚教学安排为宜,字数不限
及计算 第三 章 紧李群的表示理 论 6 授课 每次 2-3 道题目, 掌握基 本概念 及计算 深厚的基 础理论 3 第四 章 简单复李代数的 分类 6 授课 每次 2-3 道题目, 掌握基 本概念 及计算 深厚的基 础理论 4 注 1:建议按照教学周周学时编排。 注 2:相应章节的课程思政融入点根据实际情况填写。 *考核方式 (Grading) 课程项目 100 分 *教材或参考资 料 (Textbooks & Other Materials) 1. Introduction to Mechanics and Symmetry, Jerrold E. Marsden and Tudor S. Ratiu, Springer. 2. Lie Groups, J.J. Duistermaat and J. Kolk. 其它(More) 备注(Notes) 备注说明: 1.带*内容为必填项。 2.课程简介字数为 300-500 字;课程大纲以表述清楚教学安排为宜,字数不限